- #1
TerryW
Gold Member
- 191
- 13
- Homework Statement
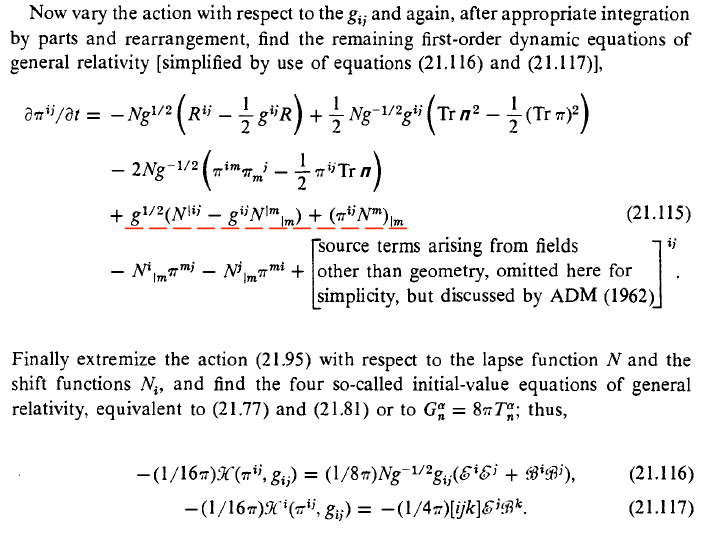
- I'm having another attempt to get a bit of help working out how MTW (21.115) (attached) can be derived from:
##I = \frac{1}{16π}\int[ \dot π^{ij} γ_{ij} - N\mathcal{H} -N_i\mathcal{H^i}]d^4x + \int\mathfrak{L}_{Field}d^4x \quad## MTW (21.95)
- Relevant Equations
- In the hope of making progress, I'm going to present my working so far bit by bit to see if anyone can spot where I am going wrong. I'll start with a bit that seems to work OK:
I am first going to vary ## - N\mathcal{H} ## wrt ##g_{ij}##
##\delta(- N\mathcal{H}) = \delta(-N[\gamma^{-\frac{1}{2}}(Trπ ^2 - \frac{1}{2}(Trπ )^2 -\gamma^{\frac{1}{2}}R])##
and I am going to concentrate on the first part and find ##\delta(-N[\gamma^{-\frac{1}{2}}(Trπ ^2 - \frac{1}{2}(Trπ )^2]) ## wrt ##g_{ij}##.
##\delta(-N[\gamma^{-\frac{1}{2}}(Trπ ^2 - \frac{1}{2}(Tr π )^2]) = \delta(-N^2[(-g)^{\frac{1}{2}}]^{-1}(Trπ ^2 - \frac{1}{2}(Tr π )^2) ##
## = -N^2(-1)[(-g)^{\frac{1}{2}}]^{-2} \frac{1}{2}(-g)^{\frac{1}{2}}g^{ij} (Trπ ^2 - \frac{1}{2}(Tr π )^2)\delta g_{ij} -N\gamma^{-\frac{1}{2}}\delta(Trπ ^2 - \frac{1}{2}(Tr π )^2)##
## = \frac{1}{2}N^2(-g)^{-\frac{1}{2}} g^{ij} (Trπ ^2 - \frac{1}{2}(Tr π )^2)\delta g_{ij} -N\gamma^{-\frac{1}{2}}\delta(Trπ ^2 - \frac{1}{2}(Tr π )^2)##
## = \frac{1}{2}N\gamma^{-\frac{1}{2}} g^{ij} (Trπ ^2 - \frac{1}{2}(Tr π )^2)\delta g_{ij} -N\gamma^{-\frac{1}{2}}(\delta(g_{js}π^{sm}g_{mi}π^{ij}) - \frac{1}{2} 2(Trπ)π^{ij}\delta g_{ij}##
## = \frac{1}{2}N\gamma^{-\frac{1}{2}} g^{ij} (Trπ ^2 - \frac{1}{2}(Tr π )^2)\delta g_{ij} -N\gamma^{-\frac{1}{2}}(π^{im}g_{ms}π^{sj} +g_{ms}π^{sj} π^{im}- (Trπ)π^{ij})\delta g_{ij}#### = [\frac{1}{2}N\gamma^{-\frac{1}{2}} g^{ij} (Trπ ^2 - \frac{1}{2}(Tr π )^2) -2N\gamma^{-\frac{1}{2}}(π^{im}π_m{}^j +g_{ms}π^{sj} π^{im}- \frac{1}{2}(Trπ)π^{ij})]\delta g_{ij}##
This has produced two of the terms in MTW's (21.115) which makes me feel that my process for finding the variation wrt ##g_{ij}## is probably sound.
Can anyone suggest where I might be missing something?RegardsTerry W
and I am going to concentrate on the first part and find ##\delta(-N[\gamma^{-\frac{1}{2}}(Trπ ^2 - \frac{1}{2}(Trπ )^2]) ## wrt ##g_{ij}##.
##\delta(-N[\gamma^{-\frac{1}{2}}(Trπ ^2 - \frac{1}{2}(Tr π )^2]) = \delta(-N^2[(-g)^{\frac{1}{2}}]^{-1}(Trπ ^2 - \frac{1}{2}(Tr π )^2) ##
## = -N^2(-1)[(-g)^{\frac{1}{2}}]^{-2} \frac{1}{2}(-g)^{\frac{1}{2}}g^{ij} (Trπ ^2 - \frac{1}{2}(Tr π )^2)\delta g_{ij} -N\gamma^{-\frac{1}{2}}\delta(Trπ ^2 - \frac{1}{2}(Tr π )^2)##
## = \frac{1}{2}N^2(-g)^{-\frac{1}{2}} g^{ij} (Trπ ^2 - \frac{1}{2}(Tr π )^2)\delta g_{ij} -N\gamma^{-\frac{1}{2}}\delta(Trπ ^2 - \frac{1}{2}(Tr π )^2)##
## = \frac{1}{2}N\gamma^{-\frac{1}{2}} g^{ij} (Trπ ^2 - \frac{1}{2}(Tr π )^2)\delta g_{ij} -N\gamma^{-\frac{1}{2}}(\delta(g_{js}π^{sm}g_{mi}π^{ij}) - \frac{1}{2} 2(Trπ)π^{ij}\delta g_{ij}##
## = \frac{1}{2}N\gamma^{-\frac{1}{2}} g^{ij} (Trπ ^2 - \frac{1}{2}(Tr π )^2)\delta g_{ij} -N\gamma^{-\frac{1}{2}}(π^{im}g_{ms}π^{sj} +g_{ms}π^{sj} π^{im}- (Trπ)π^{ij})\delta g_{ij}#### = [\frac{1}{2}N\gamma^{-\frac{1}{2}} g^{ij} (Trπ ^2 - \frac{1}{2}(Tr π )^2) -2N\gamma^{-\frac{1}{2}}(π^{im}π_m{}^j +g_{ms}π^{sj} π^{im}- \frac{1}{2}(Trπ)π^{ij})]\delta g_{ij}##
This has produced two of the terms in MTW's (21.115) which makes me feel that my process for finding the variation wrt ##g_{ij}## is probably sound.
Can anyone suggest where I might be missing something?RegardsTerry W