Intermediate axis theorem (Tennis racket theorem)

[Lambda96]

Homework Statement

see screenshot

Relevant Equations

Euler equation

Hi,

unfortunately, I am not getting anywhere with task b

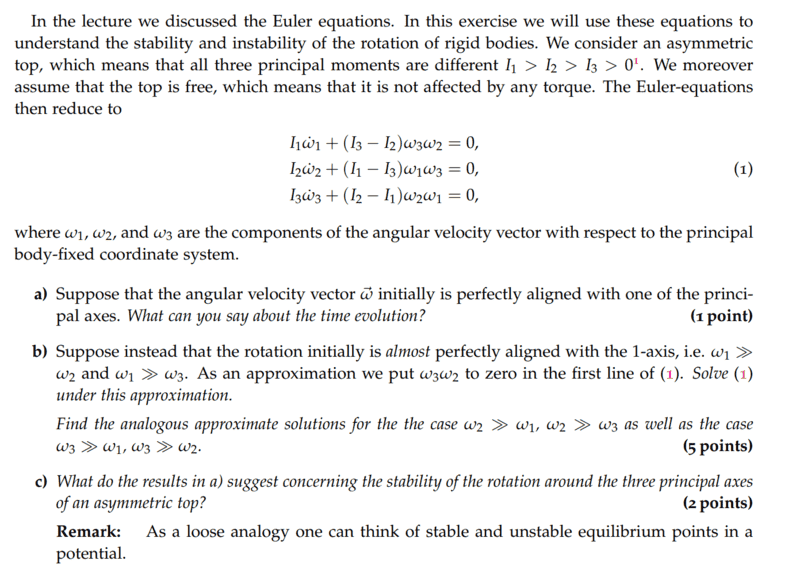
In the lecture we had the special case that $\vec{M}=0$ , $I_x=I_y=I , I \neq I_z$ and $\omega_z=const.$

Then the Euler equation looks like this.

$$I_x\dot{\omega_x}+\omega_y \omega_z(I_z-i_y)=0$$
$$I_y\dot{\omega_y}+\omega_z \omega_x(I_x-i_z)=0$$
$$I_z\dot{\omega_z}=0$$

With this, we then set up the following equations, where $\Omega=\frac{I_z-I}{I}$.

$$\dot{\omega_x}+\Omega \omega_y=0$$
$$\dot{\omega_y}-\Omega \omega_x=0$$

The solution for $\dot{\omega_x}$ and $\dot{\omega_y}$ are then as follows

$$\dot{\omega_x}(t)=Acos{\Omega t + \alpha}$$
$$\dot{\omega_y}(t)=Asin{\Omega t + \alpha}$$

I then wanted to use the approximation $\omega_1 \gg \omega_2$ , $\omega_1 \gg \omega_3$ and $\omega_3 \omega_2=0$ to solve the Euler equation also according to the same recipe

The Euler equation looks like this:

$$I_1\dot{\omega_1}=0$$
$$I_2\dot{\omega_2}+\omega_1 \omega_3(I_1-I_3)=0$$
$$I_3\dot{\omega_3}+\omega_2 \omega_1(I_2-I_1)=0$$

After that I got the following equation

$$\dot{\omega}_2+\frac{(I_1-I_3)\omega_1}{I_2}\omega_3=0$$
$$\dot{\omega}_3-\frac{(I_2-I_1)\omega_1}{I_3}\omega_2=0$$

With $\frac{(I_1-I_3)\omega_1}{I_2}=\Omega_1$ and $\frac{(I_2-I_1)\omega_1}{I_3}=\Omega_2$, the above equation is

$$\dot{\omega}_2+\Omega_1 \omega_3=0$$
$$\dot{\omega}_3- \Omega_2 \omega_2=0$$

Unfortunately, I'm not getting anywhere now, because I don't know what $\omega_1$ and $\omega_2$ have to look like to satisfy the two equations, because unfortunately I don't have $\Omega$, but two different ones with $\Omega_1$ and $\Omega_2$.

 

[TSny]

After that I got the following equation$$\dot{\omega}_2+\frac{(I_1-I_3)\omega_1}{I_2}\omega_3=0$$$$\dot{\omega}_3-\frac{(I_2-I_1)\omega_1}{I_3}\omega_2=0$$

Shouldn't the second equation have a plus sign for the second term on the left side?

With $\frac{(I_1-I_3)\omega_1}{I_2}=\Omega_1$ and $\frac{(I_2-I_1)\omega_1}{I_3}=\Omega_2$,

With these definitions, note that $\Omega_2$ is a negative number. It might be less confusing if you define $\Omega_2 = \frac{(I_1-I_2)\omega_1}{I_3}$ so that both $\Omega_1$ and $\Omega_2$ are positive.

$$\dot{\omega}_2+\Omega_1 \omega_3=0$$
$$\dot{\omega}_3- \Omega_2 \omega_2=0$$

Unfortunately, I'm not getting anywhere now, because I don't know what $\omega_1$ and $\omega_2$ have to look like to satisfy the two equations, because unfortunately I don't have $\Omega$, but two different ones with $\Omega_1$ and $\Omega_2$.

Take the time derivative of the first equation and then use the second equation to substitute for $\dot \omega_3$.

 

[Lambda96]

Thank you TSny for your help

Regarding the minus sign, I had made a mistake, I actually meant that $\Omega_2$ is negative, since $I_1>I_2$ and thus becomes negative. But I'll leave this interpretation out of the derivation for now.

The equation $\dot{\omega_2}+\Omega_1 \omega_3=0$ derived in time is as follows, actually I would have to use the product rule, but since $\dot{\omega_1}=0$ I can leave this term out directly and get

$$\ddot{\omega_2}+\Omega_1 \dot{\omega_3}=0$$

The second equation then solved for $\dot{\omega_3}$ is $\dot{\omega_3}=-\Omega_2 \omega_2$.

Then substituting back into the first equation gives

$$\ddot{\omega_2}-\Omega_1 \Omega_2 \omega_2=0$$

Solving this differential equation then gives:

$$\omega_2=c_1e^{\sqrt{\Omega_1 \Omega_2} t}+c_2e^{-\sqrt{\Omega_1 \Omega_2} t}$$

 

[TSny]

The equation $\dot{\omega_2}+\Omega_1 \omega_3=0$ derived in time is as follows, actually I would have to use the product rule, but since $\dot{\omega_1}=0$ I can leave this term out directly and get

$$\ddot{\omega_2}+\Omega_1 \dot{\omega_3}=0$$

ok

The second equation then solved for $\dot{\omega_3}$ is $\dot{\omega_3}=-\Omega_2 \omega_2$.

ok. It appears to me that you are still defining $\Omega_2$ as $\frac{(I_2-I_1)\omega_1}{I_3}$. So, $\Omega_2$ is a negative number. That's alright, but then you need to keep in mind that $\sqrt{\Omega_1 \Omega_2}$ is imaginary.

Then substituting back into the first equation gives

$$\ddot{\omega_2}-\Omega_1 \Omega_2 \omega_2=0$$

Solving this differential equation then gives:

$$\omega_2=c_1e^{\sqrt{\Omega_1 \Omega_2} t}+c_2e^{-\sqrt{\Omega_1 \Omega_2} t}$$

ok. The exponents on $e$ are imaginary. I think it would be nicer to rewrite this without imaginary quantities. You could use Euler's formula. Or, you could go back and define $\Omega_2$ such that it is positive and rederive the differential equation for $\ddot \omega_2$. Then, you don't run into imaginary quantities.