Method of Characteristics/Fan of characteristics

[Leb]

Hi all,

I expect that this might be rarely taught (as I have not found much on the web).

But maybe some of you have encountered this. I attach the problem

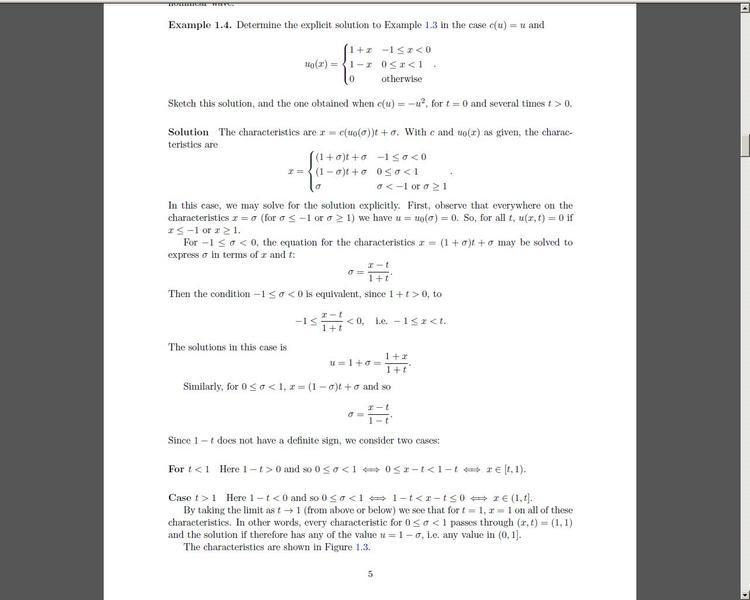
At the end the author says to take the limit as t goes to 1. But to me, it does not make sense to look at something that is ε<x<=ε (I mean this is false) and from that to deduce that all the characteristics pass through (x,t)=(1,1)

 

[Vargo]

The method of characteristics is not so rare. Usually the simplest thing to do once you have found all characteristics is to draw a good picture in order to eliminate the extra parameter. This appears to be about half the original problem, but I surmise that u is constant along the characteristics in this example.

So you have three types of characteristics. Outside the band, |x|<=1, the characteristics are parallel to the x-axis and therefore, u = 0 on those. So u(x,t)=0 if x < -1 or x > 1.

The other characteristics are straight lines whose slope depends on where they they intersect the x axis. If they intersect it at a point 0<= x < 1, then they join the x-intercept to (t,x)=(1,1). If they intersect it at a point -1<=x<0, then they join the x intercept to (t,x)=(-1,1). If you draw that system of lines (which is apparently done in figure 1.3, then all that algebra should make sense.

You can see that if you are inside the band, then there are two regions which are separated by the line x=t. So assuming -1<x<1, if x>t, then the point is on a line connected with (1,1), so you can find the slope 1-s= 1-x / 1-t. On that characteristic u = 1 - s = (1-x)/(1-t)

If x < t, then its characteristic passes through (-1,-1), so its slope is 1+s = (x+1)/(t+1). On that characteristic, u = 1+s = (1+x)/(1+t).

Ultimately, the value of u is just the slope of the characteristic passing through that point, so you can see from the drawing where the discontinuities occur. In particular, along x = 1, for t>=1, and x=-1, for t<=-1.

 

[Leb]

Thank you for your reply, but I don't get a few things you explained.

If they intersect it at a point 0<= x < 1, then they join the x-intercept to (t,x)=(1,1).

How do you know that ? How do you know it will intersect at (t,x)=(1,1) ?

So assuming -1<x<1, if x>t, then the point is on a line connected with (1,1), so you can find the slope 1-s= 1-x / 1-t. On that characteristic u = 1 - s = (1-x)/(1-t)

Lost you here again. How do you define s ?

I am mostly bothered by the fact that our sigma is defined by having a denominator 1-t and we let t=1

I THINK I sort of get how the lines are drawn (you just follow the wave profile in different times, and for every point, you connect it with the same one, just at a different time). And in the example above the, when the wave profile becomes a right triangle u=1 at x=1 (intuitively) but I have no time intuition in this case...

 

[Vargo]

s = Lazy man's sigma.

I don't know why the author explains it the way he does. It's like he doesn't want to refer to a picture to explain the reasoning. So instead you have to follow the algebra which is more complicated. Perhaps I am missing something.

First of all, s=sigma is not defined by that formula with 1-t in the denominator. It is defined to be the initial x value along the characteristic (i.e. the x-intercept). That is, it is a parameter for the Cauchy surface.

Take 0<=s < 1. Then the corresponding characteristic is
x= (1-s)t + s.
That is a straight line with x intercept = s and positive slope. At t=1, x=1 (by substitution).

Similarly, if -1<s<0, the characteristic is
x = (1+s)t+s.
Its x intercept is s and also has positive slope. Again by substituting t=-1, you find that it passes through the point (-1,-1).

If you draw all these possible lines, then you can see that there are three types. The horizontal ones outside the band. The ones to the left of x=t (inside the band) which have positive x intercepts and which pass through (1,1). And the ones to the right of x=t (inside the band) which have negative x intercepts and which pass through (-1,-1). So given any point (t,x) inside that band, you can recover s by first checking which region of the band you are in (x>t or x<t), and then finding the slope to the corresponding point (1,1) or (-1,-1).

Now there is a question of how to define u(t,x) at points (t,x) which lie on more than one of these lines. For example the point (3,2) lies on three different lines in this system of lines. The simplest thing is probably to just think of u(t,x) as a multiple valued function. u(3,2) would have three different values. u(-1,0) would just have one value because there is only one characteristic passing through that point.

Or you can choose to define u(t,x) at these points by some scheme of your choosing. For example, think of u(t,x) as a wave radiating from the Cauchy surface t=0. Then let u(t,x) be the "closest" value from the Cauchy surface. For example, the point (3,2) is on the characteristic x=2 (which starts at (0,2)). And it lies on the characteristic that started at (0,1/2), and it lies on the characteristic that started from (0,-1/4). That gives three possible values: 0, 1/2, 3/4. The "closest" value would be 0, which came from the point nearest of the three points, (0,2). By that scheme the value of u(3,2)=0. Similarly u(1,1)=0.

 

[Leb]

Take 0<=s < 1. Then the corresponding characteristic is
x= (1-s)t + s.
That is a straight line with x intercept = s and positive slope. At t=1, x=1 (by substitution).

Similarly, if -1<s<0, the characteristic is
x = (1+s)t+s.
Its x intercept is s and also has positive slope. Again by substituting t=-1, you find that it passes through the point (-1,-1).

Oh, so all you do is look at the characteristic equation and check what values of x and t you need get rid of s ? Which will then work for any s in the region you described !

Thank you ver much, Vargo, you are awesome !