Moment of Inertia of an inclined disk

[Raghavendar Balaji]

Homework Statement

1. Homework Statement
An uniform disk of mass m, thickness L and radius r is suspended from the ceiling by a wire. It is attached to the disk at a distance, d from the center of mass, so that it inclines at an angle A to the vertical. What is the moment of inertia of the inclined disk through that axis/string?

Homework Equations

I know that the MOI of a horizontal disc about the vertical perpendicular axis to the base is MR^2 /2.

The Attempt at a Solution

I did the problem initially without the parallel axis theorem, hinging the body to the center. I took an element dr at a distance of r × sin(A) to the vertical. Then dV = 2 × pi × r × dr × L and ρ = M/(pi × (R^2) × L). Then ∫(distance)^2 × ρ × dV. I got the answer which is ((M × R^2) × sin^2 (A)) /2, which is wrong, so I can't proceed to the next step.

Kindly help me regarding this.

 

[Orodruin]

I know that the MOI of a horizontal disc about the vertical perpendicular axis to the base is MR^2 /2.

This is not sufficient. You need the full moment of inertia tensor, i.e., you also need to know what the MOI about the axis parallel to the base is (or compute it). This will give you the full moment of inertia tensor.

I did the problem initially without the parallel axis theorem, hinging the body to the center.

Since the centre of mass needs to be on the axis of rotation for the disk to be in equilibrium, you will not need the parallel axis theorem. What you do need is the relation between the general moment of inertia tensor and the moment of inertia relative to a particular axis of rotation (unless you want to compute the moment of inertia from first principles using its definition).

I took an element dr at a distance of r × sin(A) to the vertical. Then dV = 2 × pi × r × dr × L and ρ = M/(pi × (R^2) × L).

This argumentation is correct only for points that are directly in the direction of the attachment. Using polar coordinates on the disk, as you have done, the distance to the axis will depend on both $r$ and the polar coordinate $\varphi$.

 

[Raghavendar Balaji]

This is not sufficient. You need the full moment of inertia tensor, i.e., you also need to know what the MOI about the axis parallel to the base is (or compute it). This will give you the full moment of inertia tensor.Since the centre of mass needs to be on the axis of rotation for the disk to be in equilibrium, you will not need the parallel axis theorem. What you do need is the relation between the general moment of inertia tensor and the moment of inertia relative to a particular axis of rotation (unless you want to compute the moment of inertia from first principles using its definition).This argumentation is correct only for points that are directly in the direction of the attachment. Using polar coordinates on the disk, as you have done, the distance to the axis will depend on both $r$ and the polar coordinate $\varphi$.

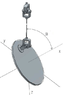
Thanks for the suggestion! :D Sorry, I forgot to add that . Considering that there are no product of inertia terms, the moment of inertia through the diameter i.e., Iyy and Izz are same, which is (MxR^2) / 4. So we know the matrix, now. And, initially when the vertical axis passes through the center, we won't need parallel axis theorem but if it is to be translated to a distance as shown in the diagram, I guess we need it. Am I fortunately right in this case?

Can I know if I should consider the angle for the dV element too or something else? I saw somewhere that the MOI for an inclined disk is MR^2/4 * (1 + sin^2(A)) but not sure if it's right. I tried deriving this but sadly didn't succeed.

 

[Orodruin]

And, initially when the vertical axis passes through the center, we won't need parallel axis theorem but if it is to be translated to a distance as shown in the diagram, I guess we need it

It is not translated a distance in the diagram. The attachment point is not on the axis of rotation.

Considering that there are no product of inertia terms, the moment of inertia through the diameter i.e., Iyy and Izz are same, which is (MxR^2) / 4. So we know the matrix, now.

So given that. What would be the moment of inertia relative to the direction offset by an angle $\alpha$ relative to the x-axis? (The unit vector in that direction is $\vec e_x \cos(\alpha) + \vec e_y \sin(\alpha)$.)

Can I know if I should consider the angle for the dV element too or something else? I saw somewhere that the MOI for an inclined disk is MR^2/4 * (1 + sin^2(A)) but not sure if it's right. I tried deriving this but sadly didn't succeed.

I suggest that you use polar coordinates on the disk and use geometry to figure out the perpendicular distance between the axis of rotation and any given point on the disk as a function of those coordinates. The resulting integral should be simple.

 

[Raghavendar Balaji]

It is not translated a distance in the diagram. The attachment point is not on the axis of rotation.So given that. What would be the moment of inertia relative to the direction offset by an angle $\alpha$ relative to the x-axis? (The unit vector in that direction is $\vec e_x \cos(\alpha) + \vec e_y \sin(\alpha)$.)I suggest that you use polar coordinates on the disk and use geometry to figure out the perpendicular distance between the axis of rotation and any given point on the disk as a function of those coordinates. The resulting integral should be simple.

I thought that this would be the step by step process. So can you tell me if it's possible?

Since the angle of inclination isn't changing from 0 to R or Rcos(A), I didn't know if I had to consider it in dV. If it has to be considered, should it be 0 to pi/2?

Sorry I couldn't comprehend totally what you had mentioned in the comment, but I solved it this way. It's wrong of course, but is the approach still right?
Thanks in advance! :)

P.S - Forgive me for the shabby writing!

 

[Orodruin]

You are still not taking the $\phi$ dependence into account. I suggest you write the y and z positions as functions of the polar coordinates and then think about which of these get a closer distance to the axis upon rotation.

 

[Raghavendar Balaji]

You are still not taking the $\phi$ dependence into account. I suggest you write the y and z positions as functions of the polar coordinates and then think about which of these get a closer distance to the axis upon rotation.

Hi, so from what I understand, if the angle between the base of the disc and the vertical is taken as Φ, which is 90-α in the diagram, then y co-ordinate is bound to change and z remains constant. The y co-ordinates would be y.cos(Φ) and y.sin(Φ). So for the moment of inertia calculation, considering the perpendicular distance from the axis of rotation(about the vertical string), we should take y.sin(Φ), but what should I take as the limits of the inner integral? 0 to R?

For the Φ consideration, should it be the outer integral from 0 to Φ and the total terms inside the integrals as (y.sin(Φ))^2.2πy.dy.L.dΦ? I'm confused. :/

 

[Orodruin]

One step at a time. If I use polar coordinates on the disk with radius $r$ and polar coordinate $\varphi$, what are the $y$ and $z$ coordinates of a point with given polar coordinates?

Once you have answered that, what is the corresponding distance to the tilted axis?

 

[Raghavendar Balaji]

One step at a time. If I use polar coordinates on the disk with radius $r$ and polar coordinate $\varphi$, what are the $y$ and $z$ coordinates of a point with given polar coordinates?

Once you have answered that, what is the corresponding distance to the tilted axis?

:D sure will do! The polar co-ordinates with angle to the vertical would be y = r.cosΦ and z = r.sinΦ and if the angle is w.r.t horizontal, it would be the reverse. Is it right?

 

[Orodruin]

Do not confuse the angle between the disk and the axis with the polar angle used as a coordinate on the disk. One of those angles (the angle between the disk and the axis) is constant whereas the other (the polar coordinate angle on the disk) depends on where on the disk you are.

 

[Raghavendar Balaji]

Yes I agree, a point on the circumference of the disk would have the mentioned coordinates which changes with the location of the point, but the hinged angle would remain constant. Right?

 

[Orodruin]

Yes I agree, a point on the circumference of the disk would have the mentioned coordinates which changes with the location of the point, but the hinged angle would remain constant. Right?

Yes, so you should use different notation for those two angles. Call the inclination angle $\beta$ and the polar angle $\varphi$. So your y and z coordinates are $y = r\cos\varphi$ and $z = r\sin\varphi$. Given $y$ and $z$ coordinates (and an x-coordinate $x = 0$), what is the distance to the inclined axis? (You can just write this in terms of y and z and later insert the expressions in terms of $r$ and $\varphi$).

 

[Raghavendar Balaji]

When the inclination is β, then for both the co-ordinates, would it be multiplied by sinβ? Sorry if I am wrong :(

 

[Orodruin]

When the inclination is β, then for both the co-ordinates, would it be multiplied by sinβ? Sorry if I am wrong :(

No, this is not the case. I suggest you try it out by taking a piece of paper and a pen. Draw two orthogonal lines on the paper, representing y and z coordinate lines. Then you can use a pen as the normal vector (away from the lines) and rotate around the z axis. Which of the lines come closer to the pen when you do the rotation?

 

[Raghavendar Balaji]

No, this is not the case. I suggest you try it out by taking a piece of paper and a pen. Draw two orthogonal lines on the paper, representing y and z coordinate lines. Then you can use a pen as the normal vector (away from the lines) and rotate around the z axis. Which of the lines come closer to the pen when you do the rotation?

When the vector is kept normal to the lines i.e., perpendicular to the surface of the paper, the y-axis comes closer to the pen when rotated about the z axis. Is it right?

 

[Orodruin]

When the vector is kept normal to the lines i.e., perpendicular to the surface of the paper, the y-axis comes closer to the pen when rotated about the z axis. Is it right?

Well, not the y-axis (it already has zero distance to the axis), but the general y-coordinate line comes closer, yes. What about the z-coordinate line?

 

[Raghavendar Balaji]

Well, not the y-axis (it already has zero distance to the axis), but the general y-coordinate line comes closer, yes. What about the z-coordinate line?

Oh yes, I'm sorry, the y coordinate line comes closer and not the axis whereas the z coordinate line remains constant throughout the rotation. :)

 

[Orodruin]

Oh yes, I'm sorry, the y coordinate line comes closer and not the axis whereas the z coordinate line remains constant throughout the rotation. :)

So what does this mean for the squared distance to the axis of the point given by coordinates x = 0 and arbitrary y and z?

 

[Raghavendar Balaji]

So what does this mean for the squared distance to the axis of the point given by coordinates x = 0 and arbitrary y and z?

I guess only the square of y coordinate line, since z remains constant and x = 0. Am I right?

 

[Orodruin]

No, that the z coordinate does not change does not mean that you can ignore it when you compute the distance to the axis.

 

[Raghavendar Balaji]

No, that the z coordinate does not change does not mean that you can ignore it when you compute the distance to the axis.

Ohh yes! So it would be the sum of squared distance of y and z co-ordinate. Am I right?

 

[Orodruin]

Ohh yes! So it would be the sum of squared distance of y and z co-ordinate. Am I right?

It is difficult to say when you just put it in words. Can you write it down in terms of equations?

 

[Raghavendar Balaji]

Oh sorry! The distance would be √((ysinβ)^2 + z^2). Is that it ?

 

[Orodruin]

Oh sorry! The distance would be √((ysinβ)^2 + z^2). Is that it ?

Yes, but with a cosine instead of a sine. When there is no inclination ($\beta = 0$) you should recover the Pythagorean theorem. (Essentially you can replace your $\beta$ with $\alpha$.)

Now, what happens when you insert the expressions for y and z in terms of the polar coordinates $r$ and $\varphi$? What does this tell you about the moment of inertia that is given by
$$
\int_D \rho \ell^2 dA,
$$
where $\rho$ is the area density, $\ell$ the distance from the axis discussed above, and $dA = r\, dr \, d\varphi$ is the area element of the disk?

 

[Raghavendar Balaji]

Yes, then it would be ρ∫((r.cos(Φ).cos(α))^2 + (r.sin(Φ))^2).r.dr.dΦ, with Φ ranging from 0 to π/2 and r from 0 to R?

 

[Raghavendar Balaji]

Hi! I did it from 0 to 2.pi and got the right value! Thank you very much for the invaluable help! I'll never forget this anytime, now! :) Just a small question, we found out the moment of inertia about an axis through the COG. So for the actual problem, since it is offset from the center, can I use the parallel axis theorem?

 

[Orodruin]

So for the actual problem, since it is offset from the center, can I use the parallel axis theorem?

The rotational axis in this problem is not offset from the centre of mass.

If it was, then yes you could use the parallel axis theorem.

 

[Raghavendar Balaji]

Oh okay! Is it possible to project the disk onto a horizontal plane which would result in an ellipse and then use the parallel axis theorem to it? Or if not, how can this be dealt with?