- #1
WWCY
- 479
- 12
- Homework Statement
- From Blundell's QFT
- Relevant Equations
- Fourier transforms
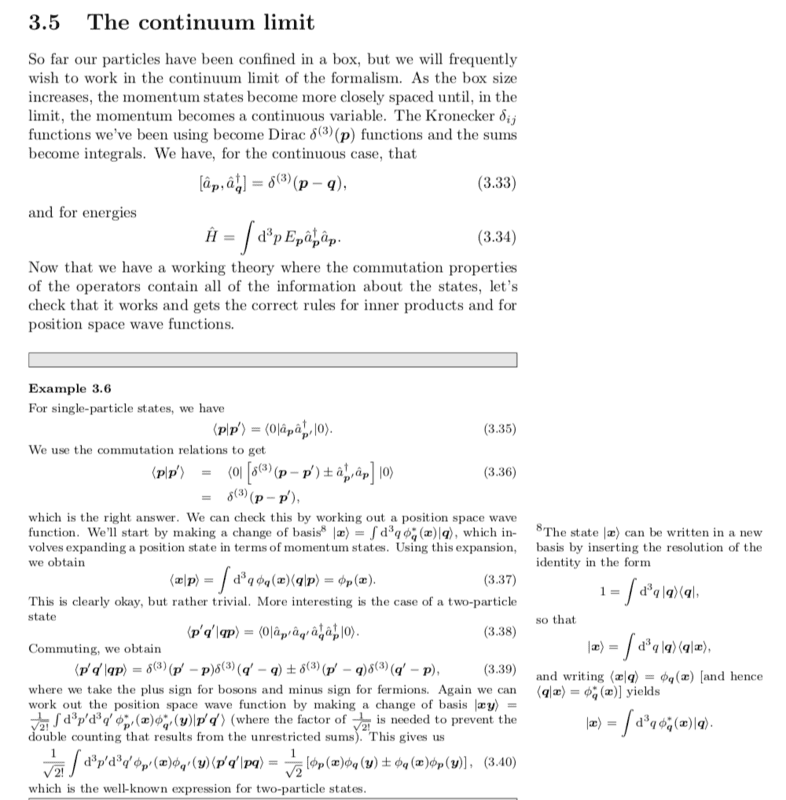
I have an issue trying to understand the derivation of equation 3.40 (screenshot attached) of Blundell's QFT book. Here's my attempt.
##| x,y \rangle = |x\rangle \otimes |y\rangle = \Big( \int dp' \phi_{p'}(x)|p'\rangle \Big) \otimes \Big( \int dq' \phi_{q'}(y)|q'\rangle \Big)## which gives ##\int dp' \int dq' \phi_{p'}(x) \phi_{q'}(y) |p',q'\rangle##.
I can't seem to understand the argument by Blundell that "the factor of ##1/\sqrt{2!}## is needed to prevent double counting that results from unrestricted sums". Why does the factor of ##1/\sqrt{2!}## not "pop out" on its own? Aren't Fourier transforms supposed to preserve normalisation?Cheers.
##| x,y \rangle = |x\rangle \otimes |y\rangle = \Big( \int dp' \phi_{p'}(x)|p'\rangle \Big) \otimes \Big( \int dq' \phi_{q'}(y)|q'\rangle \Big)## which gives ##\int dp' \int dq' \phi_{p'}(x) \phi_{q'}(y) |p',q'\rangle##.
I can't seem to understand the argument by Blundell that "the factor of ##1/\sqrt{2!}## is needed to prevent double counting that results from unrestricted sums". Why does the factor of ##1/\sqrt{2!}## not "pop out" on its own? Aren't Fourier transforms supposed to preserve normalisation?Cheers.