Optics/ total internal reflection (non-trivial at least for me)

[ed1234]

Homework Statement

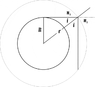
The refractive index of the Earth's atmosphere is:
n=1.01+(alpha)(R-r)
where (alpha) is a constant, r is radial distance from Earth's centre and R is the Earth's radius. By considering a path comprising a series of total internal reflections or otherwise, find a value of alpha for which a light ray emitted horizontally close to the Earth's surface would go around the Earth.

The Earth may be taken to be a perfect sphere radius R and the effects of absorption ignored).

Homework Equations

n1sin(theta1)=n2sin(theta2)

The Attempt at a Solution

I'm really fairly stuck on this on. I think we're looking for a gradient of refractive index such that the curvature of the light ray is equal to the curvature of the Earth but I'm not sure how to go about doing this quantitativly. Any hints/help would be appreciated.
Thanks

 

[andrevdh]

A very simplistic approach, but at least it gives you something.

I guess you take n1 at r = R.

 

[ed1234]

Thanks for your suggestion, but since there's no single boundary (n changes gradually) I'm not sure that your picture is quite what the question wants.

If anyones got any further help I'd appreciate it.
Thanks

 

[andrevdh]

With a gradual change in refractive index I doubt that a state of total internal reflection would occur, but I might be proven wrong. My take on the situation is that one needs a drastic change in refractive index to achieve total internal reflection. Unless the problem uses the term but actually means that the beam just keeps on gradually curling around the earth, which I find hard to believe, since you generally find such behaviour only in the vicinity of black holes!

A more realistic approach for the refractive indices in my drawing might be to take n1 at r and n2 = 1.0 (vacuum).

I am amazed that the astonomy people have'nt jumped onto this thread by now.

It seems you are trying to solve a quite advance problem http://www.journals.uchicago.edu/AJ/journal/issues/v119n5/200020/200020.html"