Power dissipated by a resistor on a coaxial cable

[gausswell]

Homework Statement

Find the power dissipated by the resistor.

Relevant Equations

P=IV, P=V^2/R

I need help with part c.

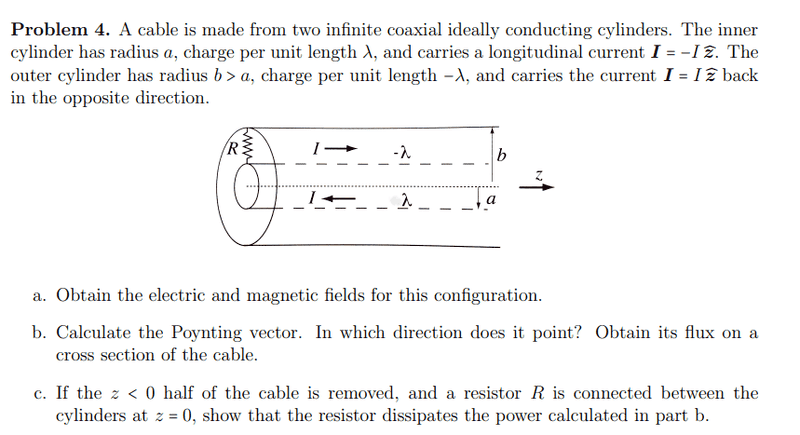
My solution:

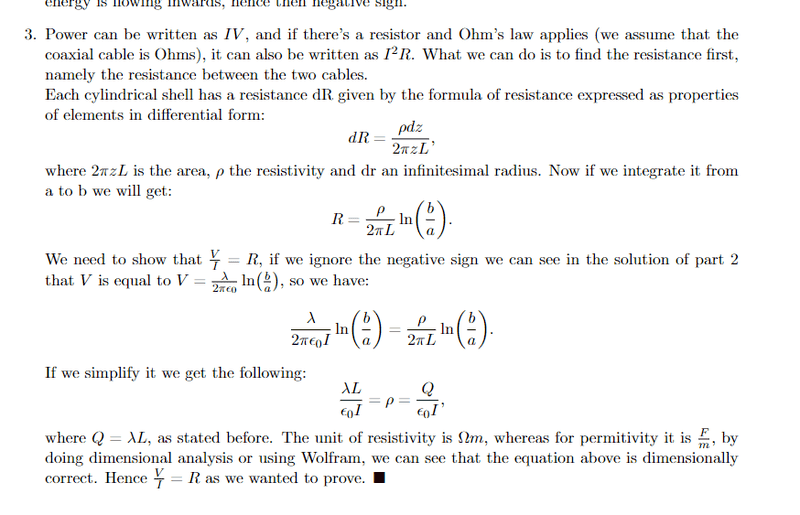
Is there an other way to do this other than dimensional analysis?
P.S "dr an infinitesimal radius", it ofcourse should be dz.

 

[TSny]

If possible, please type your work rather than post images of your work. It makes it easier for us to quote specific parts of your work.

I'm not following your calculation of R. In part (c), R represents the resistance of a resistor that is connected between the two cylinders. R can have an arbitrary value. The question asks you to show that for any value of R, the power dissipated in the resistor equals the rate at which electromagnetic field energy is propagating along the cable in the space between the two cylinders (as found using the Poynting vector from part (b)).

EDIT: For steady-state conditions with the resistor in place, we need to satisfy $V = IR$. $V$ is determined by $\lambda$ (and $a$ and $b$). So, if the values of $\lambda$ and $I$ are specified, then $R$ would need to have the value determined by $V = IR$. Or, if $R$ is chosen arbitrarily, then $I$ and/or $\lambda$ would need to be adjusted so that $V = IR$.

 

[hutchphd]

This is a very bad problem (or at least badly stated). May I inquire from the OP the exact origin?
What is the answer, please, for the Poynting vector in part b?