- #1
Jurgen M
I need proof how find average height of half circle?
Lets say pressure distribution is half circle with Pmax = radius,I must find average/resultant pressure..
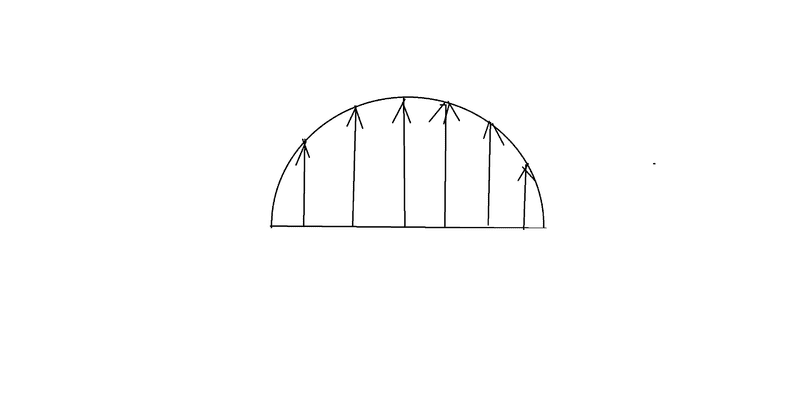
Lets say pressure distribution is half circle with Pmax = radius,I must find average/resultant pressure..
Last edited by a moderator: