Question re Galaxy Rotation Curve

[Buzz Bloom]

The diagram below is from https://en.wikipedia.org/wiki/Galaxy_rotation_curve .

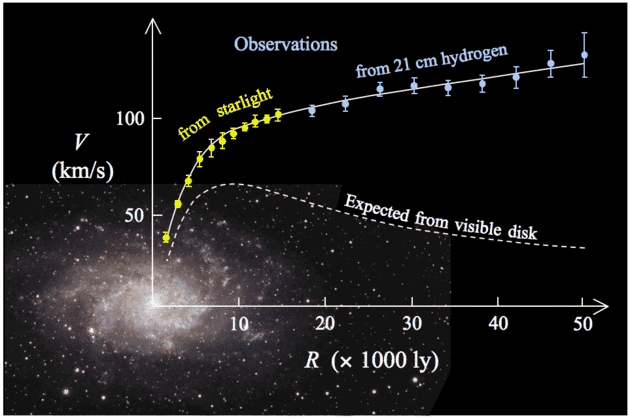
I would much appreciate a derivation explaining the shape of the "Expected from visible disk" curve in the diagram. Naively, based on Newtonian mechanics for the orbital velocity of a circular orbit,

V = √GM/R ∝ 1/R^1/2 .​

Obviously, this is not the shape of the diagram curve. I suppose the diagram curve towards the right might be close to the above formula, but what is the derivation for the shape of the left part of the curve?

 

[Orodruin]

The formula you quote is only valid outside the mass distribution (assumed spherically symmetric). In a galaxy, the stars are orbiting inside the mass distribution itself (they are part of it!).

 

[Buzz Bloom]

The formula you quote is only valid outside the mass distribution (assumed spherically symmetric). In a galaxy, the stars are orbiting inside the mass distribution itself (they are part of it!).

Hi @Orodruin:

Thanks for your post. I apologize for being vague in specifying the information I was seeking.

I interpret your quote as implying that projected onto the primary plane of a spiral galaxy, the "disk" is assumed to have a 2D radially symmetric distribution of baryonic mass, including star mass, dust, and gas, say ρ_b(R). Then the mass M(R) for r < R is

M(R) = ∫₀^R π r ρ_b(r) dr .​

The total mass of the baryonic matter in the galaxy would then be M(∞).

Q1: Is there some theory that produces a calculation of the function ρ_b(R) given M(∞)? If so, what is it, and what is this function ρ_b(R) that the theory produces?
I have tried to find the answer to this on the internet, but, if it is there, my research skills are inadequate to find it.

Given ρ_b(R), I know how to write down a complicated integral for the value of the gravitational acceleration A_b(R) of a test particle at radius R based on this distribution. I am guessing that to calculate the function of V(R) for the lower curve in the diagram, it would be first necessary to calculate the value of this integral A_b(R). Then V(r) = √A_b(R)/R.

Q2: Please cite a reference, if you know of one, that shows the calculation of A_b(R) given ρ_b(R).

Regards,
Buzz

 

[Buzz Bloom]

CORRECTIONS

M(R) = ∫₀^R 2 π r ρ_b(r) dr

V(R) = √(A_b(R)/R)​

ADDITION

Here is the integral form of A_b(R).

A_b(R) = (G/2π) ∫₀^R ρ_b(r) ∫₀^2π [(r-R cos θ)/(R²+r²-2 r R cos θ)^3/2] dθ dr​