Smallest perpendicular velocity to orbit

[unscientific]

Homework Statement

Distance from Earth to sun = 1.50 * 10¹¹m
Earth's orbital period = 3.16 * 10⁷ s

Distance from venus to sun = 1.08 * 10¹¹m
Venus's orbital period = 1.94 * 10⁷ s

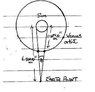
Both orbits around the sun are coplanar, and the transit of venus about the sun is observed by a telescope on earth.QN: To calculate the smallest perpendicular velocity to its orbit, required by venus so that the next expected transit does not occur.

Homework Equations

Since venus and Earth are orbiting at different angular velocities, (with venus moving faster)
Relative period = 5.02 * 10⁷ s

Therefore, time interval between successful transists = 5.02 * 10⁷ s

The Attempt at a Solution

http://img200.imageshack.us/img200/2987/earthvenus.jpg"

The angle theta was found to be 0.00928 rad.

In order for the next transit not to occur (say originally both Earth venus are along the left line that connects to left extreme end of sun), in the 5.02 * 10⁷ s that venus spends traveling relative to earth, it must be displaced by an angle of 0.00928 rad for venus to 'escape' being caught in the camera's frame that is pointing to the sun.But the answer says that " If during the interval between transits venus has a velocity v_s perpendicular to its orbit and as a result, is displaced by (1/2)(0.00928) rad as observed from Earth (half aug. diameter of of sun at earth) , venus will not pass in front of the sun"

My question is - why only half the angle?!

The original question can be found here at Qn 8:
Qn paper: http://www.physics.ox.ac.uk/olympiad/Downloads/PastPapers/BPhO_Paper2_2005_QP.pdf

Answers:
http://www.physics.ox.ac.uk/olympiad/Downloads/PastPapers/BPhO_Paper2_2005_MS.pdf

Really appreciate any help offered!

 

[omoplata]

If the orbits of Venus and Earth are coplanar when the first transit happens, Venus will pass through the exact centre of the image of the Sun. It only need to be displaced by half the angular diameter of the sun as seen from the Earth to not be Silhouetted by the Sun at the next expected transit. That's (1/2)(0.00928) rad.

 

[unscientific]

If the orbits of Venus and Earth are coplanar when the first transit happens, Venus will pass through the exact centre of the image of the Sun. It only need to be displaced by half the angular diameter of the sun as seen from the Earth to not be Silhouetted by the Sun at the next expected transit. That's (1/2)(0.00928) rad.

If venus and Earth are aligned, through the Earth's lenses won't venus appear to be at the leftmost edge of the sun's diameter and not the centre?

the left picture shows the 3 planets, while the right picture shows what it looks like when viewed from earth.

 

[omoplata]

It'll still pass through the centre of the sun as seen from Earth (pass from left to right through the centre of the sun).

Velocity component perpendicular to the plane of orbit means that velocity component is directed either up or down as seen from the earth.

 

[unscientific]

It'll still pass through the centre of the sun as seen from Earth (pass from left to right through the centre of the sun).

Velocity component perpendicular to the plane of orbit means that velocity component is directed either up or down as seen from the earth.

oh i get it now! All venus needs to do is to move upwards/downwards by half of sun's diameter so it would be hidden behind! (Since originally Earth and venus are always on the same plane that passes through the centre of the sun) Without the vertical velocity, venus will always pass through from left to right of the sun's centre plane. Thank you!

 

[omoplata]

hmmm i thought that the transit starts when venus appears at the leftmost diameter of the sun (at the edge) and not when it is at the centre of the sun?

Yes, the transit starts at the leftmost diameter of the sun. But if Earth, Sun and Venus are coplanar (in the same plane), will Venus pass through the exact center of the Sun as seen from Earth or not? What do you think?

I even if it moves up or down, why must it be half a diameter in the vertical direction? It would make more sense if it was displaced rightwards by half a diameter? that's one part i didnt get about the perpendicular velocity!

The question says velocity component perpendicular to its orbit. If Venus passes from left to right in front of the Sun as seen from the Earth, that means the plane is horizontal. So a direction perpendicular to that plane would be vertical as seen from the Earth.

 

[omoplata]

Oh, it seems I took too long to post.

You're welcome.