- #1
opus
Gold Member
- 717
- 131
Please see the attached image which is presented in my text. This is the end step after using the Rational Zeros Theorem to find possible rational zeros, testing by synthetic division, and then factoring.
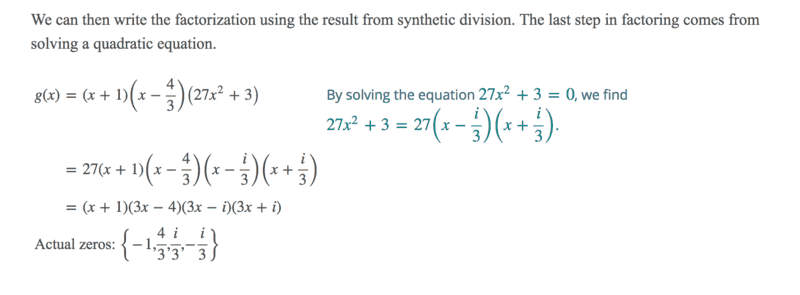
What I don't understand here is that we have the term
##27\left(x+1\right)\left(x-\frac{4}{3}\right)\left(x-\frac{i}{3}\right)\left(x+\frac{i}{3}\right)##
This the 27 is broken up into 3⋅3⋅3 and distributed to three of the four other factors to give us ##\left(x+1\right)\left(3x-4\right)\left(3x-i\right)\left(3x+i\right)##
The zero's are then found to be as listed.
Why was this necessary?
##27\left(x+1\right)\left(x-\frac{4}{3}\right)\left(x-\frac{i}{3}\right)\left(x+\frac{i}{3}\right)## gives us the exact same zeros as is. So what was the purpose of this step?
What I don't understand here is that we have the term
##27\left(x+1\right)\left(x-\frac{4}{3}\right)\left(x-\frac{i}{3}\right)\left(x+\frac{i}{3}\right)##
This the 27 is broken up into 3⋅3⋅3 and distributed to three of the four other factors to give us ##\left(x+1\right)\left(3x-4\right)\left(3x-i\right)\left(3x+i\right)##
The zero's are then found to be as listed.
Why was this necessary?
##27\left(x+1\right)\left(x-\frac{4}{3}\right)\left(x-\frac{i}{3}\right)\left(x+\frac{i}{3}\right)## gives us the exact same zeros as is. So what was the purpose of this step?