- #1
chompysj
- 19
- 0
Hi, this is my first post, so please tell me if I'm doing anything incorrectly...thanks.
Three blocks are connected, as shown in Fig. 5-47, on a horizontal frictionless table and pulled to the right with a force T3 = 64.9 N.
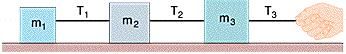
Assume that m1 = 12.1 kg, m2 = 24.5 kg, and m3 = 32.7 kg.
a = (net force) / (total mass)
T = (a) x (mass)
I'm supposed to find the tensions at T1 and T2. I got the answer to T1 by multiplying the acceleration (0.94 m/s^2) by the mass of m1 (12.1 kg) and got a correct answer. However, when I tried doing the same thing for T2 (multiplying 0.94 m/s^2 by the mass of M2, 24.5 kg), the answer was wrong.
My question: why can't I find T2 the same way I found T1? Is there a difference between T1 and T2?
Homework Statement
Three blocks are connected, as shown in Fig. 5-47, on a horizontal frictionless table and pulled to the right with a force T3 = 64.9 N.
Assume that m1 = 12.1 kg, m2 = 24.5 kg, and m3 = 32.7 kg.
Homework Equations
a = (net force) / (total mass)
T = (a) x (mass)
The Attempt at a Solution
I'm supposed to find the tensions at T1 and T2. I got the answer to T1 by multiplying the acceleration (0.94 m/s^2) by the mass of m1 (12.1 kg) and got a correct answer. However, when I tried doing the same thing for T2 (multiplying 0.94 m/s^2 by the mass of M2, 24.5 kg), the answer was wrong.
My question: why can't I find T2 the same way I found T1? Is there a difference between T1 and T2?
Last edited: