- #1
unscientific
- 1,734
- 13
Hi guys, I'm doing a quantum course at the moment, and there's one thing in Binney's book which I don't really understand:
Why must you multiply the radial eigenfunction by ##Y_l^m## to get the "complete wavefunction" ?
They do this to normalize the eigenfunction, and to do things like calculate mean energies and mean distance from the nucleus:
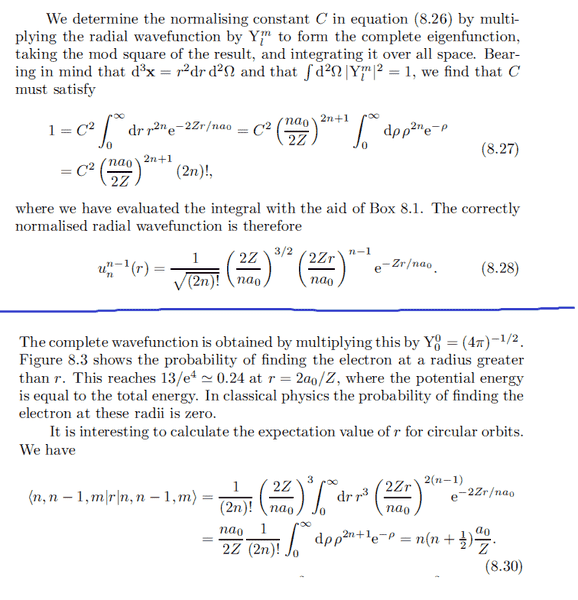
Why must you multiply the radial eigenfunction by ##Y_l^m## to get the "complete wavefunction" ?
They do this to normalize the eigenfunction, and to do things like calculate mean energies and mean distance from the nucleus:
Last edited: