Trouble with infinity and complex numbers

[Troxx]

TL;DR Summary

Trouble with infinity and complex numbers, just curious.

Summary: Trouble with infinity and complex numbers, just curious.

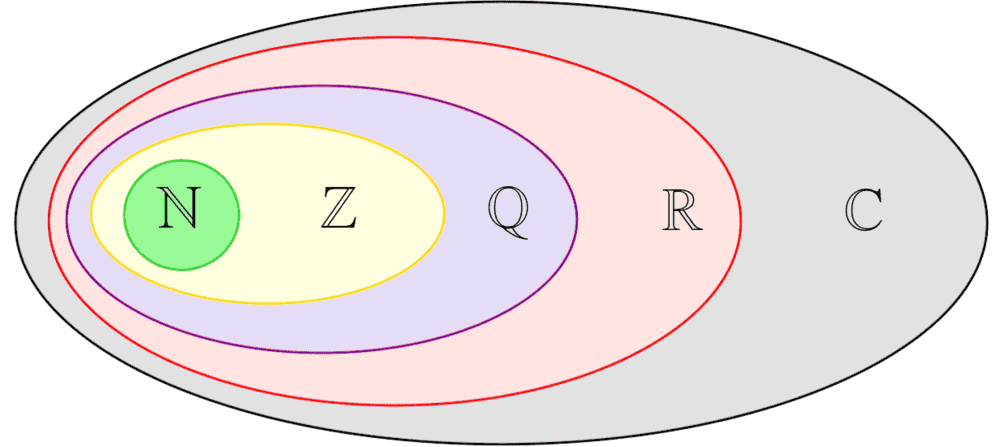
I'm not too familiar with set theory ... but <-∞, ∞> contains just real numbers?
Does something similar to <-∞, ∞> exist in Complex numbers?
My question, is it "wrong"?

 

[.Scott]

Yup, using your nomenclature, it would be (<-∞, ∞>, <-∞, ∞>).
Or a+bi where a and b are real numbers.

But one item of caution: ∞ is not a number - real or otherwise.

 

[Troxx]

Thanks...
And further.. when plotting functions with a imaginary axis... there can be more imaginary axis' than one I guess..

 

[.Scott]

Thanks...
And further.. when plotting functions with a imaginary axis... there can be more imaginary axis' than one I guess..

If you are plotting a function with both a complex range and domain, you would need four dimensions: a+bi=f(c+di) - one each for a, b, c, and d with b and d being imaginary axis. Of course, drawing in four dimensions requires some creativity.

 

[Michael Price]

Thanks...
And further.. when plotting functions with a imaginary axis... there can be more imaginary axis' than one I guess..

For complex numbers there is only one imaginary axis, for quarternions there are three and for octonions there are seven.
https://en.m.wikipedia.org/wiki/Octonion

 

[jbriggs444]

If you are plotting a function with both a complex range and domain, you would need four dimensions: a+bi=f(c+di) - one each for a, b, c, and d with b and d being imaginary axis. Of course, drawing in four dimensions requires some creativity.

If you are going to build a graph, you need four dimensions for the domain and another two for the range.

 

[WWGD]

The thing is that there is a different way of "Approaching Infinity" as you have additional dimensions. In $\mathbb R$ , as you pointed out, you go along the +, - x-axis far right or left respectively. In , e.g., $\mathbb R^2$, your set is unbounded if it is not contained in a ball of finite radius r. Similar in higher dimensions, where being contained in a ball of finite radius is equivalent to being bounded, while you "Go to infinity" by not being contained in balls of finite radius.

 

[Mark44]

If you are going to build a graph, you need four dimensions for the domain and another two for the range.

Is this really what you meant? For a function $f : \mathbb C \to \mathbb C$? You have an extra two dimensions. For the domain you need only two dimensions, not four.

 

[mfb]

For complex numbers it is better to add a single infinity to get the Riemann sphere.

 

[WWGD]

There is a lot of material on compactifications of 1,2, etc points.

 

[Delta2]

There is a way to "graph" a complex valued function similar to the way we graph a 2D vector field in just 2 dimensions. That is at every point of the domain (x,y) we plot an arrow which represents a vector that has its y-component equal to the Imaginary part of f(x+iy) and its x-component equal to the Real part of f(x+iy). I know this is not exactly a graph as we usually mean it but anyway..

Alternatively we can use 3 dimensions (where the z axis represent the real or the imaginary part of f(x+iy) )and do two graphs, one for the imaginary part and one for the real part.

 

[jbriggs444]

Is this really what you meant? For a function $f : \mathbb C \to \mathbb C$? You have an extra two dimensions. For the domain you need only two dimensions, not four.

Oops. I'd misread a function of two complex arguments.

 

[Mark44]

Oops. I'd misread a function of two complex arguments.

I thought that might be it.