- #1
EthanVandals
- 55
- 2
Member advised to use the homework template for posts in the homework forums.
Here is all the work I have done so far (hopefully it is right). My brain somewhat shuts down when it comes to ratios.
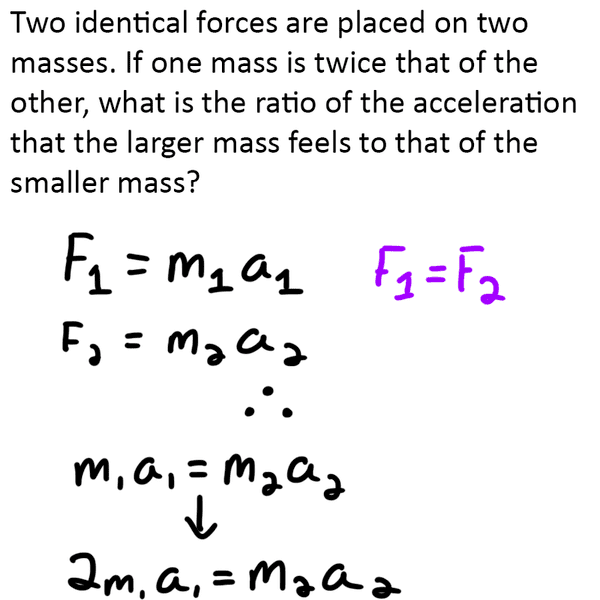
So something more like this then? I got this far, then got confused again as to what kind of ratio I'm supposed to get since I'm not given any values. If I divided by M2, then it would give me (2)A1=A2...so is that saying that the mass that is double the size of the other one is going to accelerate at half the speed of the smaller mass? And would the ratio then be 2:1? Thanks for all your help! :)TomHart said:
EthanVandals said:
The acceleration of the masses is directly proportional to the magnitude of the forces applied. This means that if the magnitude of the forces increases, the acceleration of the masses will also increase.
In this scenario, the acceleration of the masses is inversely proportional to their masses. This means that if one mass is increased, the acceleration will decrease, and vice versa.
Yes, the forces can be in opposite directions and still have the same effect on the masses. This is because the magnitude of the forces is more important in determining the acceleration, rather than the direction.
The distance between the masses has an inverse square relationship with the force. This means that as the distance between the masses increases, the force decreases, and vice versa.
In this scenario, the direction of the forces is not as important as the magnitude. As long as the forces are equal in magnitude, the direction will not affect the acceleration of the masses.