- #1
Diff.Ed
- 5
- 0
Homework Statement
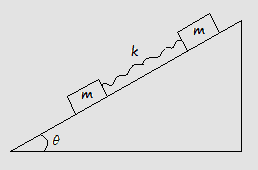
Two blocks both of mass [itex]m[/itex] are placed on an inclined plane making an angle [itex]\theta[/itex] with the ground. The two masses are connected with a spring of spring constant [itex]k[/itex]. The coefficient of kinetic friction is [itex]\mu _k[/itex] for both blocks. Assume that the spring is initially stretched to a length [itex]L+x_0[/itex] where [itex]L[/itex] is its equilibrium length when it is at rest on a flat surface. Find the displacement of the two blocks ALONG THE INCLINE as a function of time,t, assuming that at that particular time the spring is still stretched. (Hint : Call the displacement of the leading block along the inclined plane [itex]x_1[/itex]. Write the displacement of the trailing block in terms of [itex]x_1[/itex] and stretch in the spring, [itex]x[/itex]. You will have two time-dependent equations in two unknowns.)
Related Equations
F=-kx (Hooke's Law), differential equations
The attempt at a solution
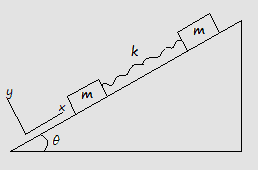
[itex]m\frac{d^2x_1}{dt^2}=-k(x_1+x_0)-mg\sin\theta+\mu _k mg\cos\theta[/itex]and general solution of this diff. equation
I couldn't continue anymore. Could you help me ?
Two blocks both of mass [itex]m[/itex] are placed on an inclined plane making an angle [itex]\theta[/itex] with the ground. The two masses are connected with a spring of spring constant [itex]k[/itex]. The coefficient of kinetic friction is [itex]\mu _k[/itex] for both blocks. Assume that the spring is initially stretched to a length [itex]L+x_0[/itex] where [itex]L[/itex] is its equilibrium length when it is at rest on a flat surface. Find the displacement of the two blocks ALONG THE INCLINE as a function of time,t, assuming that at that particular time the spring is still stretched. (Hint : Call the displacement of the leading block along the inclined plane [itex]x_1[/itex]. Write the displacement of the trailing block in terms of [itex]x_1[/itex] and stretch in the spring, [itex]x[/itex]. You will have two time-dependent equations in two unknowns.)
Related Equations
F=-kx (Hooke's Law), differential equations
The attempt at a solution
[itex]m\frac{d^2x_1}{dt^2}=-k(x_1+x_0)-mg\sin\theta+\mu _k mg\cos\theta[/itex]
[itex]x_1 = A\sin(\sqrt{k/m}t) + B\cos(\sqrt{k/m}t) - \frac{kx_0+mg\sin\theta+\mu _k mg\cos\theta}{k}[/itex]
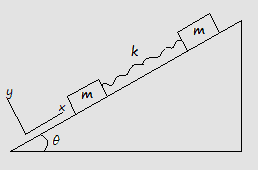
[itex]m\frac{d^2x_2}{dt^2}=k(x_1+x_0)-mg\sin\theta+\mu _k mg\cos\theta[/itex]
[itex]m\frac{d^2x_2}{dt^2}=k(x_1+x_0)-mg\sin\theta+\mu _k mg\cos\theta[/itex]
I couldn't continue anymore. Could you help me ?
Last edited: