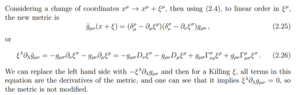dwd40physics
- 32
- 0
- TL;DR Summary
- understanding coordinate transformations of metric and killing vectors
Hi, I am reading through my lecture notes - I haven't formally covered killing vectors but it was introduced briefly in lectures.
Reading through the notes has highlighted something I am not sure about when it comes to co-ordinate transformations.
Q1.Can someone explain how to go from ##\tilde{g}_{uv}## to ##g_{uv} ##
The bit where it says: we can replace the left hand side with ##-\xi^{\lambda}\partial_{\lambda} g_{uv}##
Q2. more basic question how does ##\tilde{g}_{uv}## differ to ##g_{uv} ## ? how does a prime function differ from un-primed functions ?
Q3. what does ##\xi^{\lambda}\partial_{\lambda} g_{uv} = 0 ## tell us ?
thanks :-)
Reading through the notes has highlighted something I am not sure about when it comes to co-ordinate transformations.
Q1.Can someone explain how to go from ##\tilde{g}_{uv}## to ##g_{uv} ##
The bit where it says: we can replace the left hand side with ##-\xi^{\lambda}\partial_{\lambda} g_{uv}##
Q2. more basic question how does ##\tilde{g}_{uv}## differ to ##g_{uv} ## ? how does a prime function differ from un-primed functions ?
Q3. what does ##\xi^{\lambda}\partial_{\lambda} g_{uv} = 0 ## tell us ?
thanks :-)
Attachments
Last edited: