- #1
lucas7
- 9
- 0
or why is
 ?
?
thx in advance!
thx in advance!
lucas7 said:or why is?
thx in advance!
lucas7 said:I don't understand why.
lucas7 said: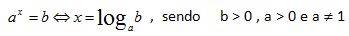
lurflurf said:Logarithms can be used to define exponentiation as
[itex]u(x)^{v(X)}=e^{v(x) \log(u(x))}[/itex]
Otherwise it can be proved as a theorem.
Note also that e^x is continuous is used in your example to justify moving the limit past e.
micromass said:OK, so you're saying that [itex]x=ln(b)[/itex] iff [itex]e^x=b[/itex].
So take an arbitrary b. Then we can of course write [itex]ln(b)=ln(b)[/itex]. Define [itex]x=ln(b)[/itex]. The "iff" above yields directly that [itex]b=e^x = e^{ln(b)}[/itex].
lucas7 said:I got it. But I fail to apply it for my case, when x has an exponential. Like [tex]{x}^{1/x}={e}^{(1/x)lnx}[/tex]
micromass said:So you understand why [itex]b=e^{ln(b)}[/itex]?? Good. Now apply it with [itex]b=x^{1/x}[/itex]. Then you get
[tex]x^{1/x} = e^{ln\left(x^{1/x}\right)}[/tex]
Do you agree with this? Now apply the rules of logarithms: what is [itex]ln(a^b)=...[/itex]. Can you apply this identity with a=x and b=1/x ?
lucas7 said:[tex]x=ln({x}^{1/x})[/tex]
micromass said:Why is this true? This isn't correct for all x.
x^(1/x) is an exponential expression where the base (x) is raised to the power of the reciprocal of itself (1/x). It can also be written as x^(1/x) = e^((1/x)lnx).
e is a mathematical constant that is approximately equal to 2.71828. It is the base of the natural logarithm and is often used in exponential and logarithmic functions.
This is a property of logarithmic and exponential functions. When a logarithmic function and an exponential function have the same base, they cancel each other out and result in the original base. In this case, the base is x and the logarithm and exponential functions have the same base of e.
e^((1/x)lnx) is a special form of the exponential function that is used to solve equations involving exponents and logarithms. It is also commonly used in calculus and other advanced mathematical concepts.
Yes, x^(1/x) can be simplified further to e. This is because as x approaches infinity, the expression approaches the value of e. However, for any finite value of x, x^(1/x) cannot be simplified any further.