072 is Q(theta) a linear transformation from R^2 to itself.

[karush]

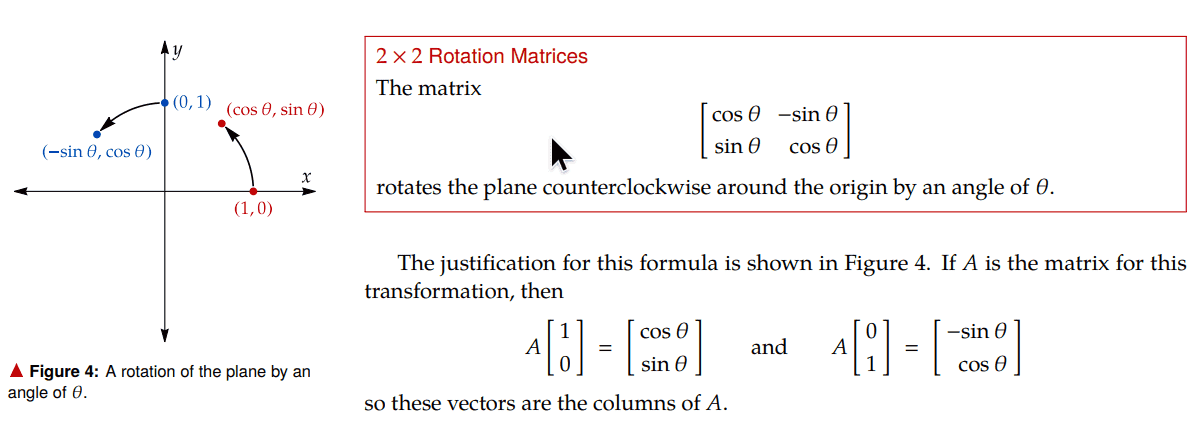
if $Q(\theta)$ is

$\left[\begin{array}{rr}
\cos{\theta}&- \sin{\theta}\\
\sin{\theta}&\cos{\theta}
\end{array}\right]$

how is $Q(\theta)$ is a linear transformation from R^2 to itself.

ok I really didn't know a proper answer to this question but presume we would need to look at the unit circle

not sure if this helps

 

[karush]

Ok thanks
I usually don't get much replies on these linear algebra posts

 

[skeeter]

Ok thanks
I usually don't get much replies on these linear algebra posts

I'd help out, but it's been since my sophomore year in school (1973) since I've taken a course in Linear Algebra ... except for the very basic stuff, I haven't used it so I've "losed" it.

 

[HOI]

Better is to understand what "linear transformation" means! ANY transformation that can be written as a matrix multiplication is linear!

A transformation, L, on a vector space is "linear" if and only if
L(u+ v)= Lu+ Lv, for any vectors u and v, and
L(au)= aLu, for any vector u and scalar, a.

Here if $u= \begin{pmatrix}x \\ y \end{pmatrix}$ and $v= \begin{pmatrix} a \\ b\end{pmatrix}$, $L(u+ v)= \begin{pmatrix} cos(\theta) & -sin(\theta) \\ sin(\theta) & cos(\theta)\end{pmatrix}\begin{pmatrix}x+ a \\ y+ b\end{pmatrix}= \begin{pmatrix}(x+ a)cos(\theta)- (y+ b)sin(\theta) \\ (x+ a)sin(\theta)+ (y+ b)cos(\theta)\end{pmatrix}$.

While $Lu+ Lv= \begin{pmatrix} cos(\theta) & -sin(\theta) \\ sin(\theta) & cos(\theta)\end{pmatrix}\begin{pmatrix}x \\ y \end{pmatrix}+ \begin{pmatrix} cos(\theta) & -sin(\theta) \\ sin(\theta) & cos(\theta)\end{pmatrix}\begin{pmatrix} a \\ b \end{pmatrix}= \begin{pmatrix} xcos(\theta)- ysin(\theta) \\ xsin(\theta)+ y cos(\theta)\end{pmatrix}+ \begin{pmatrix} acos(\theta)- bsin(\theta) \\ asin(\theta)+ bcos(\theta)\end{pmatrix}= \begin{pmatrix}(x+ a)cos(\theta)- (y+ b)sin(\theta) \\ (x+ a)sin(\theta)+ (y+ b)cos(\theta)\end{pmatrix}$.

And $L(au)= \begin{pmatrix} cos(\theta) & -sin(\theta) \\ sin(\theta) & cos(\theta)\end{pmatrix}\begin{pmatri
https://mathhelpboards.com/forums/-/create-thread
072 is Q(theta) is a linear transformation from R^2 to itself.

• Thread starterkarush
• Start datehttps://mathhelpboards.com/threads/072-is-q-theta-is-a-linear-transformation-from-r-2-to-itself.28497/

https://mathhelpboards.com/threads/072-is-q-theta-is-a-linear-transformation-from-r-2-to-itself.28497/#post-124753https://mathhelpboards.com/threads/072-is-q-theta-is-a-linear-transformation-from-r-2-to-itself.28497/watch
https://mathhelpboards.com/threads/072-is-q-theta-is-a-linear-transformation-from-r-2-to-itself.28497/post-124753

• Thread starter
• New
• https://mathhelpboards.com/posts/124753/bookmark
• https://mathhelpboards.com/threads/072-is-q-theta-is-a-linear-transformation-from-r-2-to-itself.28497/post-124753

https://mathhelpboards.com/data/avatars/s/0/55.jpg?1588030209
karush
Well-known member
Jan 31, 2012 2,838
if Q(θ)Q(θ) is

[cosθsinθ−sinθcosθ][cos⁡θ−sin⁡θsin⁡θcos⁡θ]

how is Q(θ)Q(θ) is a linear transformation from R^2 to itself.

ok I really didn't know a proper answer to this question but presume we would need to look at the unit circle

not sure if this helps

Last edited by a moderator: Today at 12:07 AM
https://mathhelpboards.com/posts/124753/react?reaction_id=1 https://mathhelpboards.com/threads/072-is-q-theta-is-a-linear-transformation-from-r-2-to-itself.28497/reply?quote=124753 https://mathhelpboards.com/threads/072-is-q-theta-is-a-linear-transformation-from-r-2-to-itself.28497/reply?quote=124753
https://mathhelpboards.com/posts/124753/report

x}ax \\ ay \end{pmatrix}= \begin{pmatrix}ax coz

 

[karush]

I'd help out, but it's been since my sophomore year in school (1973) since I've taken a course in Linear Algebra ... except for the very basic stuff, I haven't used it so I've "losed" it.

wow... my senior year was 1970 but my highest level in math was algebra II which today is much more advanced