Application of Supremum Property .... Garling, Remarks on Theorem 3.1.1

[Math Amateur]

TL;DR Summary

Concerns properties of the positive real numbers including an explicit procedure for determining a rational r with $x \lt r \lt y$ where $x \gt 0$ ...

I am reading D. J. H. Garling's book: "A Course in Mathematical Analysis: Volume I: Foundations and Elementary Real Analysis ... ...

I am focused on Chapter 3: Convergent Sequences

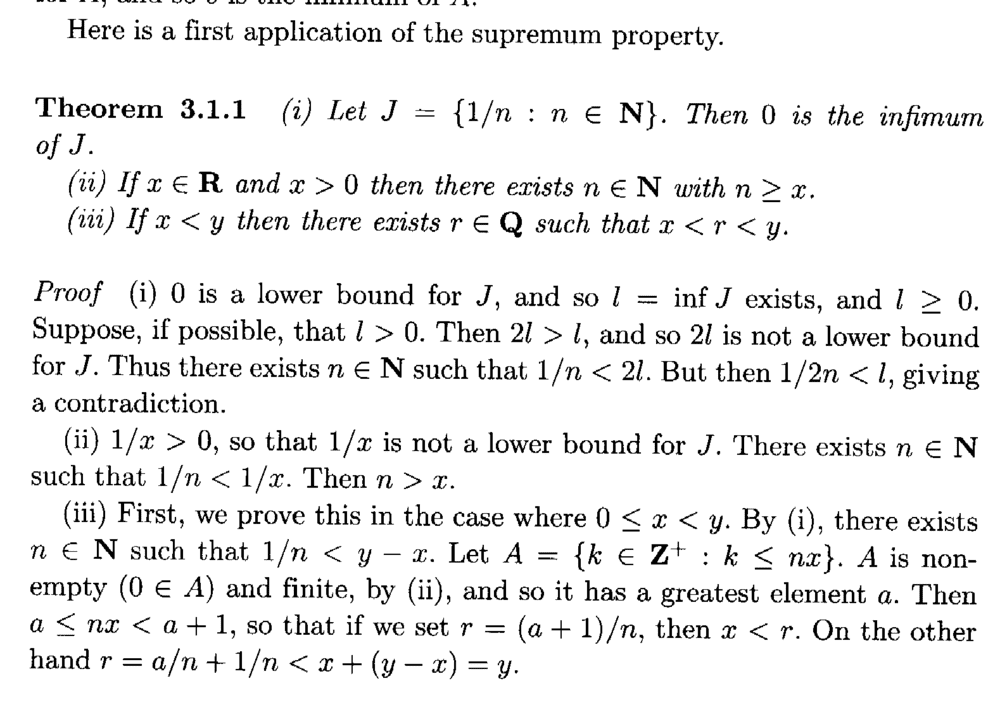
I need some help to fully understand some remarks by Garling made after the proof of Theorem 3.1.1 ...Garling's statement and proof of Theorem 3.1.1 (together with the interesting remarks) reads as follows:

My questions on the remarks after the proof are as follows:
Question 1

In the remarks after the proof of Theorem 3.1.1 we read the following:

" ... ... There exists a least positive integer, $q_0$, say, such that $1/q_0 \lt y - x$ ... ... "Can someone please explain exactly (rigorously) why this is true ... ..

Question 2

In the remarks after the proof of Theorem 3.1.1 we read the following:

" ... ... and there then exists a least integer,$p_0$, say, such that $x \lt p_0 / q_0$ ... ..."Can someone please explain exactly (rigorously) why this is true ... ..
Question 3

In the remarks after the proof of Theorem 3.1.1 we read the following:

" ... ... Then $x \lt p_0 / q_0 \lt y$ and $r_0 = p_o / q_0$ is uniquely determined ... ... "Can someone please explain exactly (rigorously) why this is true ... ..Help will be appreciated ...

Peter

 

[member 587159]

Have you established the archimedian property at this point?

 

[Math Amateur]

The Archimedian Property is proved as Part (ii) of the proof of Theorem 3.1.1 ... so I think that as far as the remarks after the theorem are concerned we can take the Archimedian Property as proved ... ...

Peter

 

[member 587159]

Q1: We prove there is an positive integer $n$ such that $1/n < y-x$. Suppose not, then for all positive integers we have $1/n \geq y-x$ and thus $n \leq 1/(y-x)$ for all positive integers $n$, contradicting archimedian principle.

Now that we know that there is such an integer, we can take it minimal and we call it $p_0.$

I guess the other questions are proven similarly. Let me know if this helps. I find these questions always difficult to answer because I use these properties all the times.

 

[Math Amateur]

Q1: We prove there is an positive integer $n$ such that $1/n < y-x$. Suppose not, then for all positive integers we have $1/n \geq y-x$ and thus $n \leq 1/(y-x)$ for all positive integers $n$, contradicting archimedian principle.

Now that we know that there is such an integer, we can take it minimal and we call it $p_0.$

I guess the other questions are proven similarly. Let me know if this helps. I find these questions always difficult to answer because I use these properties all the times.

Thanks Math_QED ...

Yes ... get the idea ...

Peter