- #1
PainterGuy
- 940
- 69
- TL;DR Summary
- I found the formulas in two different sources for y-coordinate of center of gravity for a homogeneous lamina differ from each other by a factor "1/2".
Hi,
In one of the standard calculus textbooks, source #1, the formula for y-coordinate of center of gravity for a homogeneous lamina is given as follows.
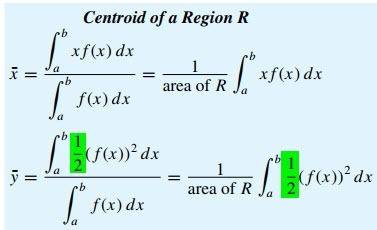
In another book of formulas, source #2, the formula is given without the factor "1/2" as is shown below. Personally, I believe that source #1 is correct. I just wanted to confirm so that I could notify the publisher of source #2 of erratum. Could you please help me with it? Thank you!
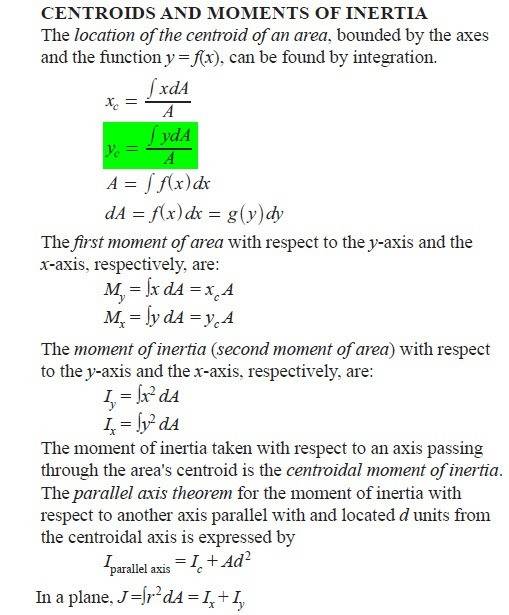
In one of the standard calculus textbooks, source #1, the formula for y-coordinate of center of gravity for a homogeneous lamina is given as follows.
In another book of formulas, source #2, the formula is given without the factor "1/2" as is shown below. Personally, I believe that source #1 is correct. I just wanted to confirm so that I could notify the publisher of source #2 of erratum. Could you please help me with it? Thank you!

