Clarification of Notation - Fourier decomposition of fields in QFT

[Wrichik Basu]

I am studying QFT from A First Book of QFT. It is a very well-written book. However, due to some personal reasons, I cannot buy the printed book at this moment. So I borrowed this book from a person (who, in turn, borrowed it from his university library), and scanned it. Everything is fine except the notation at some places, due to the low scan resolution.

The authors write at the beginning of the book that $p$ means a 4-vector, p means a 3-vector, and $\mathbf{p}$ means the magnitude of the 3-vector.

With this in place, for the Fourier decomposition of the Dirac field, the authors write:

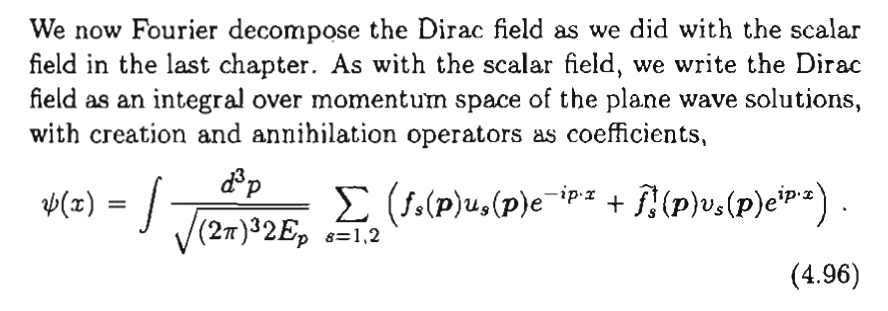
Here, the integration variable is $p$ (the four-vector), the arguments of the creation and annihilation operators for the particle and antiparticle ($f_s$ and $\hat{f_s ^\dagger}$) and the spinors ($u_s$ and $v_s$) is p, the 3-vector.

In the expression in the exponent, is it the 4-vectors or the 3-vectors that are being dotted?

A similar problem occurs for photon fields:

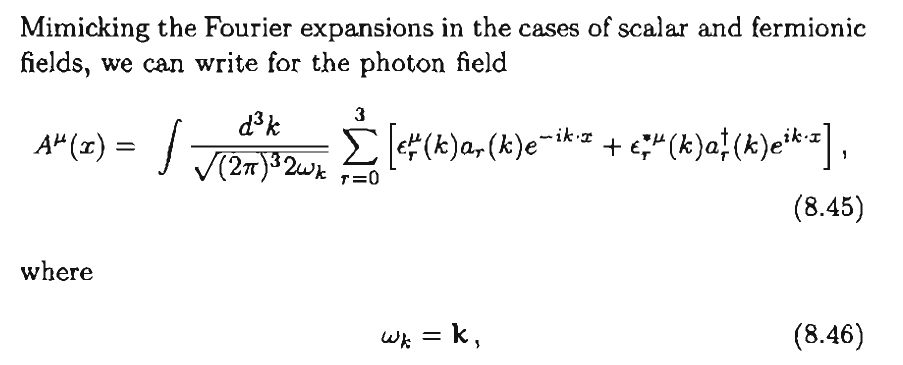
Again, is the argument in the exponent the 4-vectors or 3-vectors?

Unfortunately, at this moment I can no longer access the print book. It will be very helpful if someone clarifies the notation.

 

[vanhees71]

I find your scan pretty good. I prefer to write $p$ for the four-vector $\vec{p}$ for its spatial components (everything in a given fixed inertial reference frame defining the basis of four Minkowski-orthogonal unit vectors).

You are dealing with free quantum fields and thus can decompose it in terms of the momentum-spin (momentum-polarization) annihilation and creation operators of the corresponding single-particle basis.

Let's take the most simple case of scalar bosons first, i.e., the spin is 0 and thus there's only a momentum for the single-particle basis. The field operator fulfills the Klein-Gordon equation
$$(\Box + m^2) \hat{\phi}(x)=0,$$
which implies that the four-momentum's time component is completely determined by the three spacelike components and by definition we always choose the positive solution to label the plane-wave field modes, i.e.,
$$p^0=+\sqrt{\vec{p}^2+m^2}=\omega(\vec{p)}.$$
Note that I work in natural units with $\hbar=c=1$, and I use the west-coast convention for the Minkowski pseudometric, i.e., $(\eta_{\mu \nu})=(\eta^{\mu \nu})=\mathrm{diag}(1,-1,-1,-1)$.

Now since the KG equation allows field modes with both positive and negative frequencies, the most general solution is (with $x^0=t$) [Edit: Typo corrected in view of #5]
$$\hat{\phi}(x)=\hat{\phi}(t,\vec{x}) = \int_{\mathbb{R}^3} \frac{\mathrm{d}^3 \vec{p}}{\sqrt{(2 \pi)^3 2 \omega(\vec{p})}} \left [ \hat{a}(\vec{p}) \exp(-\mathrm{i} p \cdot x) + \hat{b}^{\dagger}(\vec{p}) \exp(+\mathrm{i} p \cdot x \right]_{k^0 =\omega(\vec{k})}.$$
The factor in front is just for convenience and chosen such that the non-trivial commutator relations for the annihilation and creation operators become as simple as possible (i.e., such that the one-particle states created by $\hat{a}^{\dagger}(\vec{p})$ and one-antiparticle states created by $\hat{b}^{\dagger}(\vec{p})$ from the vacuum state are normalized by the $\delta$ distribution without any additional factors; that's what obviously also your book does, but not all authors follow this convention):
$$[\hat{a}(\vec{p}),\hat{a}(\vec{p}')]=\delta^{(3)}(\vec{p}-\vec{p}').$$

The same holds for the photon operator, but here you also have polarization vectors. Again each wave-number four-vector obeys the on-shell condition. In this case since photons are massless you have
$$k^2=0 \; \Rightarrow \; k^0=\omega(\vec{k})=\sqrt{\vec{k}^2}=|\vec{k}|.$$
Now obviously the book follows the Gupta-Bleuler method, i.e., it defines four polarization tensors (leaving the gauge before quantization completely unfixed).

Then you need to define four polarization four-vectors. Conveniently they are chosen as a "four-bein", i.e., three Minkowski-orthonormal vectors but dependent on the vector $\vec{k}$, such that
$$(\epsilon_0^{\mu}(\vec{k}))=(1,0,0,0), \quad \epsilon_3^{\mu}(\vec{k})=(0,\vec{k}/|\vec{k}|), \quad \epsilon_{j}^{\mu}(\vec{k})=(0,\vec{\epsilon}_j(\vec{k}))$$
with $j \in \{1,2,\}$ and the $\vec{\epsilon}_j(\vec{k})$ both orthogonal to $\vec{k}$ and orthonormal to each other,
$$\vec{k} \cdot \vec{\epsilon}_j(\vec{k})=0, \quad \vec{\epsilon}_j(\vec{k}) \cdot \vec{\epsilon}_{j'}(\vec{k})=\delta_{jj'}, \quad j \in \{1,2\},$$
such that
$$\vec{\epsilon}_1(\vec{k}) \times \vec{\epsilon}_2(\vec{k})=\vec{\epsilon}_3(\vec{k})=\frac{\vec{k}}{|\vec{k}|}.$$
Then the $\epsilon_0^{\mu}(\vec{k})$ is called the time-like polarization, $\epsilon_3^{\mu}(\vec{k})$ the longitudinal and $\epsilon_j(\vec{k})$ with $j \in \{1,2\}$ the transverse polarization state.

It's of course clear that only the latter transverse polarization states are physical. As a massless field the photon field has only two physical polarization states, namely those of the transversal electromagnetic fields. For convenience of notation I've choosen real polarization vectors. Physically the transverse ones are just the usual linear polarization vectors in orthogonal polarization directions.

The field operators now are given by (taking into account that the classical em. four-potential are real four-vectors and thus the corresponding field operators have to be self-adjoint) [Edit: Typo corrected in view of #5]
$$\hat{A}^{\mu}(x) = \sum_{r=0}^3 \int_{\mathbb{R}^3} \frac{\mathrm{d}^3 \vec{k}}{\sqrt{(2 \pi)^3 2 |\vec{k}|}} \epsilon_r^{\mu}(\vec{k}) \left [\hat{a}_r(\vec{k}) \exp(-\mathrm{i} k \cdot x) + \hat{a}_r^{\dagger}(\vec{k}) \exp(+\mathrm{i} k \cdot x) \right]_{k^0=|\vec{k}|}.$$
I hope, now the notation is clarified.

 

[Wrichik Basu]

@vanhees71 Thank you. That is an informative post and answers my question completely.

I really wish the authors used $\vec{p}$ and $|\vec{p}|$. No idea why they still continue to use the math bold font.

 

[vanhees71]

Notation is a difficult issue. There are never enough symbols, accents etc. to clearly distinguish the different types of mathematical objects. In my opinion, to use bold italics and bold times symbols to have a distinct meaning is a bit too subtle to make the text well readable. I've also never heard of such an idea before. That's why I prefer this more clumpsy notation above ;-).

 

[Wrichik Basu]

@vanhees71 Can you tell me one thing? In the formula that you wrote, you took the factor inside the integral to be $$\dfrac{1}{\left[(2\pi)\ 2 |\vec{k}|\right]^{3/2}},$$ while in my book, they have taken it as $$\dfrac{1}{\left[ (2\pi)^3 \ 2 |\vec{k}|\right]^{1/2}}.$$ Basically what I am pointing out is that, you took a cube of $|\vec{k}|$ along with that of $2\pi$. So is the book wrong, or are they the same thing and I am missing something?

 

[vanhees71]

No, I am wrong :-((. I'll correct it in my original posting.

The factor comes from the "relativistic" normalization of momentum eigenmodes,
$$u_{\vec{p}}(x)=\frac{1}{[(2 \pi)^3 2 E(\vec{p})} \exp(-\mathrm{i} p \cdot x)|_{p^0=E(\vec{p})}.$$
It fulfills the covariant normalization condition, which is motivated by the four-current
$$J^{\mu}(x)=\mathrm{i} \phi^*(x) \overleftrightarrow{\partial^{\mu}} \phi(x):=\mathrm{i} \left \{\phi^*(x) \partial^{\mu} \phi(x)- [\partial^{\mu} \phi^*(x)]\phi(x) \right\}.$$
Here we need it in the form
$$\int_{\mathbb{R}^4} \mathrm{d}^4 x u_{\vec{p}'}^*(x) \overleftrightarrow{\partial^{0}} u_{\vec{p}}(x) = \delta^{(3)}(\vec{p}-\vec{p}').$$