- #1
adrms
- 5
- 1
- Homework Statement
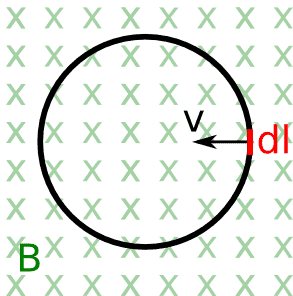
- A conductive loop is painted around a plastic balloon. A magnetic field B = 0.2 cos(4t)[T] is applied perpendicular to the equatorial plane. The balloon is contracting with a radial velocity [itex]v[/itex]. When the radius of the balloon is 0.5[m], the induced RMS voltage in the loop is 500[mV].
Find the velocity v of radial contraction in that moment.
- Relevant Equations
- $$V = \int \left(\vec{v} \times \vec{B}\right) \bullet \vec{dl} - \int _S \frac{\vec{dB}}{dt} \bullet \vec{ds}$$
$$V_{\text{rms}} = \frac{V_{\text{max}}}{\sqrt{2}}$$
$$V = \int \left(\vec{v} \times \vec{B}\right) \bullet \vec{dl} - \int _S \frac{\vec{dB}}{dt} \bullet \vec{ds}$$
From the statement I know that: B⊥v, (B x v) // dl and B // ds.
$$V = \int vBdl - \oint _S \frac{dB}{dt} ds$$
v is the speed with which all the segments dl are aproximating to the center of the loop. It is constant and does not depend on the length of the circunsference so it can be separated from the first integrand.
$$V = v \int B dl - \oint _S \frac{dB}{dt} ds$$
Can B be considered constant in the moment when radius of the loop is r=0.5?
Can I do the same with [itex]\frac{dB}{dt}[/itex] ?
In that case I will have
$$V = vB \int dl - \frac{dB}{dt} \oint _S ds$$
$$V = vB (2 \pi r) - \frac{dB}{dt} (\pi r ^2)$$
$$v = \frac{V + \frac{dB}{dt} (\pi r ^2)}{B (2 \pi r)}$$
If I replace the variables
$$v = \frac{ 500 \sqrt{2} \times 10^{-3} - 0.2 \pi \sin(4t)}{0.2 \pi \cos (4t)}$$
But I do not know the value of t so I do not know how to reach a numeric result.
---
The problem is from "Electromagnetics with Applications" 5th Edition by John Daniel Kraus
From the statement I know that: B⊥v, (B x v) // dl and B // ds.
$$V = \int vBdl - \oint _S \frac{dB}{dt} ds$$
v is the speed with which all the segments dl are aproximating to the center of the loop. It is constant and does not depend on the length of the circunsference so it can be separated from the first integrand.
$$V = v \int B dl - \oint _S \frac{dB}{dt} ds$$
Can B be considered constant in the moment when radius of the loop is r=0.5?
Can I do the same with [itex]\frac{dB}{dt}[/itex] ?
In that case I will have
$$V = vB \int dl - \frac{dB}{dt} \oint _S ds$$
$$V = vB (2 \pi r) - \frac{dB}{dt} (\pi r ^2)$$
$$v = \frac{V + \frac{dB}{dt} (\pi r ^2)}{B (2 \pi r)}$$
If I replace the variables
$$v = \frac{ 500 \sqrt{2} \times 10^{-3} - 0.2 \pi \sin(4t)}{0.2 \pi \cos (4t)}$$
But I do not know the value of t so I do not know how to reach a numeric result.
---
The problem is from "Electromagnetics with Applications" 5th Edition by John Daniel Kraus
