Connecting Two Charged Capacitors

[thelonious]

Homework Statement

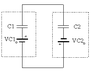
Two capacitors C1 & C2 are independently charged to potentials V1 & V2. Then the two capacitors are connected to each other, where each positive plate is connected to the other's negative plate. What is the final charge on capacitor 1 in terms of C1, C2, V1, V2?

Homework Equations

Q=CV
Capacitors in parallel have equal potential drops.
Qeq = C1 + C2 (parallel)

The Attempt at a Solution

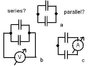
My instructor assigned this problem, worded as given. His given solution begins by stating that we can deduce from the problem statement that the capacitors are connected in parallel, and then solves by finding the equivalent capacitance, equivalent voltage, and using the expression resulting from the conservation of charge to solve for the final charge on capacitor 1. I follow the solution, but I do not understand why this problem statement describes capacitors in parallel. Isn't this a description of capacitors in series?

 

[ehild]

When only two capacitors are connected to each other they can be considered either in parallel or in series. Which to choose, depends on the situation.
When the capacitors are connected in series, the charge is the same on both. It is not the case now, as they were charged, and after connected, there is a net charge on the plates in contact. After connecting the plates, there is a charge transfer from one plate to the other till the potential becomes identical on both plates. This situation - equal potential difference and different charge on the connected capacitors - corresponds to parallel connection.


ehild

 

[thelonious]

I understand that capacitors in series have equal charge and capacitors in parallel have equal potential, but I do not understand how we know that charge will be transferred between the two until each has equal potential.
I see that initially, the charge on C1 is not equal to the charge on C2, and that charges will move. But why can't I say that charge will be transferred until each has equal charge and therefore a different potential, rather than equal potential and different charges?

 

[ehild]

You know that the charges on metals are free to move. They are driven by the electric field, and there is electric field between two places if those places are at different potential. In equilibrium, the potential is constant on a metal.
The capacitor plates are connected, so they are at equal potential in equilibrium, and the other connected pair is at an other potential, equal at both plates.

There is no reason why the charges would be equally distributed among the capacitors. ehild

 

[thelonious]

Ok thanks. Is it true that whenever two capacitors are connected to each other, and nothing else, that the two capacitors are in parallel and not series? Is it possible to connect two capacitors to each other (to each other only, and no other components) in series?

 

[ehild]

If two elements are connected in series or in parallel is defined with respect to other elements in the circuit. If they are connected only to each other and to nothing else, series and parallel have no meaning. As the connected plates of the pair of capacitors are at equal potential, so the potential difference is the same across both capacitors, you can take them connected in parallel.

ehild

 

[thelonious]

OK I see now. Each "half" of the circuit (plate + wire + plate combination) is a conductor, and therefore an equipotential surface. So the capacitors must be combined in parallel to find the equivalent capacitance.

 

[tiny-tim]

hi ehild!

If two elements … are connected only to each other and to nothing else, series and parallel have no meaning.

i think i disagree with this …

the capacitors clearly can be described as connected in series, but they can't be described together as a single capacitor, at least not one that obeys the usual rules …

usually, when we connect two capacitors in series, the charge on each is the same, and so the charge on each side of the-pair is the same, and so the-pair can be considered a single capacitor

but the-pair in this case has unequal charges on its two (outer) plates

we can apply the usual Q = CV rules to such a pair, provided that by Q we mean the difference between the charge on either plate and the equilibrium charge …

then the charges on the two (outer) plates are q₁ = Q + qC₁/(C₁ + C₂) and -q₂ = -Q + qC₂/(C₁ + C₂),

where q is the "trapped" charge shared by the two inner plates

and so V = V₁ + V₂ = q₁/C₁ + q₂/C₂ = Q/C₁ + Q/C₂

= Q/C, with C equal to the usual 1/(1/C₁ + 1/C₂) ​

 

[gneill]

One way to overcome the initial charge issue that will allow you to combine the capacitors into a single equivalent capacitance is to replace each initially charged capacitor with an equivalent circuit model consisting of an uncharged capacitor of the same value in series with a fixed voltage source with a value equal to the initial voltage on that capacitor. Thus:

Now you're free to combine the capacitors and voltage sources in this equivalent circuit to solve for the total charge that will be moved onto the equivalent capacitance. That total charge will also be equal to the individual charges that end up on the individual capacitors in the model. Stepping back once more, that will also be the change in the charges on the original capacitors. Take care with the polarities as dictated by the direction of the current that will flow due to the net voltage.

 

[ehild]

Hi tiny-tim,

i think i disagree with this …

And I am sorry, but I disagree with you.

the capacitors clearly can be described as connected in series, but they can't be described together as a single capacitor, at least not one that obeys the usual rules …

usually, when we connect two capacitors in series, the charge on each is the same, and so the charge on each side of the-pair is the same, and so the-pair can be considered a single capacitor

but the-pair in this case has unequal charges on its two (outer) plates

There are no outer plates, as both pairs of plates are connected: The positive plate of one capacitor to the negative plate of the other one, and the negative plate to the positive plate of the other capacitor. As the positive and negative charges have a metallic contact to move from one plate to the other, they will partially neutralise each other on both plates, till the voltage becomes equal on both capacitors.

I stick to my statement that the distinction between series and parallel has no sense if two circuit elements (two-poles) are connected only to each other.

Two elements are connected in series if they have a common pair of terminals and nothing else is connected to that junction. The free terminals are connected to the circuit.
Two elements are connected in parallel if both their terminals are common with the other element. The pairs of common terminals are connected to the circuit.

See drawing a. Two capacitors are connected to each other. They have a pair of terminals connected, and nothing else is connected to the junction, so the capacitors are connected in series.
But the other terminals are also connected, so they have two pairs of terminals in common, so they are parallel. So WHICH one?

If you add a third element, an ideal voltmeter or an ideal ammeter, which does not change anything, the situation becomes clear. b is parallel connection. C is series connection. And the charges and voltage of the individual capacitors are the same in both cases.

If Q1 was the charge on capacitor C1 and Q2 was the charge on C2, and Q1>Q2, after connecting the capacitors, the charges rearrange so the voltage is the same on both capacitors. The connected plates both will be either positively or negatively charged. There will be q1 charge on C1 and q2 charge on C2, and the common voltage is V=q1/C1=q2/C2. As the charge is conserved, Q1-Q2=q1+q2.

There are two equation for the unknown charges q1 and q2:

q1+q2=Q1-Q2
q1/C1=q2/C2.

It is easy to solve, and then V=(Q1-Q2)/(C1+C2) just like in case of parallel capacitors!.

ehild