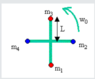
- #1
Eitan Levy
- 259
- 11
Homework Statement
4 masses attached by a cross with no mass are spinning on a smooth table around the center of the cross. The distance between any mass to the center is L. The angular velocity is ω0.
m1=m3,m2=m4
Suddenly, at t=0 (the time described in the picture), m4 disconnects from the cross.
Find the linear velocity of the cross after it disconnects.
Find the angular velocity of the three masses left around their center of mass.
Homework Equations
J=RxP
P=mv
The Attempt at a Solution
I am having a hard time figuring out what sizes will be conserved and why. Assuming the mass disconnects without any force involved, both the linear momentum and the angular momentum are supposed to be conserved in the system of the four masses.
On the other hand, when the cross will be spinning from this point, no external forces/torques will affect the three masses left, so they are supposed to be conserved there too.
I can't figure out how it's possible to get an answer, if all those sizes are conserved (I am not really sure they will but can't understand why not) I get stuck with equations with no solutions. How is it possible to get an answer if the momentum in the three mass system is conserved throughout its spin?
Thanks a lot.