- #1
Satvik Pandey
- 591
- 12
Homework Statement
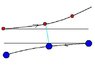
A proton and an α -particle are projected with velocity v=√[itex]\frac{e^{2}}{4∏εmL}[/itex] each from infinity as shown.The perpendicular distance between their initial velocities is L .Let the distance of their closest approach be D .
Given .Find (8D/L - 5)^2
Details and assumptions
1)mass of proton=m,charge=+e
2)mass of α-particle=4m,charge=2e
Homework Equations
The Attempt at a Solution
As the particles are located at infinite distance from each other so I think dist.L will not matter much.
I tried to use reduced mass problem here.
Here reduced mass(M) =4m/5 let it be at infinite distance from origin O.
What should be the initial velocity of reduced mass here? Is it 2v.(I used 2v here)
Lat it be at a distance D from O after some time.
At D all its kinetic energy wiil be converted to potential energy so
Mv^2/2=k2e^2/R
On putting values and after doing some calcilations I got
4D=5L
I got the value of 25.Am I right?
I think I am not.
This is the link of figure.
d3pq38zxuosm5i.cloudfront.net/solvable/2676130fa9.92b5dbd0d6.O7DvUt.png
Last edited: