- #1
ColtonCM
- 33
- 2
Member warned: Template use is required.
I've attached an image of part a of the question to this thread.
My question is this (the solution to these former homework problems are posted to help us study for exam, which is why know this already):
The angle between the two velocity vectors is determined to be pi/2. How? I know that dot product is abcos(Θ) where theta is the angle between the two velocity vectors. However, I don't know how to solve for theta when abcosΘ is not equal to anything?
I never had a trig class (which is really hurting me) so is there some unit circle technique that I'm supposed to know about to determine what the dot product is equal to so I can solve for theta? Something involving the x and y components of the velocity vectors?
Also, why can't I just use the magnitude of the velocity vector as the radius then solve using the regular T=2pi(r)/v equation? Velocity is always tangent to the trajectory, so shouldn't its magnitude always be the length of the radius?
Edit: Oops, sorry. Velocity vector 1 is (4.00)i + (3.00)j and velocity vector 2 is (-3.00)i + (4.00)j
Thanks,
Colton
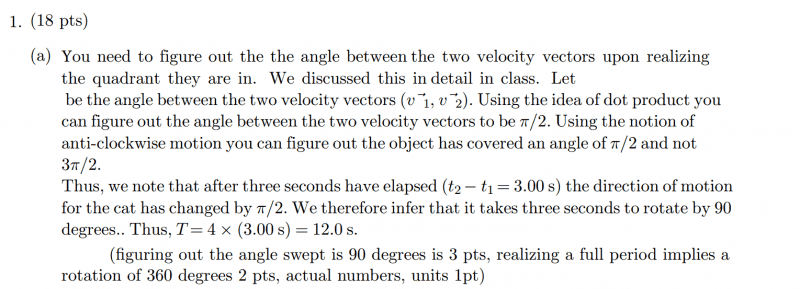
My question is this (the solution to these former homework problems are posted to help us study for exam, which is why know this already):
The angle between the two velocity vectors is determined to be pi/2. How? I know that dot product is abcos(Θ) where theta is the angle between the two velocity vectors. However, I don't know how to solve for theta when abcosΘ is not equal to anything?
I never had a trig class (which is really hurting me) so is there some unit circle technique that I'm supposed to know about to determine what the dot product is equal to so I can solve for theta? Something involving the x and y components of the velocity vectors?
Also, why can't I just use the magnitude of the velocity vector as the radius then solve using the regular T=2pi(r)/v equation? Velocity is always tangent to the trajectory, so shouldn't its magnitude always be the length of the radius?
Edit: Oops, sorry. Velocity vector 1 is (4.00)i + (3.00)j and velocity vector 2 is (-3.00)i + (4.00)j
Thanks,
Colton
Last edited: