Field Extensions - Lovett, Theorem 7.1.10 - Another question

[Math Amateur]

I am reading "Abstract Algebra: Structures and Applications" by Stephen Lovett ...

I am currently focused on Chapter 7: Field Extensions ... ...

I need help with another aspect of the proof of Theorem 7.1.10 ...Theorem 7.1.10, and its proof, reads as follows:

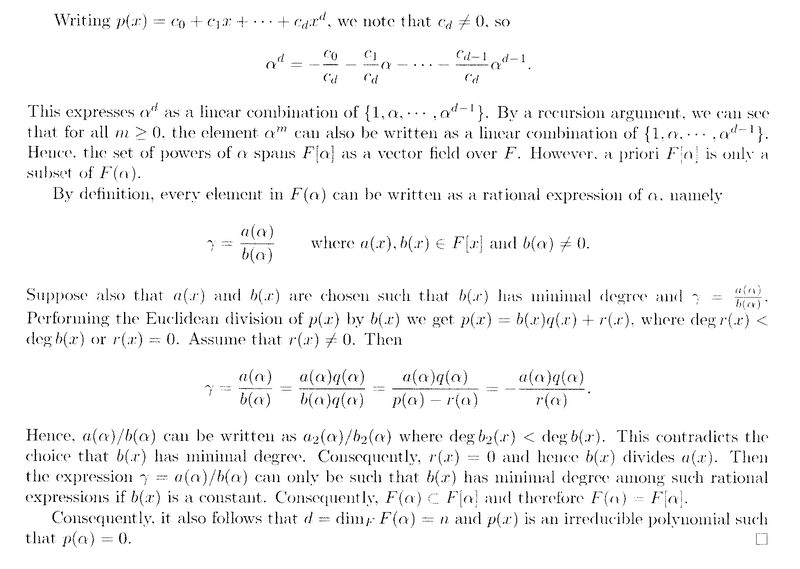
In the proof of the above Theorem, towards the end of the proof, Lovett concerns himself with proving that $F( \alpha ) \subset F[ \alpha ]$ ... ...

To do this he points out that every element in $F( \alpha )$ can be written as a rational expression of $\alpha$, namely ...

$\gamma = \frac{ a( \alpha )}{ b( \alpha )}$

where $a( \alpha ), b( \alpha ) \in F[x]$ and $b( \alpha ) \neq 0$ ...

Lovett then says ...

" ... ... Suppose also that $a( \alpha )$ and $b( \alpha )$ are chosen such that $b( \alpha )$ has minimal degree and $\gamma = \frac{ a( \alpha )}{ b( \alpha )}$. ... ... "What does Lovett mean by choosing $a( \alpha )$ and $b( \alpha )$ such that $b( \alpha )$ has minimal degree ... ... ?

It cannot mean choosing special elements for $b( \alpha )$ ... as then $\gamma$ would not be a representative element of $F( \alpha )$ ...

Can someone please clarify this issue ...

Peter

EDIT Does it just mean that when we have ... for example ...$\gamma = \frac{ a( \alpha )}{ b( \alpha )} = \frac{ x^3 - 3x }{ x^4 + 7x }$we just (in this case, for example) we just 'cancel' the $x$ ... and similarly for other examples ...

 

[fresh_42]

Yes.

The representation of a number $\gamma$ as a quotient $\frac{a(\alpha)}{b(\alpha)}$ is usually not unique.
However, the set $\mathcal{Q}_\gamma = \left\{ \left. \frac{a(x)}{b(x)} \,\right\vert \,a,b \in F[x]\, , \,\gamma = \frac{a(\alpha)}{b(\alpha)} \right\}$ can be partially ordered by the degree of $b(x)$. Furthermore, $\deg b(x) \geq 0$, and thus there must be a minimal element in any ordered chain in $\mathcal{Q}_\gamma$. Such an element is chosen.

This doesn't mean this element is unique, as we still can have common factors of nominator and denominator of degree $0$, i.e. elements of $F$, or even different polynomials $b(x)$ and $b'(x)$ in the representation of $\gamma$. But as the argument only relies on the degree of $b(x)$, we don't care, whether elements of $F$ have been canceled or not, or which $b(x)$ of minimal degree (i.e. which ordered chain in $\mathbb{Q}_\gamma$\,) we chose. Even $\alpha$ itself can be in $F$, in which case nothing would have been to prove, so we can rule out this case, too, if we like.

 

[Math Amateur]

Yes.

The representation of a number $\gamma$ as a quotient $\frac{a(\alpha)}{b(\alpha)}$ is usually not unique.
However, the set $\mathcal{Q}_\gamma = \left\{ \left. \frac{a(x)}{b(x)} \,\right\vert \,a,b \in F[x]\, , \,\gamma = \frac{a(\alpha)}{b(\alpha)} \right\}$ can be partially ordered by the degree of $b(x)$. Furthermore, $\deg b(x) \geq 0$, and thus there must be a minimal element in any ordered chain in $\mathcal{Q}_\gamma$. Such an element is chosen.

This doesn't mean this element is unique, as we still can have common factors of nominator and denominator of degree $0$, i.e. elements of $F$, or even different polynomials $b(x)$ and $b'(x)$ in the representation of $\gamma$. But as the argument only relies on the degree of $b(x)$, we don't care, whether elements of $F$ have been canceled or not, or which $b(x)$ of minimal degree (i.e. which ordered chain in $\mathbb{Q}_\gamma$\,) we chose. Even $\alpha$ itself can be in $F$, in which case nothing would have been to prove, so we can rule out this case, too, if we like.

Thanks fresh_42 ... most helpful ...

BUT ... just a couple more clarifications pertaining to Lovett's argument that $b(x)$ must be a constant (an argument I am having trouble fully understanding ... )

(QUESTION 1) Lovett brings the minimal polynomial $p(x)$ into the argument ... why is he doing this ... what is his objective in this matter ... ?

Further ... ... Lovett writes ... :

" ... ... Hence $a( \alpha ) / b( \alpha )$ can be written as $a_2( \alpha ) / b_2( \alpha )$ where $\text{ deg } b_2( x ) \lt \text{ deg } b( x)$. This contradicts the choice that $b( x)$ has minimal degree. Consequently, $r(x) = 0$ and hence $b(x)$ divides $a(x)$. ... .. "

(QUESTION 2) I cannot see how in this argument Lovett concludes that $b(x)$ divides $a(x)$ ... ... ? Can you help ...?Then Lovett writes:

" ... ... Then the expression $\gamma = a( \alpha ) / b( \alpha )$ can only be such that $b(x)$ has minimal degree among such rational expressions if $b(x)$ is a constant. ... ... "

(QUESTION 3) I do not follow this argument that $b(x)$ must be a constant ... can you help ... ?

Peter

 

[fresh_42]

Hi Peter,

(QUESTION 1) Lovett brings the minimal polynomial $p(x)$ into the argument ... why is he doing this ... what is his objective in this matter ... ?

About the "why" question: (I'll try not to write "Because it works." and guess a motivation instead. Truth is, finding a proof is usually a process of trial and error. You still can see this in Lovett's style, which mostly is a sequence of many small contradiction arguments. Not very pleasant to read.)

If we gather all we have about $\gamma$ and $\alpha$, then it is $\gamma = \frac{a(\alpha)}{b(\alpha)}$ and $p(\alpha)=0$, so it makes sense to relate those three polynomials ( $a,b,p$ ), i.e. see what divisions bring. The goal is to show $b(x)=b_0 \in F$ so that all quotients $\gamma$ are already in $F[\alpha]$. This means the degree of $b(x)$ has to be $1$ for this purpose. Division is a good method in algebra to reduce degrees or similar valuations or to use it in an induction argument.

Further ... ... Lovett writes ... :

" ... ... Hence $a( \alpha ) / b( \alpha )$ can be written as $a_2( \alpha ) / b_2( \alpha )$ where $\text{ deg } b_2( x ) \lt \text{ deg } b( x)$. This contradicts the choice that $b( x)$ has minimal degree. Consequently, $r(x) = 0$ and hence $b(x)$ divides $a(x)$. ... .. "

$a_2(\alpha) = a(\alpha)\cdot q(\alpha)$ and $b_2(\alpha)=-r(\alpha)$. We also have (Euclid's algorithm) $\deg b_2(x) = \deg r(x) < \deg b(x)$. Minimality of $\deg b(x)$ in the representation of $\gamma$, however, implies that $b_2(x)=r(x)=0$. (We have chosen $b(x)$ this way, see my earlier post.)

Now I'm also lost. I assume it's a typo, since $r(x)=0$ implies $b(x) \mid p(x)$. Now $p(x)$ is irreducible, and therefore a factor $b(x)$ of it has to be a unit, i.e. an element of $F\, : \, b(x)=b_0 \in F$.

(QUESTION 2) I cannot see how in this argument Lovett concludes that $b(x)$ divides $a(x)$ ... ... ? Can you help ...?

Me neither, see above. $a(x)$ should be replaced by $p(x)$ here.

Then Lovett writes:

" ... ... Then the expression $\gamma = a( \alpha ) / b( \alpha )$ can only be such that $b(x)$ has minimal degree $b(x)$ has minimal degree among such rational expressions if $b(x)$ is a constant. ... ... "

(QUESTION 3) I do not follow this argument that $b(x)$ must be a constant ... can you help ... ?

We have written $\gamma = \frac{a_2(\alpha)}{b_2(\alpha)}$ with the settings for $a_2(x),b_2(x)$ I mentioned above. But $\deg b(x)$ was chosen minimal among all possible representations of $\gamma$ in this way.

I hope my answer on your first question covers the other two.

I've forgotten one point. The argument that $b(x)$ has to be a unit uses the fact, that otherwise $q(x)=q_0$ would be the unit (by irreducibility of $p(x)$). But then $0=p(\alpha)=b(\alpha)\cdot q(\alpha) = b(\alpha) \cdot q_0$ and $b(\alpha)=0$ which can't be by the choice of $b(\alpha)$ as the denominator of $\gamma$. Thus $b(x)$ has to be the unit.

 

[Math Amateur]

Hi Peter,

About the "why" question: (I'll try not to write "Because it works." and guess a motivation instead. Truth is, finding a proof is usually a process of trial and error. You still can see this in Lovett's style, which mostly is a sequence of many small contradiction arguments. Not very pleasant to read.)

If we gather all we have about $\gamma$ and $\alpha$, then it is $\gamma = \frac{a(\alpha)}{b(\alpha)}$ and $p(\alpha)=0$, so it makes sense to relate those three polynomials ( $a,b,p$ ), i.e. see what divisions bring. The goal is to show $b(x)=b_0 \in F$ so that all quotients $\gamma$ are already in $F[\alpha]$. This means the degree of $b(x)$ has to be $1$ for this purpose. Division is a good method in algebra to reduce degrees or similar valuations or to use it in an induction argument.

$a_2(\alpha) = a(\alpha)\cdot q(\alpha)$ and $b_2(\alpha)=-r(\alpha)$. We also have (Euclid's algorithm) $\deg b_2(x) = \deg r(x) < \deg b(x)$. Minimality of $\deg b(x)$ in the representation of $\gamma$, however, implies that $b_2(x)=r(x)=0$. (We have chosen $b(x)$ this way, see my earlier post.)

Now I'm also lost. I assume it's a typo, since $r(x)=0$ implies $b(x) \mid p(x)$. Now $p(x)$ is irreducible, and therefore a factor $b(x)$ of it has to be a unit, i.e. an element of $F\, : \, b(x)=b_0 \in F$.Me neither, see above. $a(x)$ should be replaced by $p(x)$ here.

We have written $\gamma = \frac{a_2(\alpha)}{b_2(\alpha)}$ with the settings for $a_2(x),b_2(x)$ I mentioned above. But $\deg b(x)$ was chosen minimal among all possible representations of $\gamma$ in this way.

I hope my answer on your first question covers the other two.

I've forgotten one point. The argument that $b(x)$ has to be a unit uses the fact, that otherwise $q(x)=q_0$ would be the unit (by irreducibility of $p(x)$). But then $0=p(\alpha)=b(\alpha)\cdot q(\alpha) = b(\alpha) \cdot q_0$ and $b(\alpha)=0$ which can't be by the choice of $b(\alpha)$ as the denominator of $\gamma$. Thus $b(x)$ has to be the unit.

Thanks fresh_42 ... just reflecting on your post ...

But one quick clarification ...

You write:

" ... ... The goal is to show $b(x)=b_0 \in F$ so that all quotients $\gamma$ are already in $F[\alpha]$. This means the degree of $b(x)$ has to be $1$ for this purpose. ... ... "Can you explain your reasoning that the degree of $b(x)$ has to be $1$ ... ...

If $b(x)=b_0 \in F$ then doesn't the degree of $b(x)$ have to be $0$ ... ... ?Hope you can clarify ... ... must be misunderstanding you somehow ...

Peter

 

[fresh_42]

Yep, it's late up here ... Of course $0$, sorry.

 

[Math Amateur]

Yep, it's late up here ... Of course $0$, sorry.

No problems ... your posts have been extremely helpful!

Thanks again ... from the edge of the world in southern Tasmania ... early afternoon here ...

Peter