Find maximum velocity in Acceleration-Time Graph

[Govind_Balaji]

Homework Statement

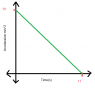
Find the maximum velocity of the particle using the graph given.(See attachment)

Homework Equations

Initial velocity was not given. I assumed it to be 0.

In book, the solution was 55m/s^2

The Attempt at a Solution

Initial acceleration was $10m/s^2$. Final acceleration after 11 seconds was $0m/s^2$.

I figured out that $a_n=\frac{110-10(n-1)}{11}$, where n is the second and $a_n$ is the acceleration in $n$th second.

I hope I am right at this step.

I think that velocity will be maximum at 11th second.

So I calculate the velocity at 1st second by
$\begin{align*}v_n&=v_{n-1}+\frac{a_n}{2}\\ &=v_{n-1}+\frac{55-5(n-1)}{11}\\\end{align*}$.

I derived this using identities of motion and my already derived $a_n$ formula.

$v_0=0\\ v_1=0+\frac{55-5(1-1)}{11}=5\\ v_2=5+\frac{55-5(2-1)}{11}=\frac{105}{11}\\ ...\\ v_{11}=295/11\\$

I should get 55m/s.

Please help me out.

 

[CAF123]

I figured out that $a_n=\frac{110-10(n-1)}{11}$, where n is the second and $a_n$ is the acceleration in $n$th second.

Does this equation recover the values of a at n=0 and n=11 that you know from looking at the graph?

 

[dauto]

No, you can't do that. Don't use n. Use t and find an expression for the acceleration as a function of time a(t) where t is any number - not just a whole number. Than integrate the acceleration to find the velocity. If you don't know integrals than you probably know some theorem relating the change in velocity to the area in a graph of acceleration vs time. In this case the area is a triangle which can be solved without integrals.

 

[Govind_Balaji]

No, you can't do that. Don't use n. Use t and find an expression for the acceleration as a function of time a(t) where t is any number - not just a whole number. Than integrate the acceleration to find the velocity. If you don't know integrals than you probably know some theorem relating the change in velocity to the area in a graph of acceleration vs time. In this case the area is a triangle which can be solved without integrals.

Does this equation recover the values of a at n=0 and n=11 that you know from looking at the graph?

Wait a minute!

I got a brilliant idea.

I know that the area below velocity-time graph gives the total displacement of the body.

I thought about it a while.

Velocity is the change of displacement over time.

Acceleration is the change of velocity over time.

Then , presto! Instead of velocity in velocity-time graph, we have acceleration.

Instead of distance(factor of velocity), we have velocity(factor of acceleration).

Hence the area under the graph is the max. velocity i.e. 55m/s.

After solving it, I came to this thread and also saw dauto saying the same.

Thank you CAF123.

 

[dauto]

Wait a minute!

I got a brilliant idea.

I know that the area below velocity-time graph gives the total displacement of the body.

I thought about it a while.

Velocity is the change of displacement over time.

Acceleration is the change of velocity over time.

Then , presto! Instead of velocity in velocity-time graph, we have acceleration.

Instead of distance(factor of velocity), we have velocity(factor of acceleration).

Hence the area under the graph is the max. velocity i.e. 55m/s.

After solving it, I came to this thread and also saw dauto saying the same.

Thank you CAF123.

Excellent.