- #1
chwala
Gold Member
- 2,650
- 351
- Homework Statement
- This is from an A level past paper question;
i. A plate of cake holds ##12## different cakes. Find the number of ways these cakes can be shared between Alex and James if each receives an odd number of cakes.
- Relevant Equations
- Stats
I went through the question; find the mark scheme here;
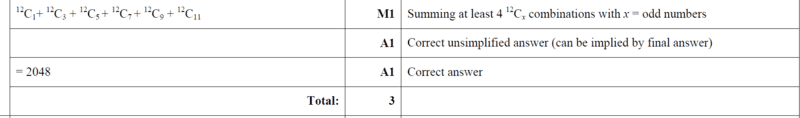
Well i can follow the mark scheme steps but i need some clarity or rather insight. The ##12## cakes are being shared to the two persons. In my understanding the odd numbers are;
##[1,3,5,7,9,11]## Now this means that they may each get ##1## cake in ##1 ×11C_1## ways or alternatively ##^{12}C_1×2##persons...
...are they not supposed to have ##2048 ×2##?
Supposing it was ##13## people instead of ##2## ...Would the steps still be the same as shown on the markscheme? A bit confusing...
your insight appreciated...
Well i can follow the mark scheme steps but i need some clarity or rather insight. The ##12## cakes are being shared to the two persons. In my understanding the odd numbers are;
##[1,3,5,7,9,11]## Now this means that they may each get ##1## cake in ##1 ×11C_1## ways or alternatively ##^{12}C_1×2##persons...
...are they not supposed to have ##2048 ×2##?
Supposing it was ##13## people instead of ##2## ...Would the steps still be the same as shown on the markscheme? A bit confusing...
your insight appreciated...
Last edited: