opus
Gold Member
- 717
- 131
Homework Statement
Find the exact value of ##y=cos\left(arcsin \frac {5} {11} \right)##
Homework Equations
The Attempt at a Solution
I'm stuck at square one. I know that I must evaluate arcsin first, which will give me an angle θ. From that angle, I can take the cosine of it and that much makes sense.
However, in this "guided homework problem" it is stating information that doesn't seem so obvious to me. I've posted a picture.
In the guided problem, it states that θ is in the range of the inverse function. I don't see how this is the case. The range of the inverse function is in the inclusive range of ##\frac {-π}{2}## and ##\frac {π}{2}##. In looking at the problem, I can tell that sine is positive, but that doesn't imply that it's in range as it could be in quadrant II.
What should I be looking for?
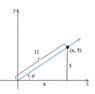
 I think I've got a much better understanding of them now. What I didn't fully grasp was that we take the sine of angles and arcsin of values which made things quite confusing. In thinking about the domain to keep the function one-to-one, I thought about a similar idea in Algebra but with quadratic graphs and how we need to restrict the domain to make them one-to-one.
I think I've got a much better understanding of them now. What I didn't fully grasp was that we take the sine of angles and arcsin of values which made things quite confusing. In thinking about the domain to keep the function one-to-one, I thought about a similar idea in Algebra but with quadratic graphs and how we need to restrict the domain to make them one-to-one.