- #1
Satonam
- 38
- 1
The bracket shown in figure 1 is applied to both ends of a beam (figure 2) which experiences a single static load at its center. The bottom of both brackets are fixed by some method, so it can't deflect. According to Solidworks, the bracket survives with flying colors, but it bothers me that I can't seem to get similar results with a simple hand calculation. I thought about treating the brackets like a wall and solving for a beam with fixed ends, but when I apply it to a physical object, I get stumped. In college, we're always concerned about the deflection of a beam and the flexural stresses, but we never consider all the different ways that a bracket can be employed and the different types of supports that it represents. How would you solve a problem like this?
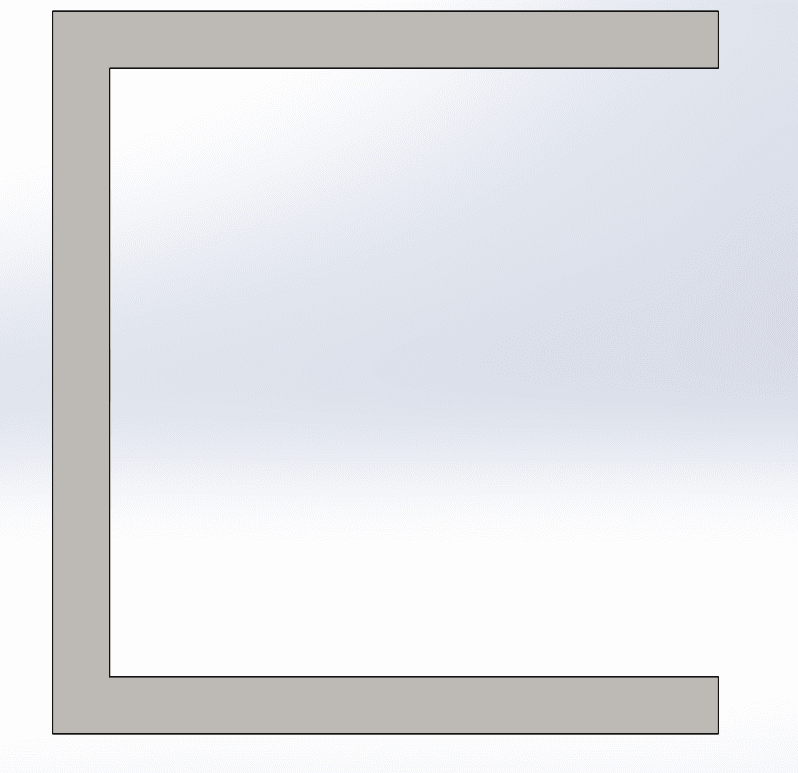
Fig. 1 Bracket

Fig. 2
For clarity, here's the set-up:
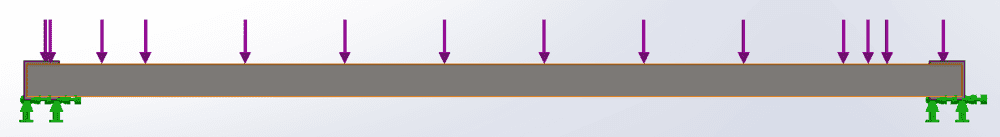
Fig. 1 Bracket
Fig. 2
For clarity, here's the set-up: