- #1
MrMuscle
- 12
- 1
- TL;DR Summary
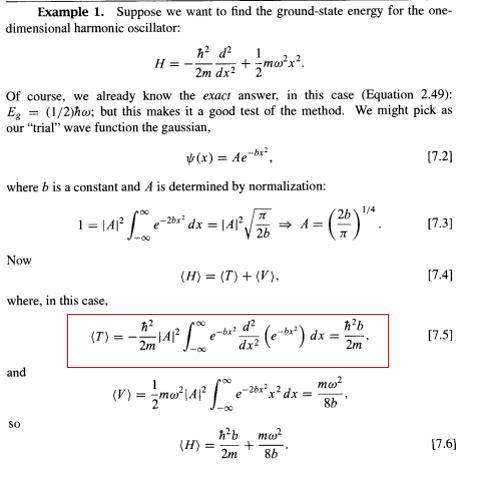
- Trying to solve the integral, for variational principle in griffith's book.
But I'm stuck at the final step. Can you please help?
Hi, I am trying to solve the problem in Griffith's book about variational principle. However, I am having trouble to solve the integral by myself that I have indicated in redbox in Griffith's book. You can see my effort in hand-written pages. I brought it to the final step I believe, but can't go further. A little bit help to finish the integration would do great! Thanks for your help in advance!