Magnetic flux of magnetic field changing as a function of time

[besebenomo]

Homework Statement

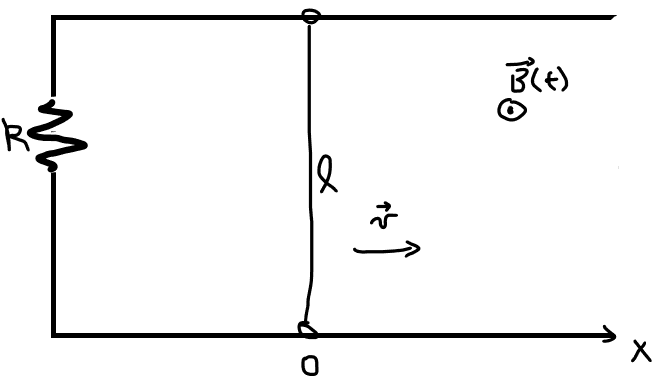
There is a circuit with resistance R, a moving bar of lenght l moves at constant velocity v=l/T and at t=0 the bar is in position x=0. In the beginning the circuit is in a region where B=0, the the bar starts moving into a region with a non-zero magnetic field (see figure), which varies with time.

What is the current flowing in the circuit?

Relevant Equations

Magnetic flux, Faraday's law

$$B(t) = B_{0} \frac{t^2}{T^2}$$

for $0 \leq t \leq T$

The issue here is more conceptual, because once I find the flux of B I know how to proceed to find the current. I got velocity (but it seems to me that it is the initial velocity), I could use it to find the time in function of space, but not sure if that is correct...

Instead I tried this way:
$$\Phi(B) = B_{0}\frac{t^2}{T^2} x(t)l$$
Should I integrate instead? And change variable?

 

[malawi_glenn]

https://www.physicsforums.com/help/latexhelp/

 

[besebenomo]

E

https://www.physicsforums.com/help/latexhelp/

thank you

 

[kuruman]

The issue here is more conceptual, because once I find the flux of B I know how to proceed to find the current. I got velocity (but it seems to me that it is the initial velocity), I could use it to find the time in function of space, but not sure if that is correct...

Instead I tried this way:
$$\Phi(B) = B_{0}\frac{t^2}{T^2} x(t)l$$
Should I integrate instead? And change variable?

You have the flux and it is what you say. Since you say you know how to proceed to find the current, do it and show your work. One piece of information you need to bring into the picture is that the bar is moving at constant velocity. What does that mean? Hint: You need to write Newton's second law just like in the other thread.