- #1
Math Amateur
Gold Member
MHB
- 3,990
- 48
I am reading Martin Crossley's book, Essential Topology.
I am at present studying Example 5.55 regarding the Mobius Band as a quotient topology.
Example 5.55 Is related to Examples 5.53 and 5.54. So I now present these Examples as follows:
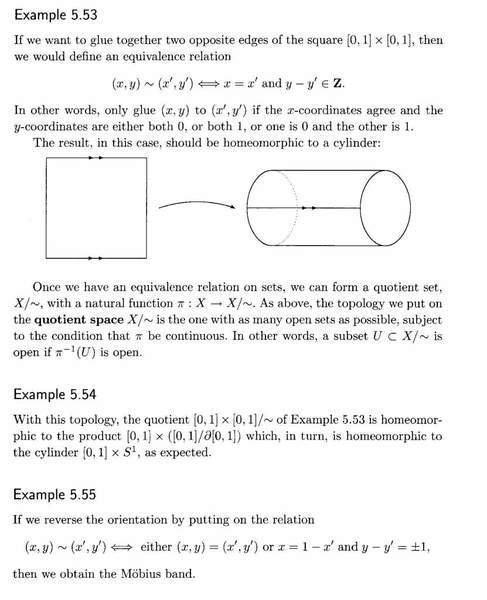 I cannot follow the relation [itex] (x,y) \sim (x', y') \Longleftrightarrow \text{ either } (x,y) = (x', y') \text{ or } x = 1 - x' \text{ and } y - y' = \pm 1 [/itex]Why do we need [itex](x,y) = (x', y') [/itex] in the relation? Indeed, why do we need [itex] y - y' = \pm 1 [/itex]?Surely all we need is [itex] (x,y) \sim (x', y') \Longleftrightarrow x = 1 - x' \text{ and } y - y' = -1 [/itex]Can anyone explain how the relation [itex] (x,y) \sim (x', y') \Longleftrightarrow \text{ either } (x,y) = (x', y') \text{ or } x = 1 - x' \text{ and } y - y' = \pm 1 [/itex] actually works to produce the Mobius Band?Peter
I cannot follow the relation [itex] (x,y) \sim (x', y') \Longleftrightarrow \text{ either } (x,y) = (x', y') \text{ or } x = 1 - x' \text{ and } y - y' = \pm 1 [/itex]Why do we need [itex](x,y) = (x', y') [/itex] in the relation? Indeed, why do we need [itex] y - y' = \pm 1 [/itex]?Surely all we need is [itex] (x,y) \sim (x', y') \Longleftrightarrow x = 1 - x' \text{ and } y - y' = -1 [/itex]Can anyone explain how the relation [itex] (x,y) \sim (x', y') \Longleftrightarrow \text{ either } (x,y) = (x', y') \text{ or } x = 1 - x' \text{ and } y - y' = \pm 1 [/itex] actually works to produce the Mobius Band?Peter
I am at present studying Example 5.55 regarding the Mobius Band as a quotient topology.
Example 5.55 Is related to Examples 5.53 and 5.54. So I now present these Examples as follows:
Attachments
Last edited:
