- #1
Rafimah
- 12
- 1
Homework Statement
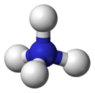
The ammonium ion NH4+ has the shape of a regular tetrahedron. The Nitrogen
atom (blue sphere) is at the center of the tetrahedron and the 4 Hydrogen atoms
are located at the vertices at equal distances L from the center (about 1 Å). Denote
the mass of the hydrogen atoms by Mh and that of the nitrogen atom by Mn.
a. What is the moment of inertia I0 of NH4+ for rotation along any of the 4
axes that passes through the central nitrogen atom and one of the 4
hydrogen atoms? Express your answer in terms of Mh, Mn and L. (Hint:
The central angle between the lines to any two vertices of a perfect
tetrahedron is acos(-1/3) or approximately 110 deg.)
b. Derive an expression for the moment of inertia tensor I for the ammonium
ion. Can you show that I0 is one of the principal moments of I and that in
fact all principal moments must equal I0 (The ammonium is a spherical
top)?
Homework Equations
To solve part a, I simply use $$ \sum{m_h r^2} $$ . I reasoned that for part b, I should do the same about two axes perpendicular to one running through one of the hydrogen atoms and the central atoms. I figured these two moments of inertia should be degenerate based on the symmetry and I solved, getting $$m_h L^2 (1+3 sin(20)^2) $$. However, I don't think this can be simplified to be equivalent to my answer for part a, $$ 3 m_h L^2 cos(70)^2 $$. Am I doing something wrong here? Also, do I need to show that the off diagonal elements are zero in this tensor or can I assume that if I find the three moments of inertia are equivalent? Also, I assumed that the origin here would be the coordinate of the central atom, is that acceptable?
The Attempt at a Solution
See above
Attachments
Last edited: