- #1
cwill53
- 220
- 40
Apologies if I make anyone frustrated.
To start, I've only had up to Calculus II so far but I was curious how to use and evaluate integrals used for moment of inertia. I know that the moment of inertia is basically an object's resistance to rotation, and is the rotational analog of mass. I know that it can be expressed for both uniform and non-uniform objects by, assuming you can divide the object into point masses, the summation of point masses multiplied by each of their respective distances from the axis of rotation, or for a continuous mass by integration, which is the part I'm shaky on. The moment of inertia I can be defined as:
$$\sum_{i}^{}m_{i}r_{i}=\int \int \int r^2dm$$where I is the moment of inertia and r is the distance of each point from the axis of rotation. I don't really understand how I would use the above integral and WHY there are three integrals in the first place, as the following expression can also be used to calculate moment of inertia:
$$I=\int r^2ρdV$$where ρρ is density and V is volume. m is mass.
If m=dρdVm=dρdV and dmdm can be expressed as ##ρdV## why would I integrate three times in the above integral?
I can give you an example of what confused me. I was reading through my physics textbook, working out problems, and every single moment of inertia problem involving calculus I simply couldn't wrap my head around.
For example, I viewed the solution online to the following problem:
A sphere with radius R= 0.200 m has density ρρ that decreases with distance r from the center of the sphere according to ρ=3.00×103kg/m3−(9.00×103kg/m4)rρ=3.00×103kg/m3−(9.00×103kg/m4)rρ=3.00×103kg/m3−(9.00×103kg/m4)rρ=3.00×103kg/m3−(9.00×103kg/m4)r (a) Calculate the total mass of the sphere. (b) Calculate the moment of inertia of the sphere for an axis along a diameter.
The solver solved the problem this way:
As you can probably tell I had no idea what was going on here. I still copied the solution down into my notebook for future reference.
Another thing I've seen attached to moment of inertia is the word tensor, and the moment of inertia tensor. As far as I know a tensor, according to a books that I have, is a linear operator that maps a vector to something else, a first order tensor mapping a vector to a scalar, a second order mapping a vector to a vector, and a third order tensor mapping a vector to whatever that is that comes after vectors.
Another book gives a different kind of introduction:
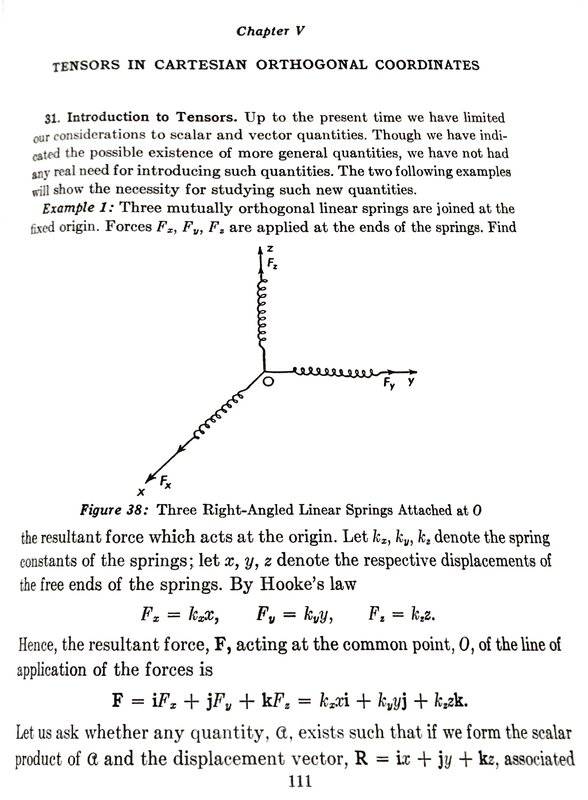
Which is admittedly confusing.
To start, I've only had up to Calculus II so far but I was curious how to use and evaluate integrals used for moment of inertia. I know that the moment of inertia is basically an object's resistance to rotation, and is the rotational analog of mass. I know that it can be expressed for both uniform and non-uniform objects by, assuming you can divide the object into point masses, the summation of point masses multiplied by each of their respective distances from the axis of rotation, or for a continuous mass by integration, which is the part I'm shaky on. The moment of inertia I can be defined as:
$$\sum_{i}^{}m_{i}r_{i}=\int \int \int r^2dm$$
$$I=\int r^2ρdV$$
If m=dρdVm=dρdV and dmdm can be expressed as ##ρdV## why would I integrate three times in the above integral?
I can give you an example of what confused me. I was reading through my physics textbook, working out problems, and every single moment of inertia problem involving calculus I simply couldn't wrap my head around.
For example, I viewed the solution online to the following problem:
A sphere with radius R= 0.200 m has density ρρ that decreases with distance r from the center of the sphere according to ρ=3.00×103kg/m3−(9.00×103kg/m4)rρ=3.00×103kg/m3−(9.00×103kg/m4)rρ=3.00×103kg/m3−(9.00×103kg/m4)rρ=3.00×103kg/m3−(9.00×103kg/m4)r (a) Calculate the total mass of the sphere. (b) Calculate the moment of inertia of the sphere for an axis along a diameter.
The solver solved the problem this way:
$$\int \int \int \rho (r)dV=\int dm\rightarrow \int_{0}^{2\pi }\int_{0}^{\pi }\int_{0}^{R}(a-br)r^2sin\phi .d\theta .d\phi .dr$$As you can probably tell I had no idea what was going on here. I still copied the solution down into my notebook for future reference.
Another thing I've seen attached to moment of inertia is the word tensor, and the moment of inertia tensor. As far as I know a tensor, according to a books that I have, is a linear operator that maps a vector to something else, a first order tensor mapping a vector to a scalar, a second order mapping a vector to a vector, and a third order tensor mapping a vector to whatever that is that comes after vectors.
Another book gives a different kind of introduction:
Which is admittedly confusing.
Last edited: