Pivoting Rod (How to find angular acceleration)

[physics817]

Homework Statement

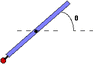
A uniform rod is pivoted at its center and a small weight of mass M = 5.07 kg is rigidly attached to one end. You may neglect the size of the weight and assume it is located right at the end of the rod.

a) What vertical force, Fv, must be applied to the opposite end to keep the rod at an angle of q = 37° to the horizontal?

Answer to part a) I calculated the answer to part a and came up with 49.74 N

b) The vertical force is now replaced by a horizontal force, Fh. How large must this be to hold the rod at the q = 37° angle?

Answer: 66.0 N

c) Now suppose that the rod has length L = 6.7 m and mass mrod = 10.9 kg. Suppose also that there are no external forces applied (i.e. Fh = Fv = 0). The system is released from rest at the q = 37° angle. What is the angular acceleration just after it is released (in rad/s^2)?

This part I do not know how to answer. I attemped it using the equation

sum of torques= (moment of inertia I)* (angular acceleration)

Homework Equations

The Attempt at a Solution

Sum of torques= I* (angular acceleration)

The moment of inertia of a rod is (1/12) ML^2, where the mass of the rod is 10.9 kg. And L is 6.7 m. [ (1/12)(10.9kg)(6.7m)^2 = 40.775 kh*m^2.]
I added this to the moment of inertia of the weight which is mr^2 [5.07m* (6.7/2)^2 = 56.9]

I combined the two to get the moment of inertia(total) = 97.673

then for torque= rF, I used F= mg= (10.9+5.070(9.81)), which came out as 156.67. I multiplied that number by r= 3.35, and came out with 524.83.

so to find angular acceleration: 524.83/97.67= 5.37. However, this turned out wrong.

My way of calculating the moment of inertia might be wrong? Somebody please help me.

Part d) What is the angular velocity when the rod is vertical?

It says to use conservation of energy but I'm not sure where to start. Thank you and help will be much appreciated.

 

[Dr.D]

You have an error in calculating the moment due to the unbalanced weight. You have calculated it as though the bar were horizontal, but in fact it is at an angle. Go back and look at this part again.

As to the last part, calculate the potential and kinetic energies when the bar starts, and then again in the position of interest. With no friction, they must be the same, so this gives you an equation that can be solved for the angular velocity in the second position.

 

[physics817]

How do you find the moment of inertia of the rod at an angle?

 

[physics817]

Oh, and did I calculate the torque correctly?

 

[Dr.D]

There was no problem with your calculation of the mass moment of inertia. The problem was in your calculation of the moment (torque); that's where your error is.

 

[physics817]

I think I have a hard time deciding what force to use. If it's at an angle, is it right to use the force in the y direction (due to gravity) and add that to the force in the y direction (or y component of force) of the weight and combine them?


For part d,
KE= 0.5I(omega squared)= change in PE(of rod and weight)
mgh=KE
(6.7)*(9.81)*(10.9+5.07)=0.5I(omega squared)
I from part C is 97.673. Solve for omega and I ended up with 4.636 but that is wrong.

 

[Doc Al]

then for torque= rF, I used F= mg= (10.9+5.070(9.81)), which came out as 156.67.

Don't just add the weight of rod and mass together and treat them as a single object. The weight of each acts at a different location and thus produces a different torque:
- Where does the weight of the rod act? What torque does it produce?
- Where does the weight of the mass act? What torque does it produce?

I multiplied that number by r= 3.35

Don't forget to consider the angle when you calculate torque.

 

[Dr.D]

Do you know the definition of torque as
T = r x F
where r is the vector from the reference point to the location of the force
F is the force vector
and x denotes a cross product?
This might help you account for the angle, and it willl also help you correctly calculate the torque from the two diffferent weights as pointed out by Doc Al.

 

[physics817]

- Where does the weight of the rod act? What torque does it produce?

The weight of the rod acts at its center of mass so at the pivot point? It produces 0 torque because it's 0m away from the axis of rotation?

- Where does the weight of the mass act? What torque does it produce?

The weight of the mass acts downward? And the T= rsin(theta)F= (half the length of the rod)*(sin (37)) * the (Weight of the mass= (9.81)(5.07)= 49.737))
total= (6.7/2)sin(37)*(49.737)= 100.273

 

[Dr.D]

You are right about the torque due to the weight of the rod.

You don't have the torque due to the mass correct yet. Do you know how the cross product works? You need the sine of the angle swept as you move from the first vector to the second vector. In this case, that is not 37 deg. Try again.

 

[physics817]

Oh! It should be 37 + 90 degrees.

So you would end up with 133.07 instead of 100.273. Thank you!

Can you help me with part d of the question? I know you need to use conservation of energy.
KE= 0.5I(omega squared)= change in PE(of rod and weight)

Do I use the angle to calculate the distance the rod has traveled and the distance the ball has traveled to become vertical?

 

[Dr.D]

Rotating r into w (the weight of the small mass, m), the angle turned is 53 deg. The vector r was down at 37 deg below the horizontal, and w is straight down.

For d) you will need the angle the rod turns, and you will need to consider how much elevation change there is for the CM.

 

[physics817]

So for part d, it's change in potential energy = just rotational energy then because the system isn't moving translationally?

change in mgh= 1/2 I (omega)^2

h= sin (90-37)* (L/2)= 1.333, and since we know I from part c, we can solve for omega. angular velocity then equals 1.1655 rad/s?


Thank you so much for all your help!

 

[Delphi51]

Don't we have to find the center of mass? It will rotate about the center of mass, and the moment of inertia and torque should be calculated with all distances measured from the c of m.

I get the cm 0.7 meters in from the 5.07 kg mass. Moment of inertia 79. Angular acceleration 2.51 CLOCKWISE. But I'm prone to mistakes.

In (d) it seems to me the angle is 37 + 90 degrees because I've got it rotating clockwise.

 

[Doc Al]

Don't we have to find the center of mass? It will rotate about the center of mass, and the moment of inertia and torque should be calculated with all distances measured from the c of m.

You could treat the rod and weight as a single object and find its center of mass, but you sure don't have to. (And it's easier not to.) The system will rotate about the axis, not the center of mass.

In (d) it seems to me the angle is 37 + 90 degrees because I've got it rotating clockwise.

The angle should be 53 degrees, as Dr.D explained. Luckily, since all we care about is the sine of the angle, 37 + 90 works just as well.

 

[Delphi51]

The system will rotate about the axis, not the center of mass.

Thanks Doc - I forgot all this rotational stuff about 30 years ago!
But what axis do you mean? Isn't this rod freely falling in the air? It says it is "released from rest"? Ah, maybe I'm misunderstanding that and it is still attached to the pivot.

 

[Doc Al]

But what axis do you mean? Isn't this rod freely falling in the air? It says it is "released from rest"? Ah, maybe I'm misunderstanding that and it is still attached to the pivot.

It's still attached to its pivot, just released from rest at its given angle and allowed to swing freely. (If there were no pivot and it were released from rest, it would just fall straight down without rotating at all.)

 

[Delphi51]

Oh, of course! Every part would have the same vertical acceleration.
A good lesson for me!