Prime Subfiellds - Lovett, Proposition 7.1.3 ....

[Math Amateur]

I am reading Abstract Algebra: Structures and Applications" by Stephen Lovett ...

I am currently focused on Chapter 7: Field Extensions ... ...

I need help with the proof of, or at least some remarks concerning, Proposition 7.1.3 ...Proposition 7.1.3 plus some introductory remarks (proof?) reads as follows:

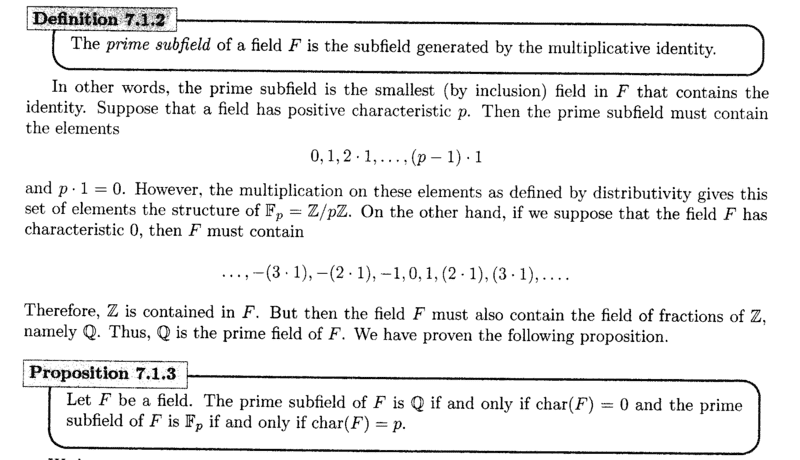
In the above text from Lovett we read the following:"... ... However, the multiplication on these elements as defined by distributivity gives this set of elements the structure of $\mathbb{F}_p = \mathbb{Z} / p \mathbb{Z}$. ... ... " ... ... BUT ... the subfield contains elements $0, 1, 2, 3, 4, 5, \ ... \ ... \ (p -1)$... and being a field, it contains divisions of these elements such as $1/2, 3/5 \ ... \ ... \ ...$... so how can this subfield be equal to $\mathbb{Z} / p \mathbb{Z}$ ... ... ?
Hope someone can help ...

Peter

 

[andrewkirk]

The missing piece is that a field's characteristic must be either zero or a prime number, otherwise there will be divisors of zero (can you see why?), which would disqualify $F$ from being a field. We then use the theorem that $\mathbb Z_p$ is a field for $p$ prime, so that the fractions you listed are all modular integers in $\mathbb Z_p$. For instance $1/2=3$ in $\mathbb Z_5$ and $3/5=2$ in $\mathbb Z_7$.

 

[Math Amateur]

Thanks for the help Andrew ...

Just reflecting on what you have said ...

However, can you give me an indication of how exactly we can demonstrate that $1/2 = 3$ in $\mathbb{Z}_5$ and $3/5 = 2$ in $\mathbb{Z}_7$ ...

Then I will get an idea of what is going on ...

Peter

 

[andrewkirk]

For the first one, $3\times 2=6 = 5+1$, so that $3_5\times 2_5=1_5$ where the 5 subscript denotes 'mod 5' (technically speaking, $k_p$ denotes $k+p\mathbb Z$).

Similarly, $2\times 5=10=7+3$, so that $2_7\times 5_7=3_7$.

A good way of thinking of it is as a clock, with the numbers 0 to p-1 marked around the outside. and a single hand. Asking 'what is 1 / 2 mod 5' is the same as asking what rotation (past how many marked numbers) must I perform 2 times, starting at 0, to end up at 1?

 

[Math Amateur]

Thanks Andrew ... really appreciate your help ..

Peter