Proof of equality of mixed partial derivatives

[Happiness]

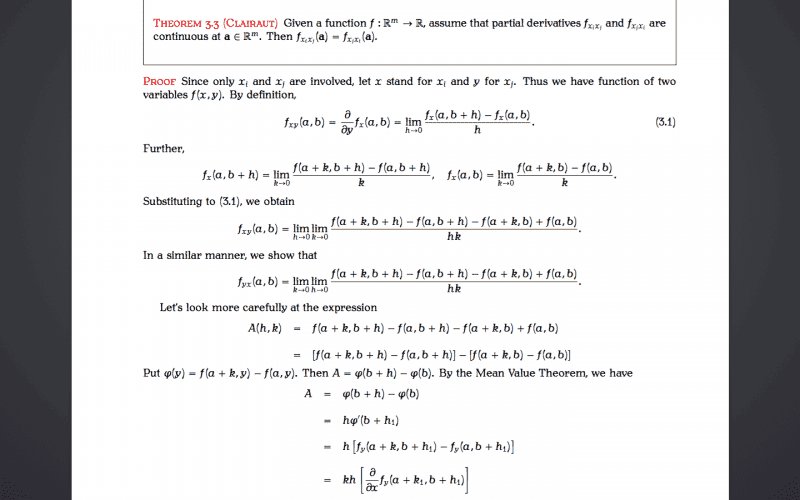
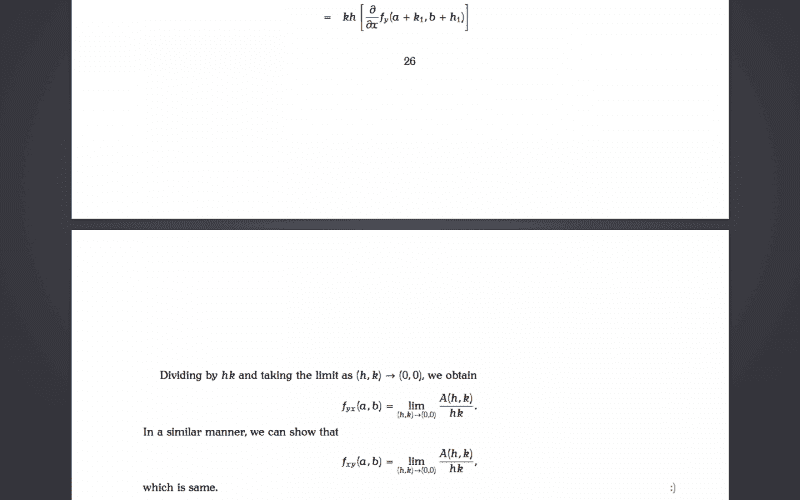
In the proof, mean value theorem is used (in the equal signs following A). Hence, the conditions for the theorem to be true would be as follows:

1. $\varphi(y)$ is continuous in the domain $[b, b+h]$ and differentiable in the domain $(b, b+h),$ and hence $f(x,y)$ is continuous in the domain $\{a, a+k\} \times [b, b+h]$ and differentiable in the domain $\{a, a+k\} \times (b, b+h)$.

2. $f_y(x,y)$ is continuous in the domain $[a, a+k] \times \{b+h_1\}$ and differentiable in the domain $(a, a+k) \times \{b+h_1\}$.

3. $f_x(x,y)$ is continuous in the domain $\{a+k_1\} \times [b, b+h]$ and differentiable in the domain $\{a+k_1\} \times (b, b+h)$.

4. Since there is a limit $(h, k) \rightarrow (0, 0)$, I presume $f(x,y), f_y(x,y)$ and $f_x(x,y)$ have to be continuous in the rectangular domain $[a, a+k] \times [b, b+h]$ and differentiable in the rectangular domain $(a, a+k) \times (b, b+h)$.

The condition stated in the theorem is that the mixed partial derivatives be continuous. How does that satisfy the 4 conditions mentioned above? Does continuity at a point imply continuity in the $\epsilon(x, y)$-neighbourhood of the point? And does differentiability at a point imply differentiability in the $\epsilon(x, y)$-neighbourhood of the point?

 

[Hawkeye18]

The assumption of the theorem is that the mixed partial are continuous at the point $(a,b)$, and it is implicitly assumed that they exist in a neighborhood $U$ (say an open rectangle) of this point. For existence of mixed partial derivatives we need existence in $U$ of first order partial derivatives $f_x$ and $f_y$.

Since any differentiable function is continuous, for existence of mixed derivative $\frac{\partial}{\partial y} f_x$ we need the partial $f_x$ to be continuous function of the variable $y$ (i.e.~it should be continuous in $y$ for any fixed $x$). Note, that is is a weaker statement than continuity of $f_x$ (in both variables $x$ and $y$). So the assumption that this mixed partial exists in $U$ implicitly implies the assumption of continuity of $f_x$ in variable $y$.

Similarly, the existence of the other mixed partial implicitly implies the assumption that the partial $f_y$ is continuous in the variable $x$.

And these assumptions are sufficient to prove the theorem, if you look carefully at the proof you presented, you will see that only these assumptions are used there.

Now about "implicit assumptions". The first one is just the author is not careful: to be absolutely rigorous, one should tell "... assume that the mixed partial derivatives exists in a neighborhood of $a\in \mathbb R^n$ and continuous at $a$.''

As for the other two, this is a perfectly acceptable way of writing, allowing to avoid writing laundry lists of assumptions. As I explained above, if the mixed partials exist then the partials $f_x$ and $f_y$ should be continuous in variables $y$ and $x$ respectively. So this continuity is a part of the assumption about existence of mixed partials, and it is common not to list such type of assumptions explicitly. A mathematician should be able to "expand" the assumptions, and see the "implicit" parts.

 

[Happiness]

When I look at this again, I don't understand why we don't need the mixed partial derivatives to be continuous in the neighbourhood of the point $(a, b)$ but only need them to be continuous at that point.

$\frac{\partial}{\partial x}f_y$ exists $\implies f_y$ is differentiable with respect to $x$ at the point $(a, b) \implies f_y$ is continuous along $x$ at the point $(a, b)$. But with just continuity at a point, we still can't use the mean value theorem, which is needed for the steps following $A$.

 

[Hawkeye18]

As I said before, one needs to assume that mixed partials exist in a neighborhood of $(a,b)$. But we do not need to assume continuity in the whole neighborhood, it is sufficient to assume continuity only at the point $(a,b)$.

 

[Happiness]

To put it explicitly:

Condition 2
$f_{yx}$ exists in the neighbourhood $\implies f_y$ is differentiable wrt $x$ and continuous along $x$ in the neighbourhood. This satisfies condition 2, required for the MVT used in the last step of page 26.

Condition 3
Similarly, $f_{xy}$ exists in the neighbourhood $\implies f_x$ is differentiable wrt $y$ and continuous along $y$ in the neighbourhood. This satisfies condition 3, required for the MVT used in the last step of page 26 for the "similar manner" case.

Condition 4
Condition 4 in post #1 is wrong. It should be "both mixed partial derivatives and $A$ and hence $f(x, y)$ have to be continuous in all directions at the point $(a, b)$", required in the last step of the proof:

$\lim_{(h,k)\to(0,0)}\frac{A(h, k)}{hk}=\lim_{(h,k)\to(0,0)}f_{yx}(a+k_1, b+h_1)=f_{yx}(a, b)$.

Since $f_{yx}$ and $f_{xy}$ are continuous in all directions at the point $(a, b)$ by the premise, and the continuity of $f_{yx}$ and $f_{xy}$ separately implies the continuity of $f(x, y)$ along $x$ and along $y$, condition 4 is satisfied.

Condition 1
Lastly, $f_{yx}$ exists in the neighbourhood $\implies f_y$ exists in the neighbourhood $\implies f(x, y)$ is differentiable wrt $y$ and continuous along $y$ in the neighbourhood. This satisfied condition 1, required in the first step following $A$. Similarly, the existence of $f_{xy}$ in the neighbourhood satisfies condition 1 for the "similar manner" case.

 

[Happiness]

As I said before, one needs to assume that mixed partials exist in a neighborhood of $(a,b)$. But we do not need to assume continuity in the whole neighborhood, it is sufficient to assume continuity only at the point $(a,b)$.

I have 2 remaining questions:

1. Does the continuity of $f(x, y)$ along $x$ and along $y$ implies its continuity along all directions, which is required in the last step of the proof? If so, how?

Or do we obtain continuity along all directions as follows:
Since $f_x$ and $f_y$ exists and are continuous in the neighbourhood, $f(x, y)$ is differentiable at the point $(a, b)$. Since it is differentiable, all directional derivatives exist at the point. Hence, $f(x, y)$ is continuous in all directions at the point. But for this method to work, we require $f_x$ and $f_y$ to be continuous in all directions in the neighbourhood. But as mentioned before, we don't have continuity in all directions:

for existence of mixed derivative $\frac{\partial}{\partial y} f_x$ we need the partial $f_x$ to be continuous function of the variable $y$ (i.e.~it should be continuous in $y$ for any fixed $x$). Note, that is is a weaker statement than continuity of $f_x$ (in both variables $x$ and $y$). So the assumption that this mixed partial exists in $U$ implicitly implies the assumption of continuity of $f_x$ in variable $y$.

2. Do we need the mixed partials to exist in a closed neighbourhood or an open neighbourhood?

Conditions 1-3 require continuity of $f$, $f_y$ and $f_x$ at the end points where $x=a+k$ and $y=b+h$. If the mixed partials exist only in an open neighbourhood, then conditions 1-3 cannot be satisfied. But according to this website, only an open neighbourhood is needed:
http://calculus.subwiki.org/wiki/Clairaut's_theorem_on_equality_of_mixed_partials

 

[Hawkeye18]

Here is the full list of assumptions needed for the theorem in your original post.

0. Function $f$ is defined in an open neighborhood $U$ of $(a,b)$.
1. Partial $f_x$ exists at any point of $U$
2. Partial $f_y$ exists at any point of $U$
3. Derivative $f_{x,y}:=\frac{\partial f_x}{\partial y}$ exists at any point of $U$
4. Derivative $f_{y,x}:=\frac{\partial f_y}{\partial x}$ exists at any point of $U$
5. Both $f_{x,y}$ and $f_{y,x}$ are continuous at the point $(a,b)$

The conclusion then will be that $f_{x,y}(a,b) = f_{y,x}(a,b)$.

The conditions 1 implies that
1'. $f$ is continuous in $x$ at all points in $U$,
and similarly, the condition 2 implies that
2.' $f$ is continuous in $y$ at all points in $U$ (this condition is used when the MVT is applied first time in computing $A$ on p. 26).

The condition 3 implies that
3.' $f_x$ is continuous in $y$ at all points in $U$,
and similarly, condition 4 implies
4'. $f_y$ is continuous in $x$ at all points in $U$ (this condition is used when the MVT is applied the second time in computing $A$ on p. 26).

The conditions 1', 2', 3', 4' and 5 are the only continuity assumptions needed.

Finally, about closed vs open neighborhoods. We only need to consider $A(h,k)$ only for all sufficiently small $h$ and $k$, so the whole closed rectangle will be in $U$. Then the necessary continuity assumptions for MVP will be satisfied at endpoints, because they are satisfied in $U$.

 

[Happiness]

The conditions 1', 2', 3', 4' and 5 are the only continuity assumptions needed.

Thank you!

I was mistaken when I thought the continuity of $A$ is needed in the last step.