Taylor expansion fine structure

[darkshadow28]

I have to do a Taylor expansion of the energy levels of Dirac's equation with a coulombian potential in orders of (αZ/n)^2 , but the derivatives I get are just too large, I guess there is another approach maybe?
This is the expression of the energy levels

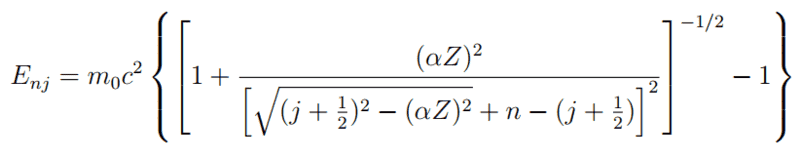
And i know it has to end like this:

 

[mfb]

You only need two derivatives. The second one will be ugly but you evaluate it at $\alpha Z=0$, which means you can skip many terms as soon as you know that appears as factor.

 

[Charles Link]

A simpler way is to first expand $\sqrt{(j+1/2)^2-(\alpha Z)^2}$ as a Taylor series. It greatly simplifies the denominator. Upon squaring the term in brackets $[ \, ]$, I get $n^2(1-\frac{(\alpha Z)^2}{n(j+1/2)} +...)$. This makes $1/ [1+(\frac{\alpha Z}{n})^2(1-\frac{(\alpha Z)^2}{n(j+1/2)} )]^{1/2}-1$. With this very last Taylor expansion with a $f(x)=\sqrt{1+x}$, it is important to also keep the second order term of the $(\frac{\alpha Z}{n})^2$ factor. I almost got complete agreement with the result you posted, but I got a 1/4 instead of 3/4 in the fourth order term. $\\$ Editing: One additional item, and I think I got their result: In taking the final result in the form $f(x)=\frac{1}{1+x}=1-x+x^2-x^3+ ...$, it is important to retain the $x^2$ term of the $\frac{1}{2} (\frac{ \alpha Z}{n})^2$ term. (Originally, I tried to use $\frac{1}{1+x} \approx 1-x$. That did not supply sufficient accuracy.) This will then give the result you provided with the 3/4.

 

[darkshadow28]

A simpler way is to first expand $\sqrt{(j+1/2)^2-(\alpha Z)^2}$ as a Taylor series. It greatly simplifies the denominator. Upon squaring the term in brackets $[ \, ]$, I get $n^2(1-\frac{(\alpha Z)^2}{n(j+1/2)} +...)$. This makes $1/ [1+(\frac{\alpha Z}{n})^2(1-\frac{(\alpha Z)^2}{n(j+1/2)} )]^{1/2}-1$. With this very last Taylor expansion with a $f(x)=\sqrt{1+x}$, it is important to also keep the second order term of the $(\frac{\alpha Z}{n})^2$ factor. I almost got complete agreement with the result you posted, but I got a 1/4 instead of 3/4 in the fourth order term. $\\$ Editing: One additional item, and I think I got their result: In taking the final result in the form $f(x)=\frac{1}{1+x}=1-x+x^2-x^3+ ...$, it is important to retain the $x^2$ term of the $\frac{1}{2} (\frac{ \alpha Z}{n})^2$ term. This will then give the result you provided with the 3/4.

Thanks, if I try doing from $n^2(1-\frac{(\alpha Z)^2}{n(j+1/2)} +...)$ i get the correct terms, but I'm getting an extra n(j+1/2) in the Taylor series of the square root.

 

[Charles Link]

In your first Taylor series, you need to write $(j+1/2) \sqrt{1-(\frac{\alpha Z}{j+1/2})^2}=(j+1/2)(1-\frac{1}{2} \frac{(\alpha Z)^2}{(j+1/2)^2}+...)$. The $j+1/2$ out front gets canceled by the $-(j+1/2)$ in the denominator. And next, factor out an $n^2$ before you square the result.