In case anybody, like me, doesn't want to read through all of this? But still wants to see it resolved
correctly (which very seldom happens)? I decided to add a response at the end of the thread. Hopefully it will be closed soon.
cmb said:
Let's say that just at the moment you are about to decide whether to swap doors or not, you go sick and your quiz partner steps in. They are now presented with two doors, which, for sure, one has the car and one has the goat. There is simply no possible way that the probability for them is not 50:50! Yet, somehow the probability for you was different?
Instead, let's say I will roll a special six-sided die. The sides are painted either red or green. Two are painted one of those colors, and four are painted the other color. I tell Alice which color is on four sides, but not Bob. I ask both to bet on which color will come up.
If Alice bets on the color that I told her is on four of the sides, she has a 2/3 chance of winning her bet. Bob has no idea which color predominates, and has to guess. So he has a 50:50 chance of guessing right. If he does, he has a 2/3 chance like Alice. If he guesses wrong, he has a 1/3 chance. His overall chances are thus:
(2/3)*(1/2) + (1/3)*(1/2) = 1/2.
This is exactly analogous to cmb's scenario. According to the usual interpretation, you (like Alice) have information that says one choice has a 2/3 chance. Your quiz partner (like Bob) does not, and must guess. This is how probability works, and it makes perfect sense if you understand probability.
But this doesn't say why your information means that one choice has a 2/3 chance. The usual explanation is that your original chances, 1/3, can't change. That's wrong. They in fact
can change, but nothing you know
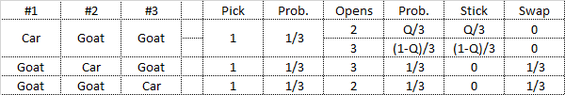
allows this change. To see why, let's examine a slightly modified version of cmb's table:
This isn't the way it should be displayed - we should fix the player's choice and let the location of the car vary - but it works the same as long as we assume the choice is random. I added the probability of reaching each state at two points in time - before a door is opened, and after. Then, under "Stick" and "Swap," I carried over the probability that each choice was a winner. As you can see, the chances that swapping wins total to 2/3.
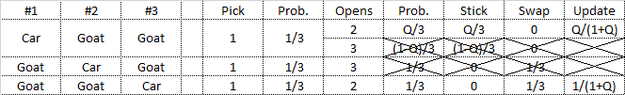
What cmb did was count the number of rows where Sticking, or Swapping, would win. That only works if there is no difference between the rows. My table shows that there is a difference. But that isn't the only mistake in this table. A better one is this, where I do switch to fixing the player's pick at door #1 while letting the car placement vary:
But I also added a new variable: Q is the probability, when the Host can open either Door #1 or #2, that he chooses #1. The chances for Staying and Swapping still sum to 1/3 and 2/3, respectively, but one piece of information is ignored. The player knows which door was opened. If it was #2, we have to update the probabilities like this:
The point is that the chances when you stay
can change, but only if you know that Monty Hall chooses with a bias. If Q=1/2, which means an unbiased choice, the probabilities evaluate to 1/3 and 2/3.