Understanding a sequence in P&S

[looseleaf]

I was wondering how to deal with this step in Peskin and Schroeder:

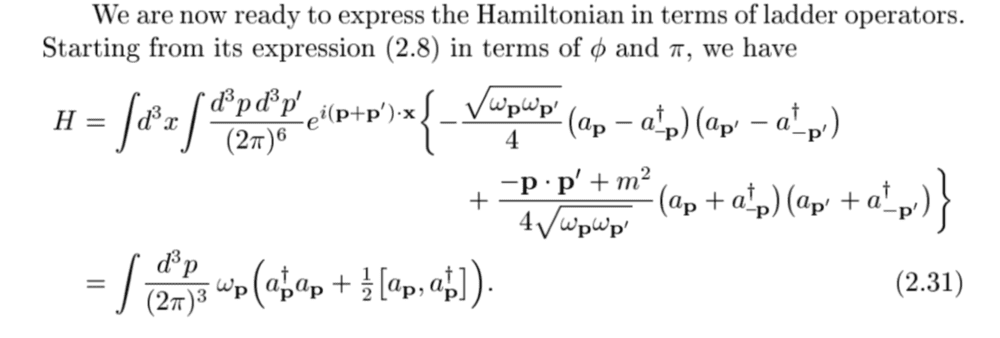
So first you make the delta fn. from the exponential as d(p - (- p')), then what do you do with the creation/annihilation operators that have a negative subscript? I don't have to go into position/momentum representation do I?..

 

[vanhees71]

You just do the integrals. Of course you integrate over $\vec{x}$ first. Then the integral over $\vec{p}'$ is trivial. Finally you use the commutation relations.

Note that (2.31) is a non-sensical expression and think about, why you can simply erase the nonsensical part ;-))).

 

[HomogenousCow]

Note that (2.31) is a non-sensical expression and think about, why you can simply erase the nonsensical part ;-))).

Imagine that, expressions of dubious existence in QFT? On a more serious note, is there a way to remedy these expressions in QFT without appealing to a lattice theory?

 

[vanhees71]

This is the first time where the sloppy math of us physicists hits back in QFT: The commutator is a nonsensical result since it's proportional with $\delta^{(3)}(0)$ (which hand-wavingly argued is alread infinite), which then is even made "more infinite" by integrating over $\omega_{\vec{p}}$.

The cure is very simple: As long as you do not refer to gravity the absolute value of energy doesn't matter since only energy differences are observable. All you want is a definition of the free-field Hamiltonian, which represents energy and the time evolution of the field operators (and any operators representing observables built from them) in the interaction picture. Thus you can simply through the non-sensical term away, because it's "an infinite operator proportional to the unit operator". Then you are left with a well-defined free Hamiltonian.

Now you can check that this "renormalized" free Hamiltonian satisfies the properties it should, i.e., that it leads to a useful time evolution for the field operators and observables.

 

[HomogenousCow]

This is the first time where the sloppy math of us physicists hits back in QFT: The commutator is a nonsensical result since it's proportional with $\delta^{(3)}(0)$ (which hand-wavingly argued is alread infinite), which then is even made "more infinite" by integrating over $\omega_{\vec{p}}$.

The cure is very simple: As long as you do not refer to gravity the absolute value of energy doesn't matter since only energy differences are observable. All you want is a definition of the free-field Hamiltonian, which represents energy and the time evolution of the field operators (and any operators representing observables built from them) in the interaction picture. Thus you can simply through the non-sensical term away, because it's "an infinite operator proportional to the unit operator". Then you are left with a well-defined free Hamiltonian.

Now you can check that this "renormalized" free Hamiltonian satisfies the properties it should, i.e., that it leads to a useful time evolution for the field operators and observables.

I'm aware of this standard approach to the issue however I was wondering if there was some way to reformulate continuum QFT to avoid all of these nasty "operator-distribution"-type issues. Is the Hamiltonian in the creation-annihilation representation even a sensible expression? I thought we couldn't multiply operator distributions, much less integrate over such products with an unbounded measure. Something just feels deeply wrong about these basic expressions in QFT.

 

[looseleaf]

You just do the integrals. Of course you integrate over $\vec{x}$ first. Then the integral over $\vec{p}'$ is trivial. Finally you use the commutation relations.

Ok, I did those two integrals, then factor out the $\omega_p$ and foil out the c/a operators to get
$$ \frac{1}{2}(a_p a_p^\dagger + a_{-p}a_{-p}^{\dagger}) $$

I see how this is equivalent to the final expression if -p = p, but how do you deal with those negative momenta? Can you just flip them to positive without worrying because of some symmetry?

 

[HomogenousCow]

Can you just flip them to positive without worrying because of some symmetry?

Yes because the energies are even functions of the momenta and the minus signs between the differentials and the integral limits cancel.

 

[looseleaf]

Yes because the energies are even functions of the momenta and the minus signs between the differentials and the integral limits cancel.

Thanks! So do you mean that the limits of integration are $(-\infty , \infty)$ which could just as well be $(\infty, -\infty)$?

 

[DarMM]

I'm aware of this standard approach to the issue however I was wondering if there was some way to reformulate continuum QFT to avoid all of these nasty "operator-distribution"-type issues. Is the Hamiltonian in the creation-annihilation representation even a sensible expression?

Yes you must Wick order them with respect to the theory's vacuum. So rather than $\phi^{4}$ you must use $:\phi^{4}:$
Similarly for all fields in the Hamiltonian. The Wick ordered Hamiltonian then does not have the problematic term.

 

[HomogenousCow]

Yes you must Wick order them with respect to the theory's vacuum. So rather than $\phi^{4}$ you must use $:\phi^{4}:$
Similarly for all fields in the Hamiltonian. The Wick ordered Hamiltonian then does not have the problematic term.

So basically, the "pre-quantization" stage with the Lagrangian is just an ad-hoc way to obtain a rough form of the Hamiltonian, after which it has to be wick-ordered to obtain a sensible theory?

 

[vanhees71]

Ok, I did those two integrals, then factor out the $\omega_p$ and foil out the c/a operators to get
$$ \frac{1}{2}(a_p a_p^\dagger + a_{-p}a_{-p}^{\dagger}) $$

I see how this is equivalent to the final expression if -p = p, but how do you deal with those negative momenta? Can you just flip them to positive without worrying because of some symmetry?

Just substitute $\vec{p}'=-\vec{p}$ in the second integral.

 

[DarMM]

So basically, the "pre-quantization" stage with the Lagrangian is just an ad-hoc way to obtain a rough form of the Hamiltonian, after which it has to be wick-ordered to obtain a sensible theory?

The form of the Hamiltonian is correct, it's just an issue with constructing the quantization. If the classical field $\phi$ is quantized to $\hat{\phi}$, then $\phi^{4}$ is quantized to $:\hat{\phi}^{4}:$

The Hamiltonian is fine, it's just an issue of how to quantize powers of the field.

 

[DarMM]

Though note one interesting thing, if $j^{\mu}$ is a conserved current in the classical theory it can be the case that $\partial_{\mu}:\hat{j}^{\mu}:\neq 0$. This is the origin of anomalies.

 

[HomogenousCow]

The form of the Hamiltonian is correct, it's just an issue with constructing the quantization. If the classical field $\phi$ is quantized to $\hat{\phi}$, then $\phi^{4}$ is quantized to $:\hat{\phi}^{4}:$

The Hamiltonian is fine, it's just an issue of how to quantize powers of the field.

Is this somehow related to Groenewold's theorem?

 

[DarMM]

Is this somehow related to Groenewold's theorem?

Not exactly.

In NRQM you really only have one choice for $\hat{q}$ and $\hat{p}$ (Stone-Von Neumann theorem). However there is then no unique choice for $\hat{Q}\left(q,p\right)$ in general. The space of quantum operators is larger than the space of classical functions of $q,p$ and there is no canonical map between them. That's Groenewold's theorem.

In QFT there are infinite choices of $\hat{q}$ and $\hat{p}$, that is $\hat{\phi}$ and $\hat{\pi}$, but first of all the quantisation of $\hat{\phi}$ and $\hat{\pi}$ does not straight forwardly extend to monomials of either. In QM monomials were no problem, even with Groenewold's theorem. There the issue is that polynomials are not uniquely quantised, here in QFT the problem is that the quantisation of monomials is undefined.

The Wick ordering then defines the monomials (and products of fields typically appearing in the Hamiltonian) as operators. However it will turn out that the Hamiltonian is typically not self-adjoint or semi-bounded except for a specific choice of $\hat{\phi}$ and $\hat{\pi}$.

This latter part is the origin of the infinities in QFT, only for a single choice/representation of the field operators do the Wick orderings produce powers that give a well-defined Hamiltonian.

 

[HomogenousCow]

Not exactly.

In NRQM you really only have one choice for $\hat{q}$ and $\hat{p}$ (Stone-Von Neumann theorem). However there is then no unique choice for $\hat{Q}\left(q,p\right)$ in general. The space of quantum operators is larger than the space of classical functions of $q,p$ and there is no canonical map between them. That's Groenewold's theorem.

In QFT there are infinite choices of $\hat{q}$ and $\hat{p}$, that is $\hat{\phi}$ and $\hat{\pi}$, but first of all the quantisation of $\hat{\phi}$ and $\hat{\pi}$ does not straight forwardly extend to monomials of either. In QM monomials were no problem, even with Groenewold's theorem. There the issue is that polynomials are not uniquely quantised, here in QFT the problem is that the quantisation of monomials is undefined.

The Wick ordering then defines the monomials (and products of fields typically appearing in the Hamiltonian) as operators. However it will turn out that the Hamiltonian is typically not self-adjoint or semi-bounded except for a specific choice of $\hat{\phi}$ and $\hat{\pi}$.

This latter part is the origin of the infinities in QFT, only for a single choice/representation of the field operators do the Wick orderings produce powers that give a well-defined Hamiltonian.

What sets these different representations apart?

 

[DarMM]

What sets these different representations apart?

Different choices of operators obeying the CCR. In NRQM it turns out all choices are unitarily equivalent.

For example
\begin{align*}
\hat{x} & = x\\
\hat{p} & = -i\hbar\frac{\partial}{\partial x}
\end{align*}
is one choice, but
\begin{align*}
\hat{x} & = x\\
\hat{p} & = -i\hbar\frac{\partial}{\partial x} + i\ln\left(x\right)
\end{align*}
is another.

One acts on the Hilbert space of square integrable functions under the measure $dx$, the other on the Hilbert space of square integrable functions under $e^{-x^2}dx$. However these choices are related under the unitary transformation $e^{x^2}$.

In QFT the choices are not unitarily related.