- #1
peguerosdc
- 28
- 7
- Homework Statement
- If ##\phi## is the potential due to a volume-charge density ##\rho##within a volume V and a surface-charge density ##\sigma##on the conducting surface S bounding the volume V, while ##\phi '## is the potential due to another charge distribution ##\rho##and##\rho'##, prove Green's reciprocity theorem.
- Relevant Equations
- Green's reciprocity theorem:
## \int_V \rho \phi' dV + \int_S \sigma \phi' dS = \int_V \rho' \phi dV + \int_S \sigma' \phi dS ##
This is Jackson's 3rd edition 1.12 problem.
So, for both ## \phi ## and ## \phi' ##, I started from Green's second identity:
## \int_V ( \phi \nabla^2 \phi' - \phi' \nabla^2 \phi )dV = \int_S ( \phi \frac {\partial \phi'} {\partial n} - \phi' \frac {\partial \phi} {\partial n} ) dS ##
And used Poisson's equation ## \nabla^2 \phi = - \frac \rho \epsilon ## and Gauss's law and ## (E = - \nabla \phi) ## to get the relation ## \frac {\partial \phi} {\partial n} = - \frac \sigma \epsilon ## between the surface charge density and the electric potential, which resulted in:
## \int_V \rho \phi' dV - \int_S \sigma \phi' dS = \int_V \rho' \phi dV - \int_S \sigma' \phi dS ##
So this is where I am stuck. I have checked many solution's on the internet and they say that the key is to realize that the normals ##n## in Gauss's law equations are pointing out of the conductor (actually, Jackson says: "where ## \frac {\partial} {\partial n} ## is the normal derivative at the surface S directed outwards from inside the volume V"), whereas the normals in Green's theorem are pointing into the conductor, so you can flip the - signs to end with the identity you want to prove. This is how I picture this (not sure is this correct):
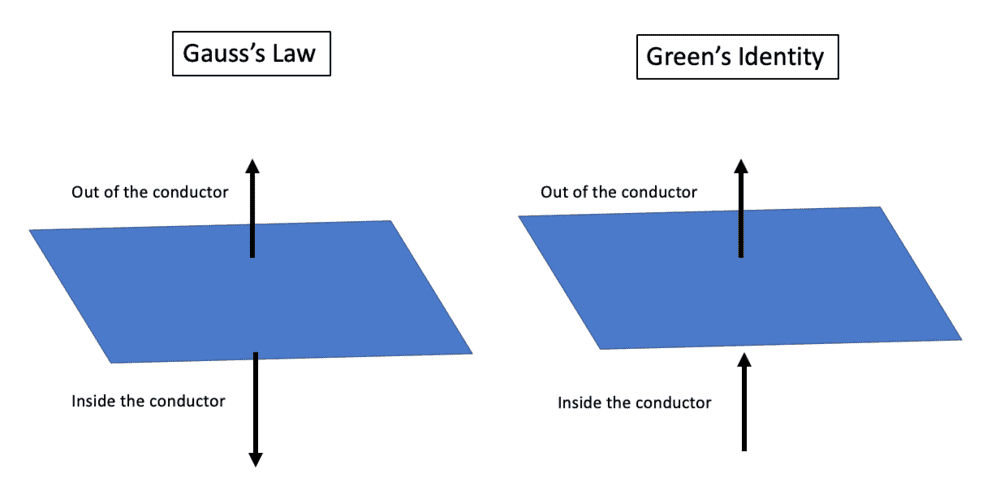
Why is this the case? This made me realize that I don't understand how to derive Green's identity as I don't get why we are considering the normals in that direction. And, if my drawing is correct, we are only changing the sign inside the conductor where ## E = 0 ##, so why does this even matter?
And finally, what is the physical meaning of this theorem? I see a relationship between the work ## W = \frac 1 2 \int \rho \phi dV ## which gets you the energy required to assemble charge distribution ##\rho## in an electric potential ##\phi##, so I read Green's reciprocity identity as "the energy required to assemble a system made of a charge distribution ##\rho## and ##\sigma## in a potential produced by ##\rho'## and ##\sigma'## is the same energy required to assemble a system made of a charge distribution ##\rho'## and ##\sigma'## in a potential produced by ##\rho## and ##\sigma##" . Is my understanding correct?
So, for both ## \phi ## and ## \phi' ##, I started from Green's second identity:
## \int_V ( \phi \nabla^2 \phi' - \phi' \nabla^2 \phi )dV = \int_S ( \phi \frac {\partial \phi'} {\partial n} - \phi' \frac {\partial \phi} {\partial n} ) dS ##
And used Poisson's equation ## \nabla^2 \phi = - \frac \rho \epsilon ## and Gauss's law and ## (E = - \nabla \phi) ## to get the relation ## \frac {\partial \phi} {\partial n} = - \frac \sigma \epsilon ## between the surface charge density and the electric potential, which resulted in:
## \int_V \rho \phi' dV - \int_S \sigma \phi' dS = \int_V \rho' \phi dV - \int_S \sigma' \phi dS ##
So this is where I am stuck. I have checked many solution's on the internet and they say that the key is to realize that the normals ##n## in Gauss's law equations are pointing out of the conductor (actually, Jackson says: "where ## \frac {\partial} {\partial n} ## is the normal derivative at the surface S directed outwards from inside the volume V"), whereas the normals in Green's theorem are pointing into the conductor, so you can flip the - signs to end with the identity you want to prove. This is how I picture this (not sure is this correct):
Why is this the case? This made me realize that I don't understand how to derive Green's identity as I don't get why we are considering the normals in that direction. And, if my drawing is correct, we are only changing the sign inside the conductor where ## E = 0 ##, so why does this even matter?
And finally, what is the physical meaning of this theorem? I see a relationship between the work ## W = \frac 1 2 \int \rho \phi dV ## which gets you the energy required to assemble charge distribution ##\rho## in an electric potential ##\phi##, so I read Green's reciprocity identity as "the energy required to assemble a system made of a charge distribution ##\rho## and ##\sigma## in a potential produced by ##\rho'## and ##\sigma'## is the same energy required to assemble a system made of a charge distribution ##\rho'## and ##\sigma'## in a potential produced by ##\rho## and ##\sigma##" . Is my understanding correct?