Can conservation of angular momentum cause rotational phase shift?

[diffusegrey]

So, I'm trying to study phase shift in an unbalanced rotating system (where "phase shift" means the resulting response of orbital motion lags behind in time/position relative to the force that's causing it)

I know that conservation of angular momentum applies ideally in a closed system. Would it apply in any form in a system like the following? ...

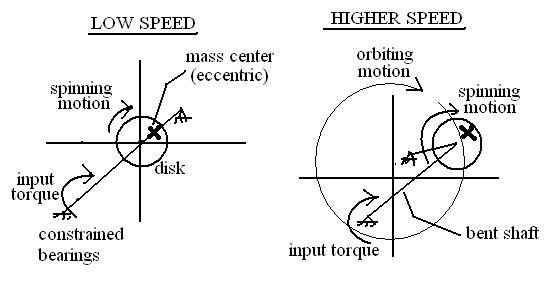
Take a highly unbalanced disk (like in a Jeffcott rotor, for anyone familiar), connected by a flexible shaft constrained at both ends. Assume that a constant steady input torque is applied to the shaft/disk at its radial center, creating a constant increase in angular velocity. With added speed, the shaft will increasingly bend and the disk will deflect and orbit in some enlarging translational path, while still spinning synchronously. The torque still remains applied to the radial center of the disk. Assume the shaft and supports are isotropic, and create a circular orbit.

Since the same input torque is causing both the spin and the translation orbit, causing the spin directly and indirectly causing the orbit (via reactive centrifugal force), is it possible to "normalize" the torque input and consider the two motions in a comparative manner as if it were a closed system? The same object with the same single input torque is in two rotational motions, but one rotation has a steadily increasing radius while the other rotation has no change in radius.

In this situation, would some form of conservation of angular momentum cause the angular velocity of the object's orbit (with growing radius) to increase more slowly relative to the angular velocity of the object's spin (with a constant zero-radius)?

*****
It's well known that this relative slowing (phase shift) happens in practice, but it is classically attributed only to external damping in the supports/bearings and/or to internal damping in the shaft material (both cases are typically modeled as a linear mass-spring-damper system). But in this example, there is no motion in the bearings (like in fixed ball bearings for instance), and since the shaft is synchronously orbiting in a circular path, with the same side always facing outward, there is no oscillation in the material of the shaft. Since damping requires motion/velocity to do anything, damping wouldn't have a source here it seems.

 

[AlephZero]

If the damping is zero, then for steady motion at a constant rotation speed the shaft bends so that unbalanced mass is always at phase angle 0 degrees below the critical (whirling) speed, and 180 degrees above the whirling speed. At the whirling speed, a model that assumes small strains, no damping, and linear behavior breaks down and predicts the size of the orbit would be infinite.

If you apply a torque to the rotor to accelerate it, you can get through the whirling speed without "infinitely large" displacements, but you have to model the the transient dynamics of the whole system. For example the size of the orbit depends on the rate of acceleration of the rotor, because the unbalanced mass has inertia and can't move "instantly" to a new equilibrium position.

Another variation on this scenario is to consider a balanced rotor running above its whirling speed, (with no bending of the shaft), and suddenly make it unbalanced - for example a piece of the rotor disk breaks off. With no damping, the motion starts with the rotor bending "heavy side outwards" but (in a rotating frame of reference fixed to the shaft) the center of the disk then moves in a curved path and ends up "light side outwards". You can explain that motion in terms of the Coriolis forces on the disk, which are stilll present even for a Jeffcott rotor which has no gyroscopic forces.

There is no violation of Newtonian mechanics here, and you don't need damping forces to explain the behavior. Of course if there are damping forces, the phase angle changes gradually from 0 to 180 degrees as the rotor speed increases through the whirling speed (it is 90 degrees at the whirling speed), and the size of the orbit remains finite.

 

[diffusegrey]

Thanks for the reply. By the way, where you said...

a piece of the rotor disk breaks off. With no damping, the motion starts with the rotor bending "heavy side outwards" but (in a rotating frame of reference fixed to the shaft) the center of the disk then moves in a curved path and ends up "light side outwards". You can explain that motion in terms of the Coriolis forces on the disk,

Does mean that the shaft naturally "self-balances" any sudden unbalance change if it happens when the speed is above the critical, in the same way as an integrated unbalance "self-centers" as it passes through the critical from 0 to 180 deg? Or is this a different mechanism in the instantaneous change when already above the critical?


Continuing the original topic though...
Now, this may be mixing up the idealized with the practical, but what allows a rotor to remain at steady state at some intermediate phase lag angle? If you follow a standard mathematical model and use no damping, then of course it looks like there is an instant flip from 0 to 180 degrees, so then there is no such thing as holding at say, 45 degrees lag. And by the standard model, if you got to a 45 degree lag, that could only have been by system damping in the first place.

But what happens in a real life system of a flexible shaft in non-moving ball bearings (no damping), where the unbalanced shaft is whirling in a circular orbit (so no internal oscillation or damping), at some steady speed a little below the critical peak? The centrifugal force will have deflected the shaft outward, and with this rise in shaft bend and orbit size would come some phase angle lag - and if input torque were maintained to keep this speed steady, the system would keep rotating with this larger orbit and with some steady amount of phase lag. What force(s) would create and then maintain this phase lag angle in the rotor-bearing system, if we have no damping in the system?

I understand the standard mathematical model likely can't create this situation unless it throws in damping, since it's based on extrapolating a linear type oscillation. But then that doesn't necessarily accurately represent the full physical event.

 

[AlephZero]

Does mean that the shaft naturally "self-balances" any sudden unbalance change if it happens when the speed is above the critical, in the same way as an integrated unbalance "self-centers" as it passes through the critical from 0 to 180 deg? Or is this a different mechanism in the instantaneous change when already above the critical?

That's correct. The point I was making was that it starts out moving the "wrong" way to self-balance.

But what happens in a real life system of a flexible shaft in non-moving ball bearings (no damping), where the unbalanced shaft is whirling in a circular orbit (so no internal oscillation or damping), at some steady speed a little below the critical peak?

There is always some source of damping. The bearings will never be perfectly rigid, and the cyclic deformation caused by the rotating unbalanced loads will absorb energy. The viscosity of the lubricating oil in the bearings will also absorb energy. There is also damping caused by air resistance. Every disk on the rotor acts like a (not very efficient) centrifugal air pump.

Modeling the damping accurately can be difficult, but a model with no damping is never "correct", even if it might be useful for some practical purposes.

 

[diffusegrey]

Would you say then that damping is a "driver" of behavior, or a "reaction" to it? What I mean is, how would the phase lag created by damping so ideally correlate with the rotor-bearing system critical speed to produce the right phase lag angle at the right speed, and bring the rotor to a perfect 90-degree lag at (or near, in practice) the critical speed and to 180 degrees following it?

Does damping just incidentally react/interact with the larger orbit motion that is created with higher centrifugal force with higher speed, and then by chance correlate to a 90 degree phase lag at the critical peak?

Or, is damping the fundamental behavior, which drives phase lag which then drives the nature of the response peak across the critical speed, such that when at a 90 degree lag, it governs the system in such a way as to allow a peak amplitude response there?

I know that with different amounts of damping, the critical speed response peak remains near the same speed, with only the peak amplitude altered, along with the rise and fall spread out over a wider speed range. This would imply that the root rotor behavior is independent of damping, and that damping only reacts to the motion and alters the nature of the response. But then this suggests that the rotor motion itself is the fundamental behavior (its deflection from centrifugal force), and that damping can only react to it and not actually drive anything of itself - so then how does the phase lag so perfectly correlate to the peak response speed if it's caused by damping and damping isn't the fundamental driver?