Finite Integral Domains .... Adkins & Weintraub, Propn 1.5

[Math Amateur]

I am reading "Algebra: An Approach via Module Theory" by William A. Adkins and Steven H. Weintraub ...

I am currently focused on Chapter 2: Rings ...

I need help with an aspect of the proof of Proposition 1.5 ... ...

Proposition 1.5 and its proof read as follows:

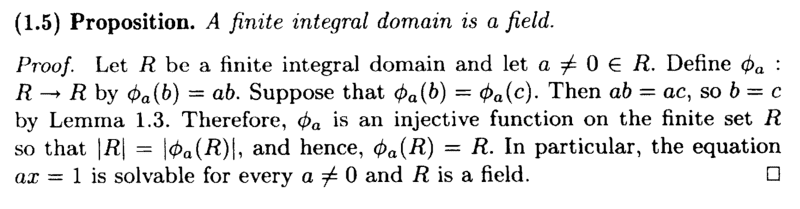At the end of the above proof from Adkins and Weintraub we read the following:

" ... ... and hence $\phi_a (R) = R$. In particular, the equation $ax = 1$ is solvable for every $a \neq 0$ and $R$ is a field. ... ... "
Can someone please explain to me how the conclusion that "the equation $ax = 1$ is solvable for every $a \neq 0$ and $R$ is a field" follows from the arguments preceding it ...

Basically I do not understand how the arguments before this statement lead to the conclusion ...Help will be much appreciated ...

Peter

 

[fresh_42]

The author showed, that $\phi_a$ is bijective, because it is injective and surjective: $\phi_a(R)=R$.
That means $a \cdot x = \phi_a(x) = 1$ has exactly one solution $x \in \phi_a^{-1}(\{1\})$.

 

[Math Amateur]

The author showed, that $\phi_a$ is bijective, because it is injective and surjective: $\phi_a(R)=R$.
That means $a \cdot x = \phi_a(x) = 1$ has exactly one solution $x \in \phi_a^{-1}(\{1\})$.

Thanks fresh_42 ...

Reflecting on what you have said ...

But ... hmm ... yes ... seems right ...

Thanks again ...

Peter

 

[lpetrich]

Integral domains and Fields has a nice proof that every finite integral domain is a field. It is much like the proof that every element of a finite group has a finite order. One takes some nonzero element a and multiplies it by itself until one comes with two exponents m and n such that a^m = aⁿ. Then,

a^m - aⁿ = aⁿ * (a^m-n - 1) = 0

From the definition of integral domain, either aⁿ = 0 or a^m-n - 1 = 0. In the first case, aⁿ = a * a^n-1, and if it equals 0, then either a or a a^n-1 equals 0. Continuing for a^n-1, we find that a = 0, contrary to our condition for a.

But if a^m-n - 1 = 0, then a * a^m-n-1 = 1. Thus, a has a multiplicative inverse, and thus every finite integral domain is a field.