- #1
dave202
- 1
- 1
<Moderator's note: Image added because otherwise the thread might once become unreadable.>
I have reason to believe this could have applications in physics, but right now it's just a mathematical result I came across recently. Either way, I think it is very interesting and fun to look at.
This is my best attempt at explaining it in words:
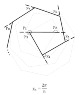
The curve generated by a series of unitary line segments placed end to end with a deviation angle given by the harmonic series, γn=2π/n, approximates a logarithmic spiral given by the polar equation r=eθ/2π. This “angular harmonic” series (γn=2π/n) is the series of external angles of all regular polygons, where n is the number of sides.
More generally, the deflection angle can be given by any scalar multiple of the harmonic series, i.e. γn=x/n, for any real (or imaginary?) number x. In this general case, the curve approximates a spiral given by the equation r=eθ/x.
Figures:
https://imgur.com/a/ZBAF2
I have reason to believe this could have applications in physics, but right now it's just a mathematical result I came across recently. Either way, I think it is very interesting and fun to look at.
This is my best attempt at explaining it in words:
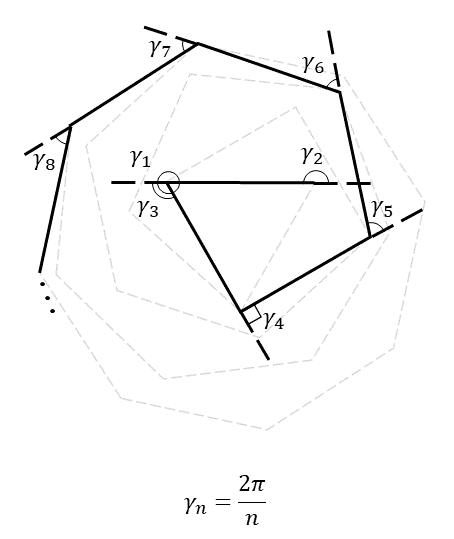
The curve generated by a series of unitary line segments placed end to end with a deviation angle given by the harmonic series, γn=2π/n, approximates a logarithmic spiral given by the polar equation r=eθ/2π. This “angular harmonic” series (γn=2π/n) is the series of external angles of all regular polygons, where n is the number of sides.
More generally, the deflection angle can be given by any scalar multiple of the harmonic series, i.e. γn=x/n, for any real (or imaginary?) number x. In this general case, the curve approximates a spiral given by the equation r=eθ/x.
Figures:
https://imgur.com/a/ZBAF2