- #1
TroyElliott
- 59
- 3
- TL;DR Summary
- Stuck on some algebra within an integral.
I am not seeing how the v goes away in the third equal sign of equation (1.8). It seems to be that it must be cos(z*sinh(u+v)), not cos(z*sinh(u)).
In the defined equations (1.7), the variable "v" can become imaginary, so a simple change of variables would change the integration sign by adding an imaginary term - not what occurs in (1.8). Any idea on how to get the third line of (1.8)?
Thanks for any insight!
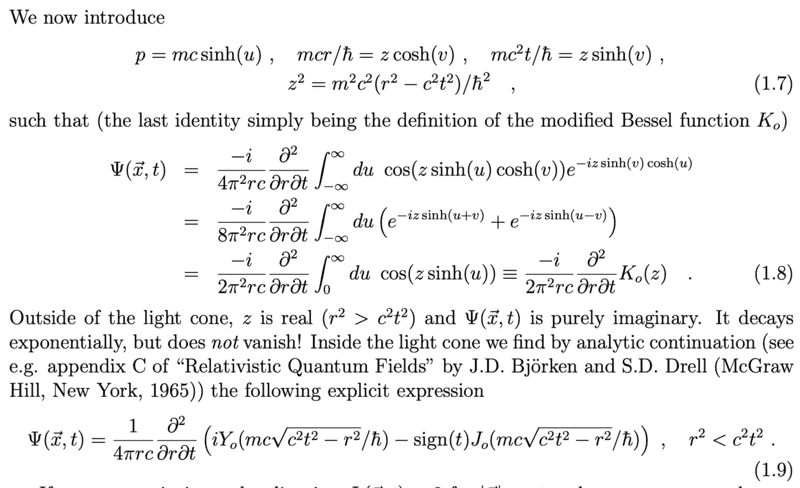
In the defined equations (1.7), the variable "v" can become imaginary, so a simple change of variables would change the integration sign by adding an imaginary term - not what occurs in (1.8). Any idea on how to get the third line of (1.8)?
Thanks for any insight!