- #1
Math Amateur
Gold Member
MHB
- 3,990
- 48
I am reading Joseph J. Rotman's book: Advanced Modern Algebra (AMA) and I am currently focused on Section 5.1 Prime Ideals and Maximal Ideals ...
I need some help with understanding the proof of Proposition 5.9 ... ...Proposition 5.9 reads as follows:
 In the proof of Proposition 5.9, Rotman writes:
In the proof of Proposition 5.9, Rotman writes:
" ... ... The Correspondence Theorem for Rings shows that ##I## is a maximal ideal if and only if ##R/I## has no ideals other than ##(0)## and ##R/I## itself ... ... "
My question is: how exactly (in clear and simple terms) does Rotman's statement of the Correspondence Theorem for Rings lead to the statement that "##I## is a maximal ideal if and only if ##R/I## has no ideals other than ##(0)## and ##R/I## itself" ... ...
Hope that someone can help ...
Peter
============================================================
The above post refers to Rotman's statement of the Correspondence Theorem for Rings, so I am providing a statement of that theorem and its proof, as follows:
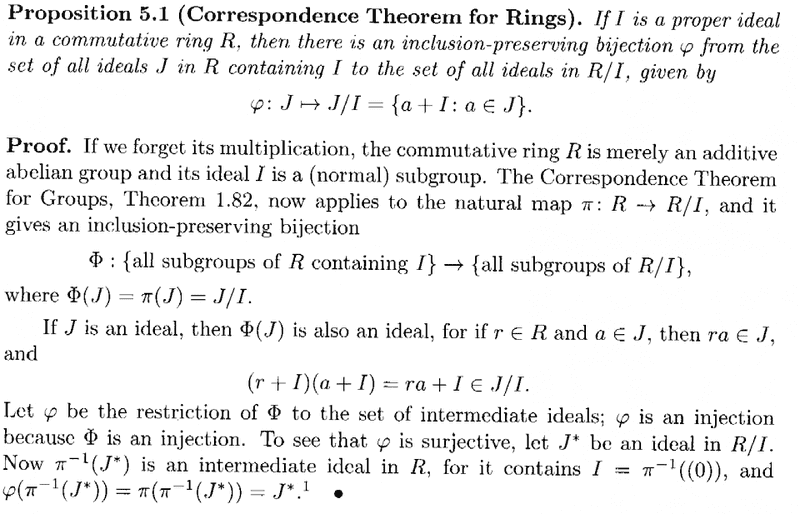
I need some help with understanding the proof of Proposition 5.9 ... ...Proposition 5.9 reads as follows:
" ... ... The Correspondence Theorem for Rings shows that ##I## is a maximal ideal if and only if ##R/I## has no ideals other than ##(0)## and ##R/I## itself ... ... "
My question is: how exactly (in clear and simple terms) does Rotman's statement of the Correspondence Theorem for Rings lead to the statement that "##I## is a maximal ideal if and only if ##R/I## has no ideals other than ##(0)## and ##R/I## itself" ... ...
Hope that someone can help ...
Peter
============================================================
The above post refers to Rotman's statement of the Correspondence Theorem for Rings, so I am providing a statement of that theorem and its proof, as follows:

