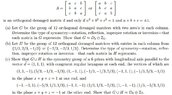The Symmetry Group of a Hexagonal Prism and Orthogonal Matrices

[JonJones]

View attachment 6246

For part (a) we have 6 rotations, 3 reflections, 1 inversion, and 2 improper rotations, determined by the determinant and trace of the given matrix. We can take K to be the group of 3 rotations and 3 reflections, which is a Normal subgroup since it has index 2. We can take J to be the group $\{I,-I\}$, which is also Normal. So we can see that $K\approx D_3$, $J\approx \mathbb{Z}_2$, $K\cap J=\{I\}$ and $G=KJ$. Which by definition means that $G=K\times J\approx K\oplus J \approx D_3\oplus \mathbb{Z}_2$. Is this correct?

Part (b) seems very similar to part (a) but it's just slightly harder to find the determinant and trace.

I'm stuck on part (c). Not sure how to proceed. I think by "6-prism" they mean a hexagonal prism.

 

[jvicens]

Hello there,

You are correct in your analysis for part (a). The given matrix has 6 rotations (corresponding to the 6 vertices of a regular hexagon), 3 reflections (corresponding to the 3 axes passing through opposite vertices), 1 inversion (corresponding to the center of the hexagon), and 2 improper rotations (corresponding to the 2 diagonals of the hexagon). This yields a total of 12 symmetries, which form the dihedral group $D_6$.

For part (b), we can use the same approach but with a slightly different matrix. We can take the matrix to be:

$$
A=\begin{pmatrix}
-1 & 0 & 0 \\
0 & 1 & 0 \\
0 & 0 & -1
\end{pmatrix}
$$

This matrix has determinant -1 and trace -3, which correspond to the 3 rotations and 3 reflections of a cube. This yields the group $K\approx D_3$ as before, and we can take $J$ to be the group $\{I,-I\}$ as before. This yields the group $G\approx D_3\times \mathbb{Z}_2 \approx D_3\oplus \mathbb{Z}_2$.

For part (c), we can use a similar approach but with a different matrix. We can take the matrix to be:

$$
B=\begin{pmatrix}
0 & 1 & 0 \\
1 & 0 & 0 \\
0 & 0 & 1
\end{pmatrix}
$$

This matrix has determinant 1 and trace 1, which correspond to the 6 rotations and 1 inversion of a hexagonal prism. We can take $K$ to be the group of rotations, which is isomorphic to the cyclic group of order 6, and $J$ to be the group of reflections, which is isomorphic to the cyclic group of order 2. This yields the group $G\approx \mathbb{Z}_6\times \mathbb{Z}_2 \approx \mathbb{Z}_6\oplus \mathbb{Z}_2$, which is isomorphic to the group of symmetries of a hexagonal prism.

I hope this helps! Let me know if you have any further questions.